著名医師による解説が無料で読めます
すると翻訳の精度が向上します
化学電位勾配上の電解溶液中のイオンのドリフトを予測することを目的とした数値モデルの主な特徴が示されています。イオン拡散のメカニズムは、拡張された方程式の拡張されたナーンストプランクシステムを解くことによって説明されます。さまざまなイオンフラックス間の電気結合は、ポアソン方程式によって説明されます。さらに、化学活性の影響はモデルで考慮されます。非線形方程式のシステム全体は、有限要素法を使用して解決されます。単純なテストケースのモデルによって得られた結果は、分析ソリューションを使用して得られたものと比較されます。より複雑な問題へのモデルのアプリケーションも提示され、議論されます。Copyright 1999 Academic Press。
化学電位勾配上の電解溶液中のイオンのドリフトを予測することを目的とした数値モデルの主な特徴が示されています。イオン拡散のメカニズムは、拡張された方程式の拡張されたナーンストプランクシステムを解くことによって説明されます。さまざまなイオンフラックス間の電気結合は、ポアソン方程式によって説明されます。さらに、化学活性の影響はモデルで考慮されます。非線形方程式のシステム全体は、有限要素法を使用して解決されます。単純なテストケースのモデルによって得られた結果は、分析ソリューションを使用して得られたものと比較されます。より複雑な問題へのモデルのアプリケーションも提示され、議論されます。Copyright 1999 Academic Press。
The main features of a numerical model aiming at predicting the drift of ions in an electrolytic solution upon a chemical potential gradient are presented. The mechanisms of ionic diffusion are described by solving the extended Nernst-Planck system of equations. The electrical coupling between the various ionic fluxes is accounted for by the Poisson equation. Furthermore, chemical activity effects are considered in the model. The whole system of nonlinear equations is solved using the finite-element method. Results yielded by the model for simple test cases are compared to those obtained using an analytical solution. Applications of the model to more complex problems are also presented and discussed. Copyright 1999 Academic Press.
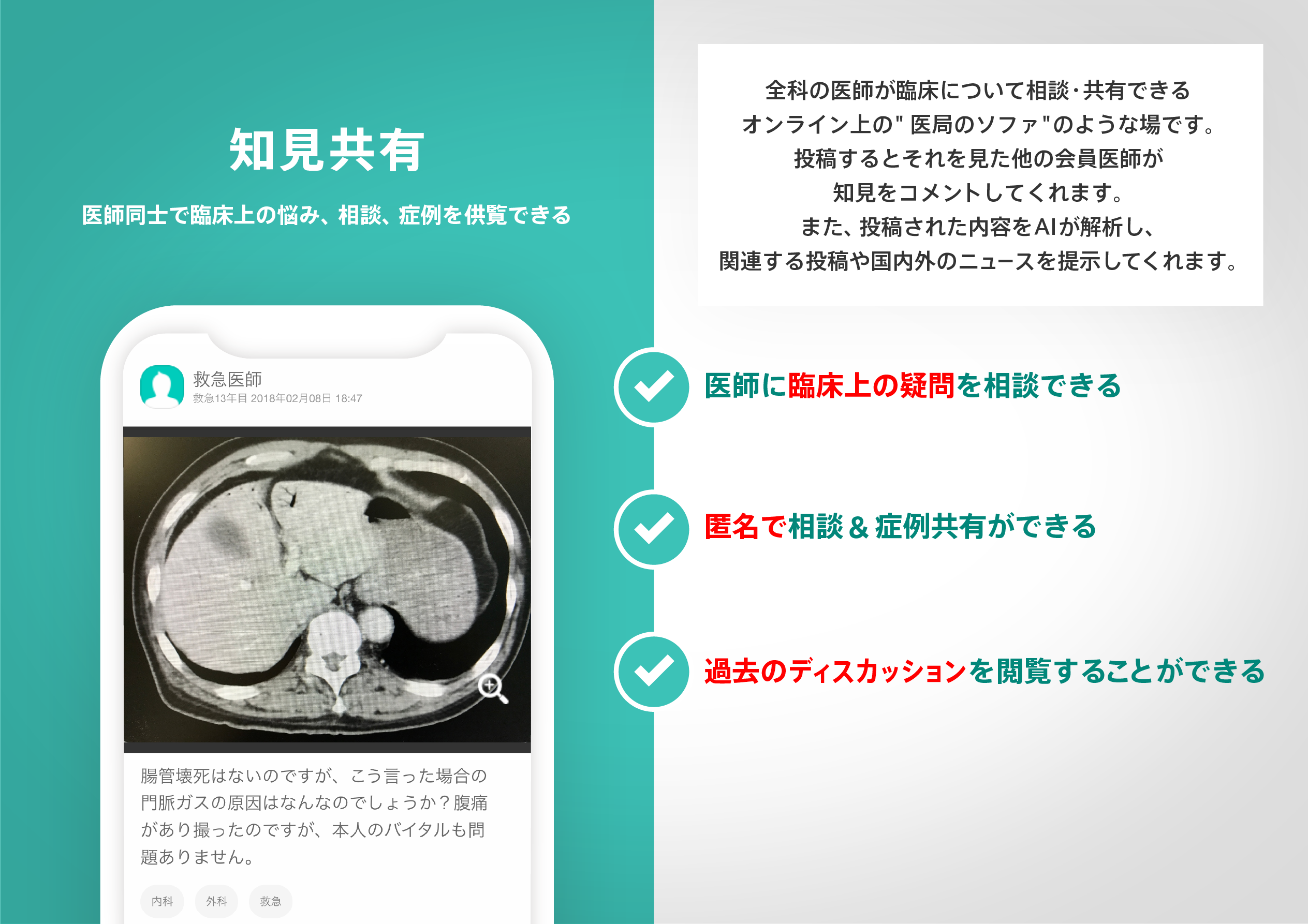
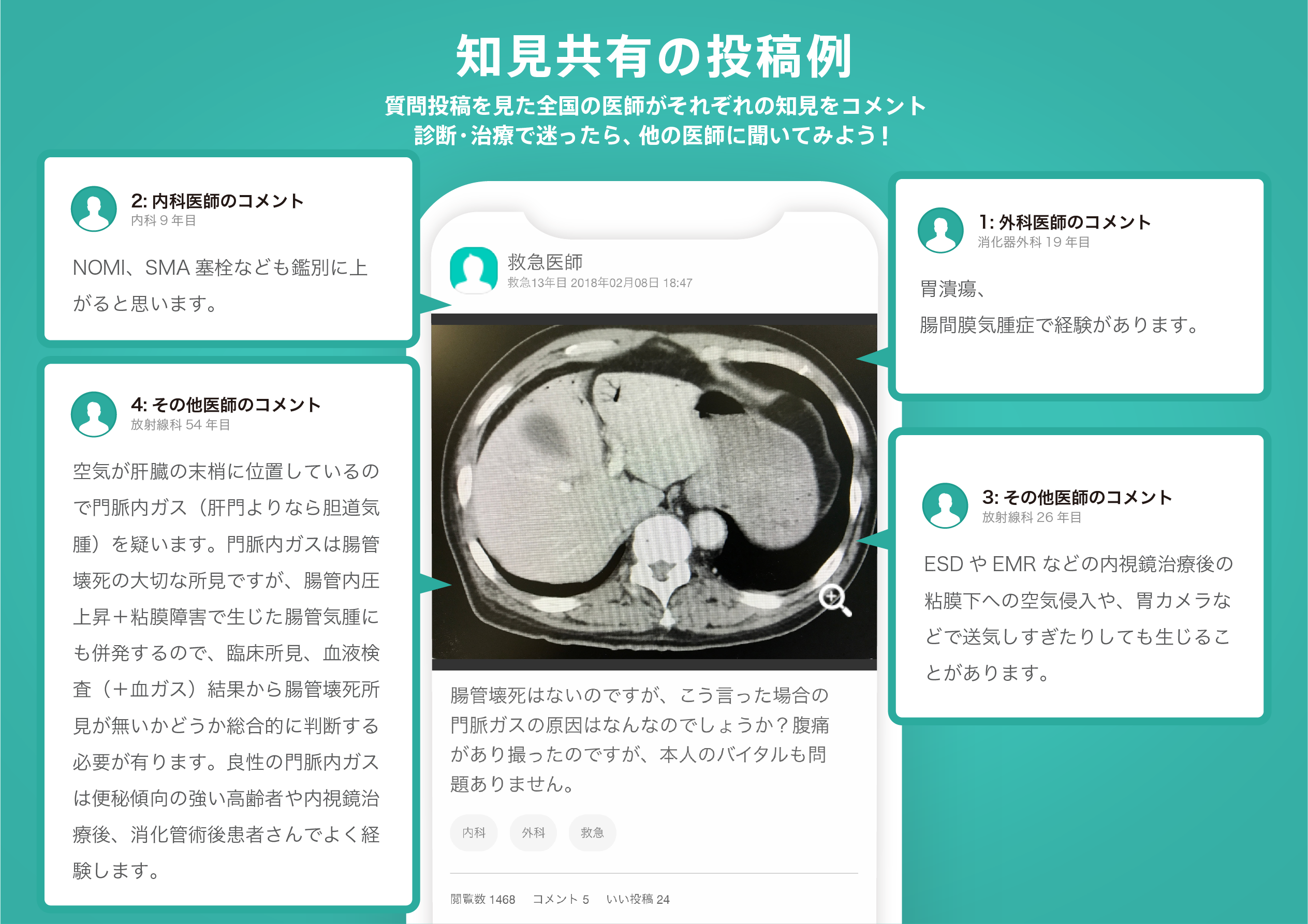
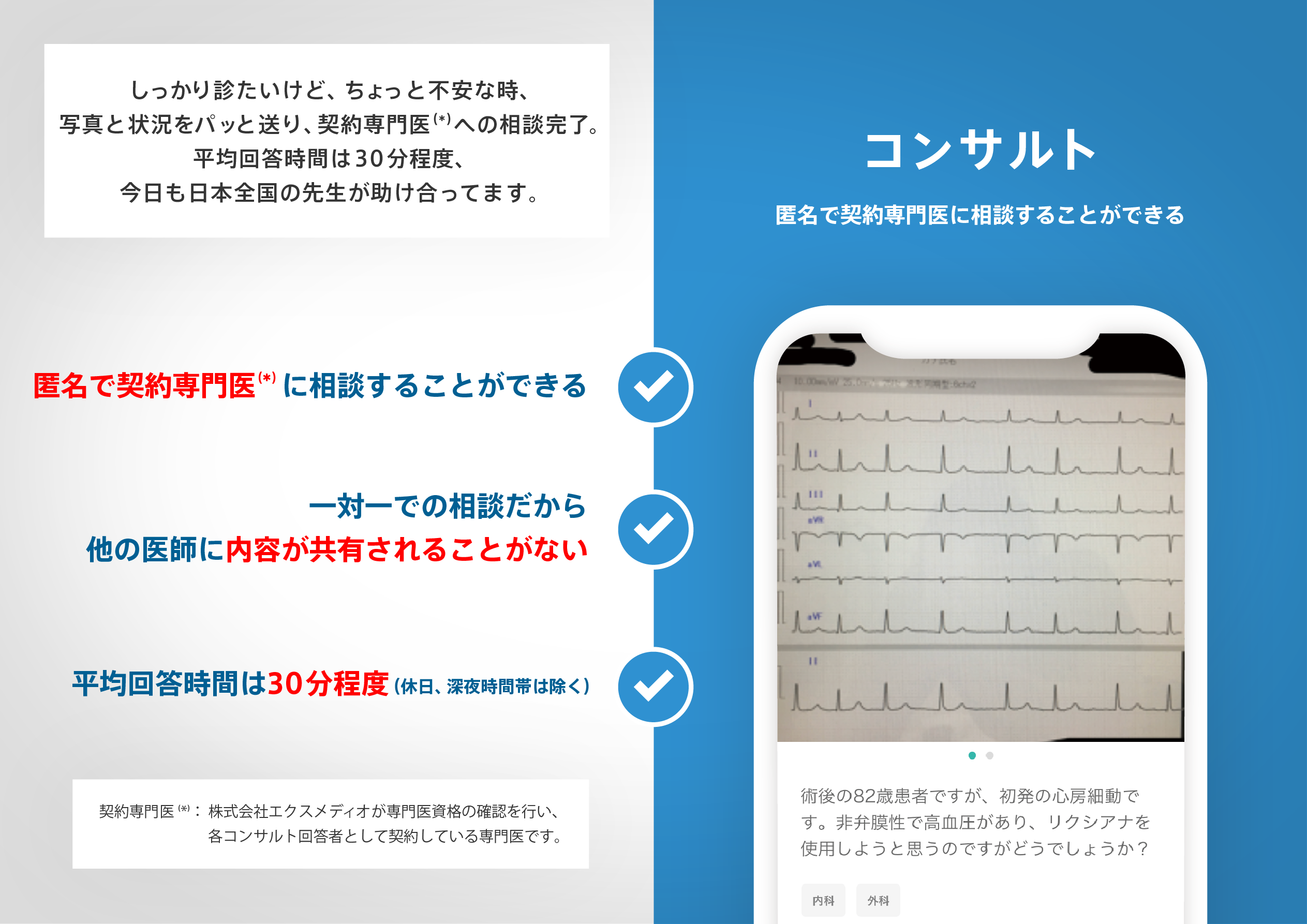
医師のための臨床サポートサービス
ヒポクラ x マイナビのご紹介
無料会員登録していただくと、さらに便利で効率的な検索が可能になります。