著名医師による解説が無料で読めます
すると翻訳の精度が向上します
私たちは、均質なボーズ・エインシュタイン凝縮液(BEC)の詳細な平衡外の時間の進化を研究します。BECの説明のための効果的な微視的モデルと、熱浴に浸された自己取り合わせる複雑なスカラーフィールドの非相性学的量子理論を考慮します。変動間の相互作用は、不安定な生成のメカニズムにおいて重要であることが証明されています。K空間に2つのレジームの存在を示し、K(2)/2Mのクロスオーバーがあり、約2ラムダ| PHI(0)|(2)があります。ここで、ラムダはカップリング定数と| phi(0)|(2)です。凝縮液密度です。凝縮液密度の非平衡ダイナミクスを完全に決定する結合方程式のセットを推定して解決します。
私たちは、均質なボーズ・エインシュタイン凝縮液(BEC)の詳細な平衡外の時間の進化を研究します。BECの説明のための効果的な微視的モデルと、熱浴に浸された自己取り合わせる複雑なスカラーフィールドの非相性学的量子理論を考慮します。変動間の相互作用は、不安定な生成のメカニズムにおいて重要であることが証明されています。K空間に2つのレジームの存在を示し、K(2)/2Mのクロスオーバーがあり、約2ラムダ| PHI(0)|(2)があります。ここで、ラムダはカップリング定数と| phi(0)|(2)です。凝縮液密度です。凝縮液密度の非平衡ダイナミクスを完全に決定する結合方程式のセットを推定して解決します。
We study the detailed out-of-equilibrium time evolution of a homogeneous Bose-Einstein condensate (BEC). We consider a nonrelativistic quantum theory for a self-interacting complex scalar field, immersed in a thermal bath, as an effective microscopic model for the description of the BEC. The interaction between fluctuations proves to be crucial in the mechanism of instability generation. We show the existence of two regimes in k space, with a crossover for k(2)/2m approximately 2 lambda|phi(0)|(2), where lambda is the coupling constant and |phi(0)|(2) is the condensate density. We deduce and solve a set of coupled equations that completely determines the nonequilibrium dynamics of the condensate density.
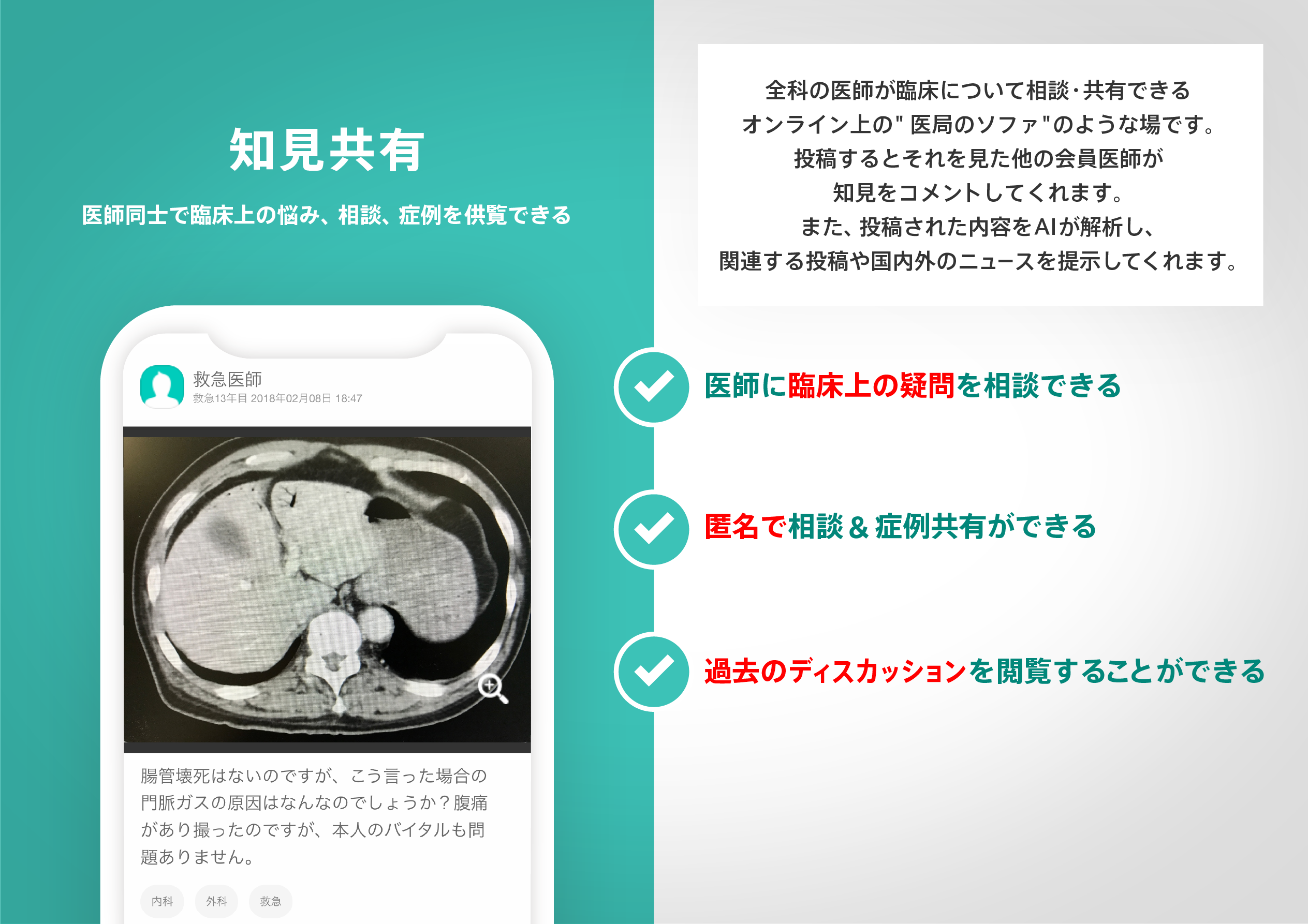
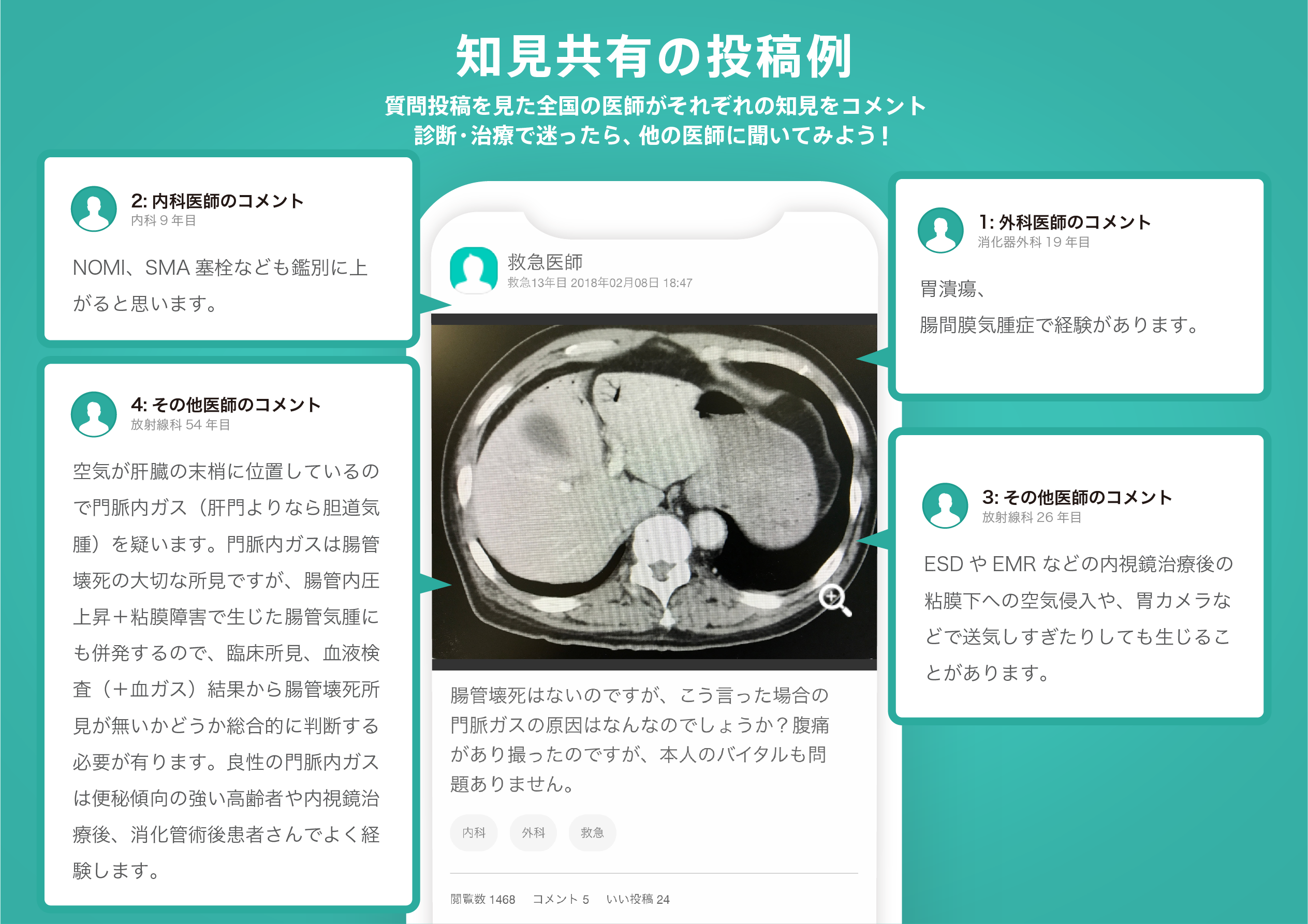
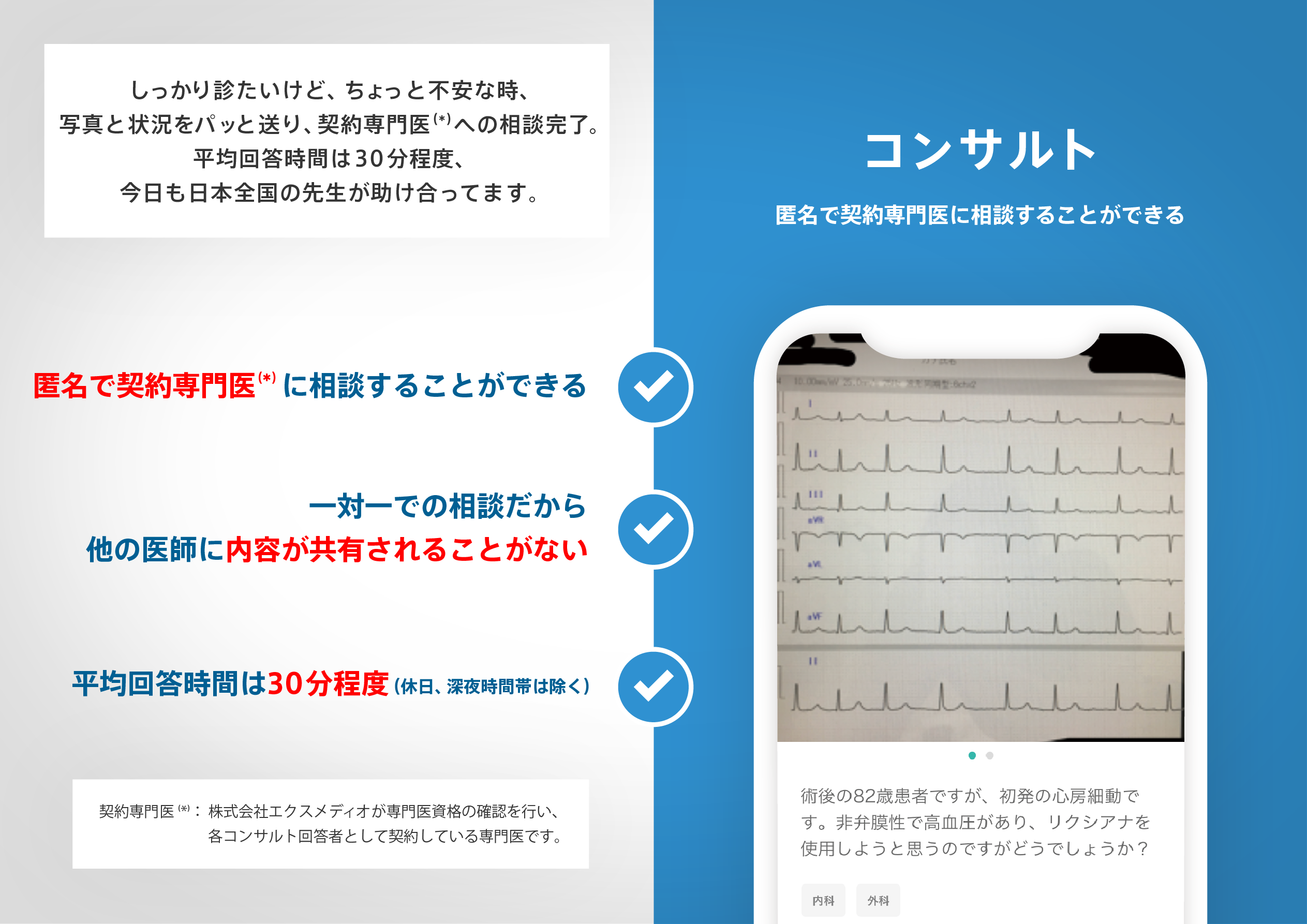
医師のための臨床サポートサービス
ヒポクラ x マイナビのご紹介
無料会員登録していただくと、さらに便利で効率的な検索が可能になります。