著名医師による解説が無料で読めます
すると翻訳の精度が向上します
統計結果のグラフィカルな表現は、多くの場合、調査結果の解釈を読者に支援するために使用されます。これは、特定の共変量の経時的な生存のパターンを説明することに関心がある生存分析に特に当てはまります。グループメンバーシップインジケーターなどの固定されたカテゴリの共変量の場合、Kaplan-Meier推定(1958)を使用して曲線を表示できます。時間依存の共変量の場合、この方法は適切ではないかもしれません。Simon and Makuch(1984)は、各イベントでリスクを抱えている個人の共変量状態を評価する手法を提案しました。この方法では、時間の経過とともに個人の共変量状態の変化を考慮しています。生存計算は、条件付き生存の推定値が、各イベントでのリスクのあるイベントの数の比率の関数であるという点で、Kaplan-Meierメソッドと同じです。2つの方法の違いは、共変量によって定義された各レベル内のリスクのある個人は、カプラン・マイヤー法のようにサイモンとマクッチ法の時間0で固定されていないことです。COX比例ハザード回帰分析における時間依存の共変量に対して、2つの方法がどのように異なるかの例が提示されます。
統計結果のグラフィカルな表現は、多くの場合、調査結果の解釈を読者に支援するために使用されます。これは、特定の共変量の経時的な生存のパターンを説明することに関心がある生存分析に特に当てはまります。グループメンバーシップインジケーターなどの固定されたカテゴリの共変量の場合、Kaplan-Meier推定(1958)を使用して曲線を表示できます。時間依存の共変量の場合、この方法は適切ではないかもしれません。Simon and Makuch(1984)は、各イベントでリスクを抱えている個人の共変量状態を評価する手法を提案しました。この方法では、時間の経過とともに個人の共変量状態の変化を考慮しています。生存計算は、条件付き生存の推定値が、各イベントでのリスクのあるイベントの数の比率の関数であるという点で、Kaplan-Meierメソッドと同じです。2つの方法の違いは、共変量によって定義された各レベル内のリスクのある個人は、カプラン・マイヤー法のようにサイモンとマクッチ法の時間0で固定されていないことです。COX比例ハザード回帰分析における時間依存の共変量に対して、2つの方法がどのように異なるかの例が提示されます。
Graphical representation of statistical results is often used to assist readers in the interpretation of the findings. This is especially true for survival analysis where there is an interest in explaining the patterns of survival over time for specific covariates. For fixed categorical covariates, such as a group membership indicator, Kaplan-Meier estimates (1958) can be used to display the curves. For time-dependent covariates this method may not be adequate. Simon and Makuch (1984) proposed a technique that evaluates the covariate status of the individuals remaining at risk at each event time. The method takes into account the change in an individual's covariate status over time. The survival computations are the same as the Kaplan-Meier method, in that the conditional survival estimates are the function of the ratio of the number of events to the number at risk at each event time. The difference between the two methods is that the individuals at risk within each level defined by the covariate is not fixed at time 0 in the Simon and Makuch method as it is with the Kaplan-Meier method. Examples of how the two methods can differ for time dependent covariates in Cox proportional hazards regression analysis are presented.
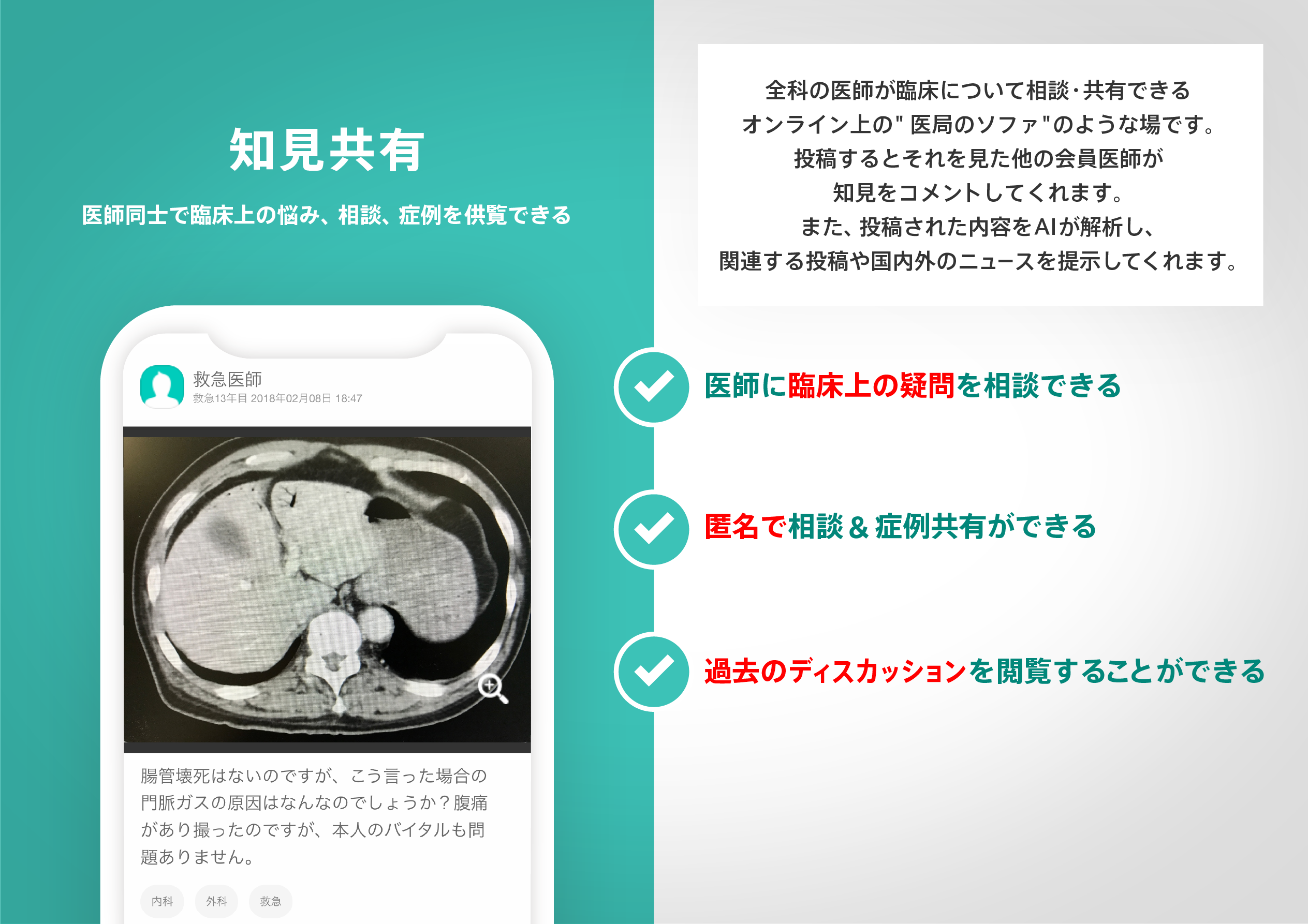
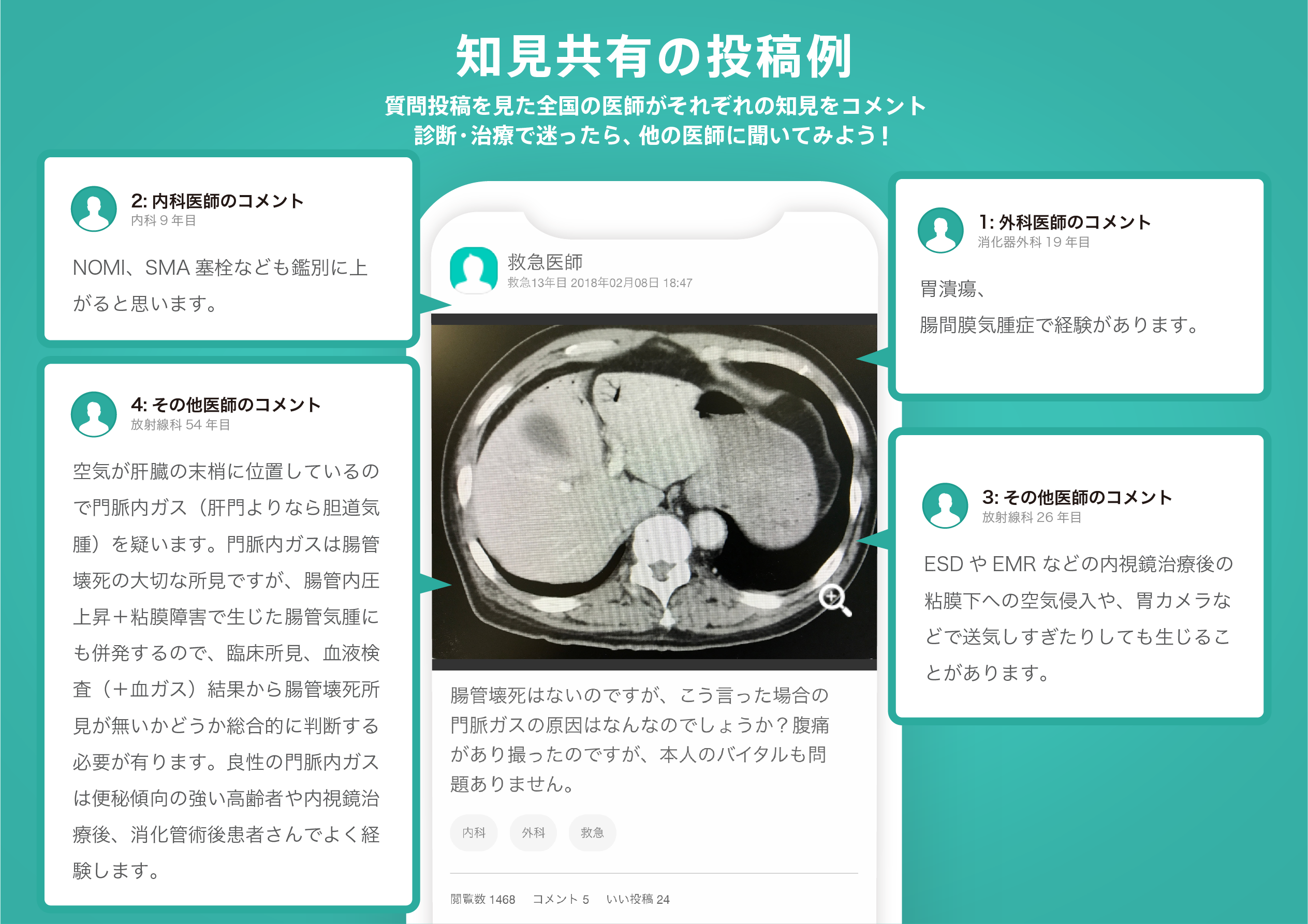
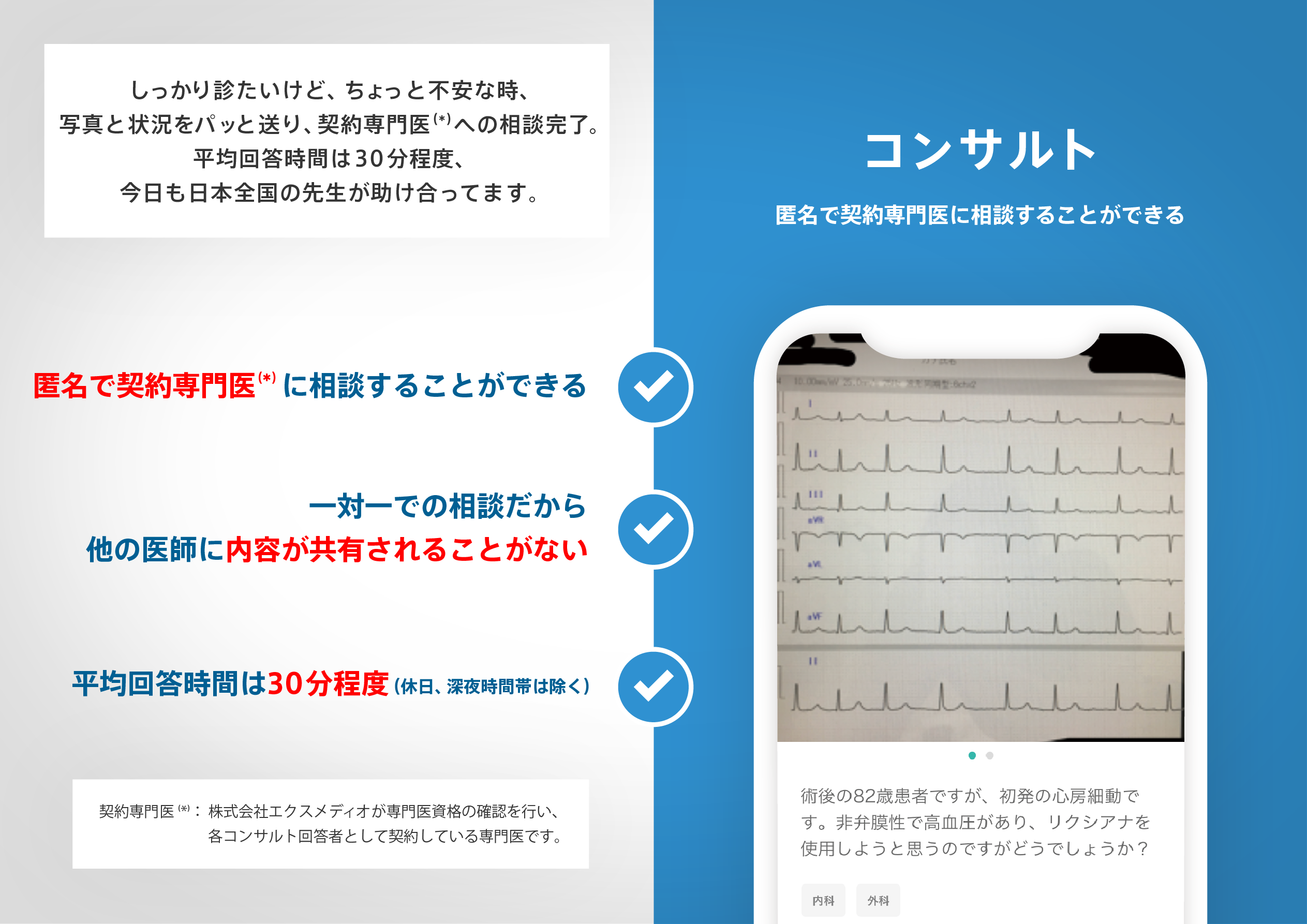
医師のための臨床サポートサービス
ヒポクラ x マイナビのご紹介
無料会員登録していただくと、さらに便利で効率的な検索が可能になります。