著名医師による解説が無料で読めます
すると翻訳の精度が向上します
逆音響散乱問題の最も堅牢な治療法は、リップマン・シュウィンガー方程式の生まれたネウマンシリーズ溶液の復帰に基づいています。この反転へのこのアプローチの重要な問題は、フレドホルム積分カーネルの生まれたネウマンシリーズの収束半径、特に相互作用が周波数の平方に依存する音響散乱の半径です。対照的に、量子散乱におけるVolterra積分方程式の生まれたネウマンシリーズは、相互作用を特徴付けるカップリングの強度とは無関係に、絶対に収束していることがよく知られています。リップマン・シュウィンガー方程式のフレッドホルムから繰り込みによるヴォルテラ構造への変換は、量子散乱計算と電磁散乱について以前に考慮されています。このホワイトペーパーでは、繰り返しの技術を採用して、逆音響散乱シリーズのVolterra方程式フレームワークを取得し、このシリーズが定数値と周波数値を結合する複雑な平面全体に完全に収束していることを証明しています。現在の結果は、1つの次元の音響散乱に関するものですが、この方法は一般的です。このアプローチは、音響散乱のための2つの単純な1次元モデルへのアプリケーションによって説明されています。
逆音響散乱問題の最も堅牢な治療法は、リップマン・シュウィンガー方程式の生まれたネウマンシリーズ溶液の復帰に基づいています。この反転へのこのアプローチの重要な問題は、フレドホルム積分カーネルの生まれたネウマンシリーズの収束半径、特に相互作用が周波数の平方に依存する音響散乱の半径です。対照的に、量子散乱におけるVolterra積分方程式の生まれたネウマンシリーズは、相互作用を特徴付けるカップリングの強度とは無関係に、絶対に収束していることがよく知られています。リップマン・シュウィンガー方程式のフレッドホルムから繰り込みによるヴォルテラ構造への変換は、量子散乱計算と電磁散乱について以前に考慮されています。このホワイトペーパーでは、繰り返しの技術を採用して、逆音響散乱シリーズのVolterra方程式フレームワークを取得し、このシリーズが定数値と周波数値を結合する複雑な平面全体に完全に収束していることを証明しています。現在の結果は、1つの次元の音響散乱に関するものですが、この方法は一般的です。このアプローチは、音響散乱のための2つの単純な1次元モデルへのアプリケーションによって説明されています。
The most robust treatment of the inverse acoustic scattering problem is based on the reversion of the Born-Neumann series solution of the Lippmann-Schwinger equation. An important issue for this approach to inversion is the radius of convergence of the Born-Neumann series for Fredholm integral kernels, and especially for acoustic scattering for which the interaction depends on the square of the frequency. By contrast, it is well known that the Born-Neumann series for the Volterra integral equations in quantum scattering are absolutely convergent, independent of the strength of the coupling characterizing the interaction. The transformation of the Lippmann-Schwinger equation from a Fredholm to a Volterra structure by renormalization has been considered previously for quantum scattering calculations and electromagnetic scattering. In this paper, we employ the renormalization technique to obtain a Volterra equation framework for the inverse acoustic scattering series, proving that this series also converges absolutely in the entire complex plane of coupling constant and frequency values. The present results are for acoustic scattering in one dimension, but the method is general. The approach is illustrated by applications to two simple one-dimensional models for acoustic scattering.
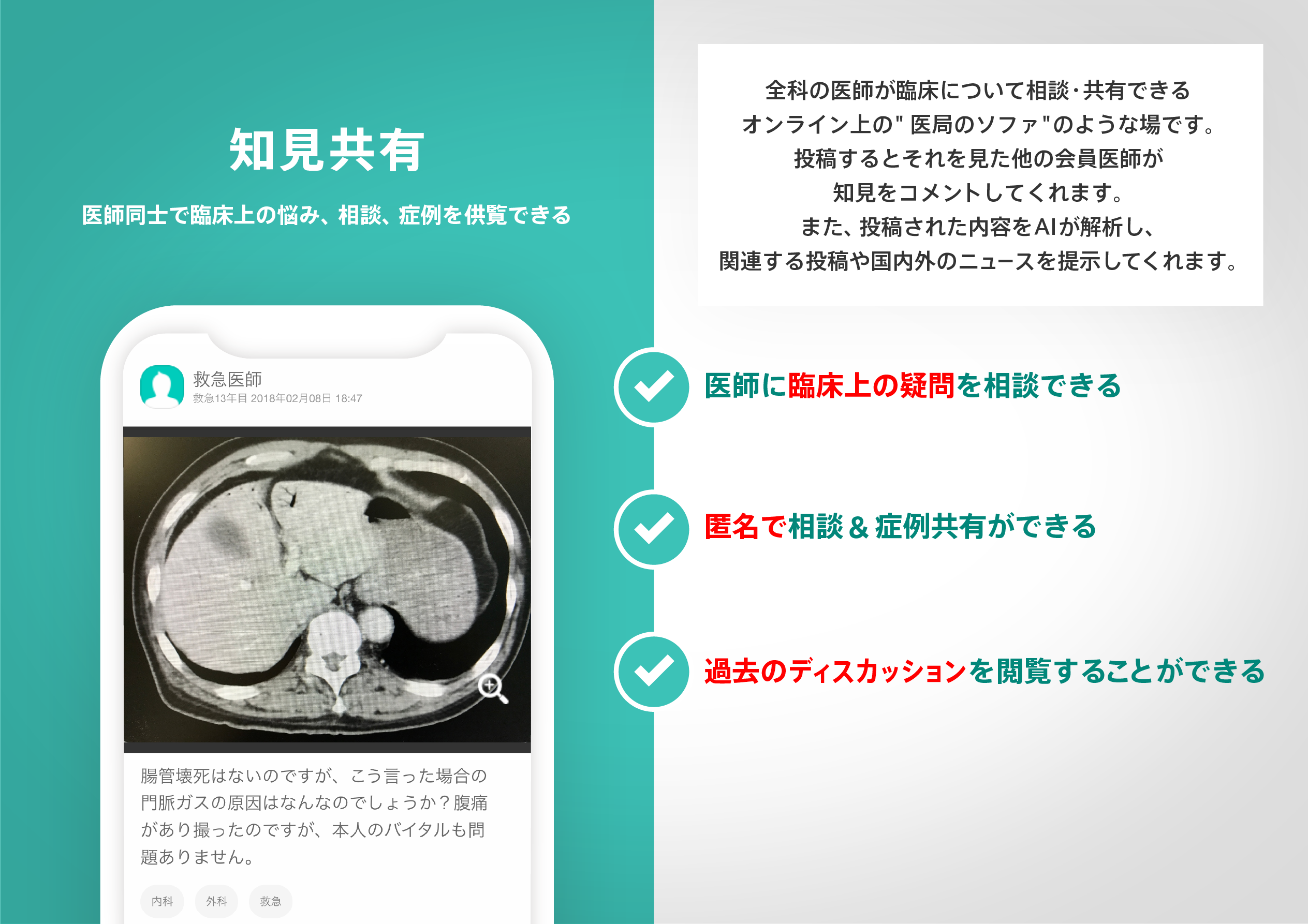
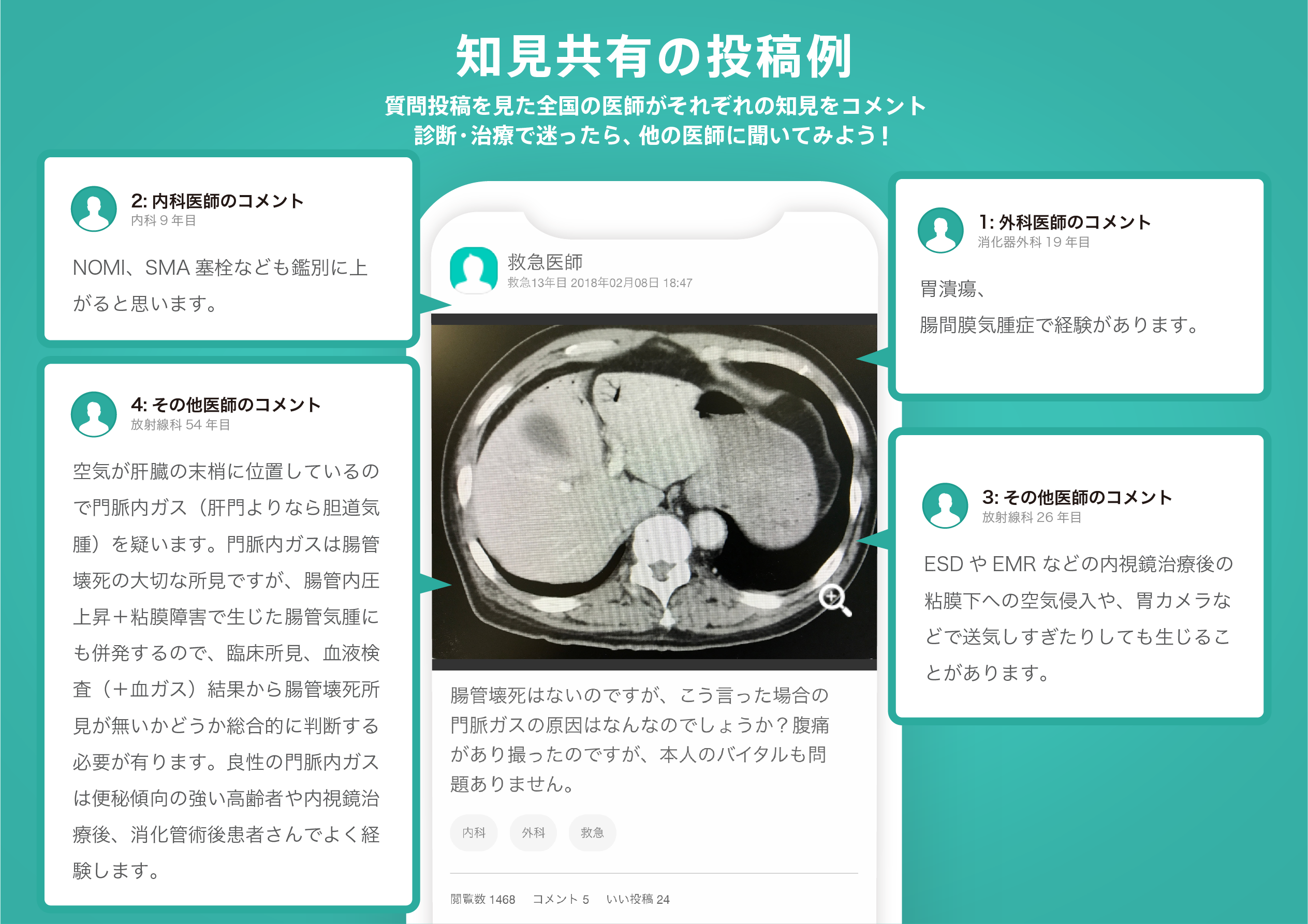
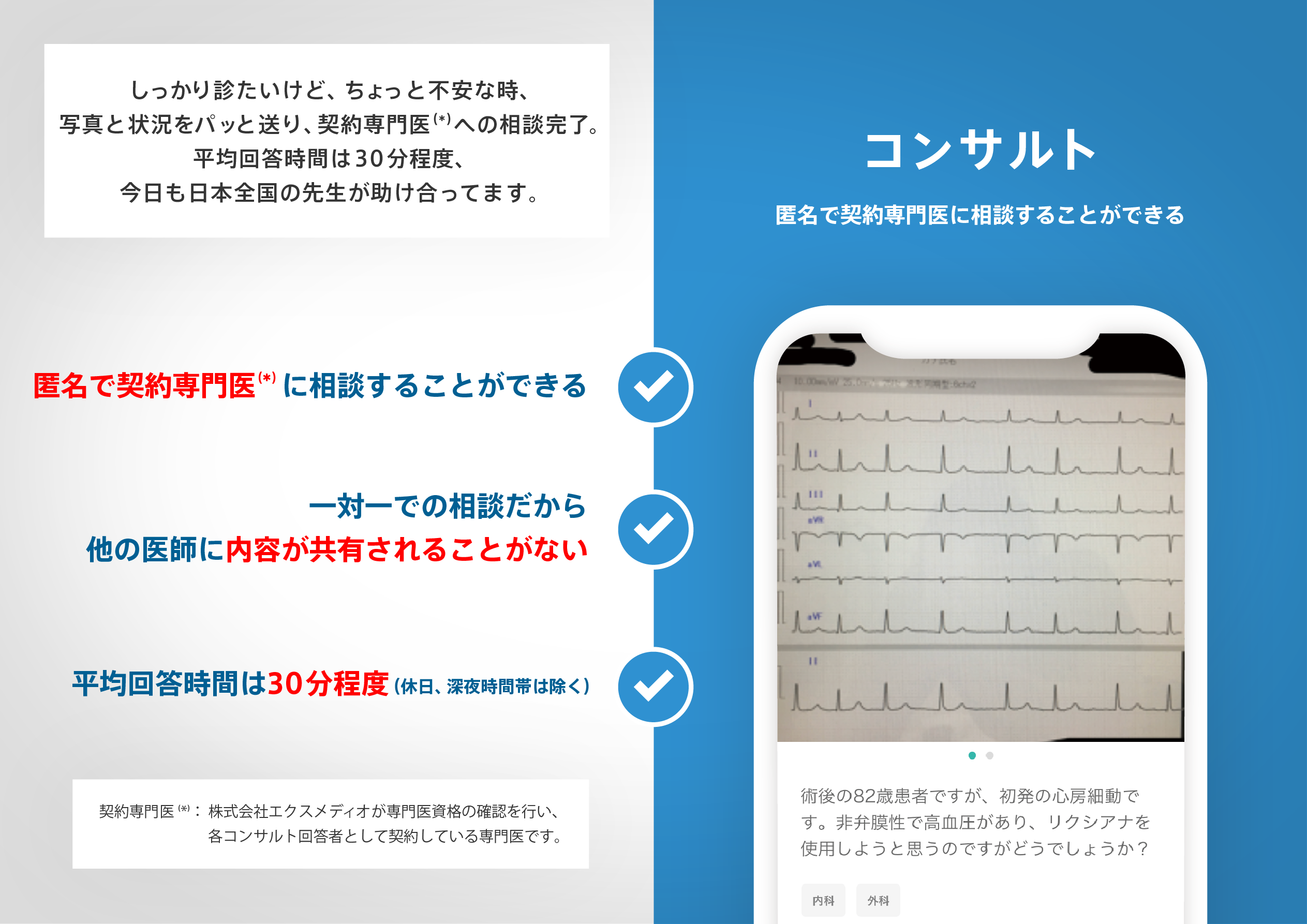
医師のための臨床サポートサービス
ヒポクラ x マイナビのご紹介
無料会員登録していただくと、さらに便利で効率的な検索が可能になります。