著名医師による解説が無料で読めます
すると翻訳の精度が向上します
(2+1)次元のKardar-Parisi-Zhang(KPZ)クラスの結晶固体のエッチングと他の離散モデルの結果のために提案されたモデルのシミュレーション結果を分析します。定常状態では、高さ分布の順序n = 2,3,4のモーメントw(n)が推定されます。エッチングモデル、弾道堆積モデル、および温度依存性体中心の制限された固体ソリッドソリッドソリッドソリッドソリッドモデルの結果は、W(3)/W(3/2)と同一の歪度Sの絶対値の普遍性を示唆しています。(2)およびw(4)/w(2)(2)-3と同一の尖度qの値。歪度の兆候は、連続体限界のプロセスを表すKPZ方程式のパラメーターラムダと同じです。エッチングモデルから得られる最良の数値推定値は、S = 0.26 +/- 0.01およびQ = 0.134 +/- 0.015の絶対値です。このモデルでは、粗さの指数alpha = 0.383 +/- 0.008が取得され、四角界面幅のスケーリングに一定の補正項(固有の幅)を占めます。この値は、広範なシミュレーションの以前の推定値をわずかに下回り、alpha = 2/5の正確な値の提案を排除します。結論は、弾道堆積モデルの結果によって裏付けられています。動的指数と成長指数の独立した推定値は、それぞれ1.605 <OR = Z <OR = 1.64およびBETA = 0.229 +/- 0.005であり、これはAlpha+Z = 2およびZ = Alpha/Betaとの関係と一致しています。
(2+1)次元のKardar-Parisi-Zhang(KPZ)クラスの結晶固体のエッチングと他の離散モデルの結果のために提案されたモデルのシミュレーション結果を分析します。定常状態では、高さ分布の順序n = 2,3,4のモーメントw(n)が推定されます。エッチングモデル、弾道堆積モデル、および温度依存性体中心の制限された固体ソリッドソリッドソリッドソリッドソリッドモデルの結果は、W(3)/W(3/2)と同一の歪度Sの絶対値の普遍性を示唆しています。(2)およびw(4)/w(2)(2)-3と同一の尖度qの値。歪度の兆候は、連続体限界のプロセスを表すKPZ方程式のパラメーターラムダと同じです。エッチングモデルから得られる最良の数値推定値は、S = 0.26 +/- 0.01およびQ = 0.134 +/- 0.015の絶対値です。このモデルでは、粗さの指数alpha = 0.383 +/- 0.008が取得され、四角界面幅のスケーリングに一定の補正項(固有の幅)を占めます。この値は、広範なシミュレーションの以前の推定値をわずかに下回り、alpha = 2/5の正確な値の提案を排除します。結論は、弾道堆積モデルの結果によって裏付けられています。動的指数と成長指数の独立した推定値は、それぞれ1.605 <OR = Z <OR = 1.64およびBETA = 0.229 +/- 0.005であり、これはAlpha+Z = 2およびZ = Alpha/Betaとの関係と一致しています。
We analyze simulation results of a model proposed for etching of a crystalline solid and results of other discrete models in the (2+1)-dimensional Kardar-Parisi-Zhang (KPZ) class. In the steady states, the moments W(n) of orders n=2,3,4 of the height distribution are estimated. Results for the etching model, the ballistic deposition model, and the temperature-dependent body-centered restricted solid-on-solid model suggest the universality of the absolute value of the skewness S identical with W(3)/W(3/2)(2) and of the value of the kurtosis Q identical with W(4)/W(2)(2)-3. The sign of the skewness is the same as of the parameter lambda of the KPZ equation which represents the process in the continuum limit. The best numerical estimates, obtained from the etching model, are absolute value of S=0.26+/-0.01 and Q=0.134+/-0.015. For this model, the roughness exponent alpha=0.383+/-0.008 is obtained, accounting for a constant correction term (intrinsic width) in the scaling of the squared interface width. This value is slightly below previous estimates of extensive simulations and rules out the proposal of the exact value alpha=2/5. The conclusion is supported by results for the ballistic deposition model. Independent estimates of the dynamical exponent and of the growth exponent are 1.605< or =z< or =1.64 and beta=0.229+/-0.005, respectively, which are consistent with the relations alpha+z=2 and z=alpha/beta.
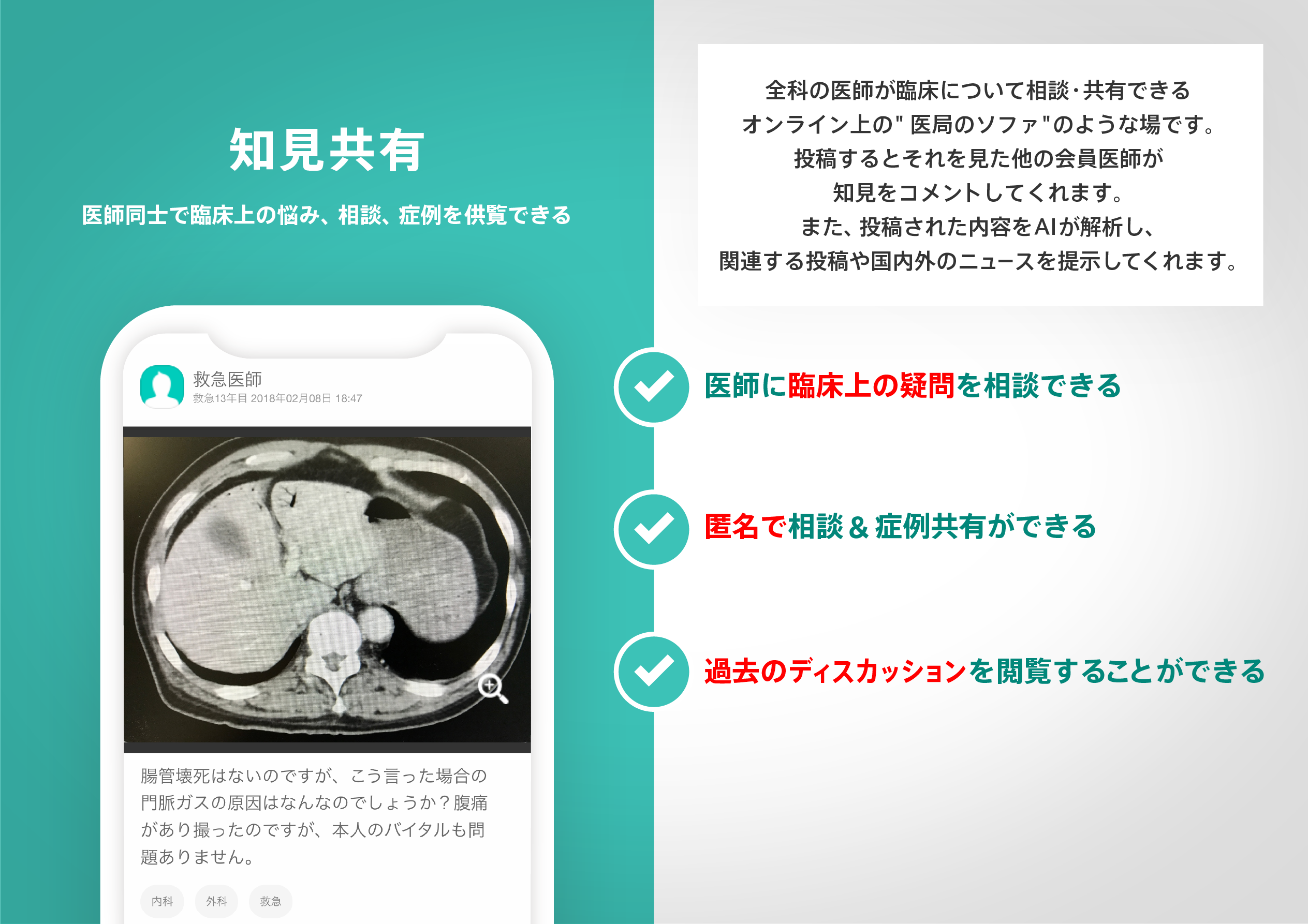
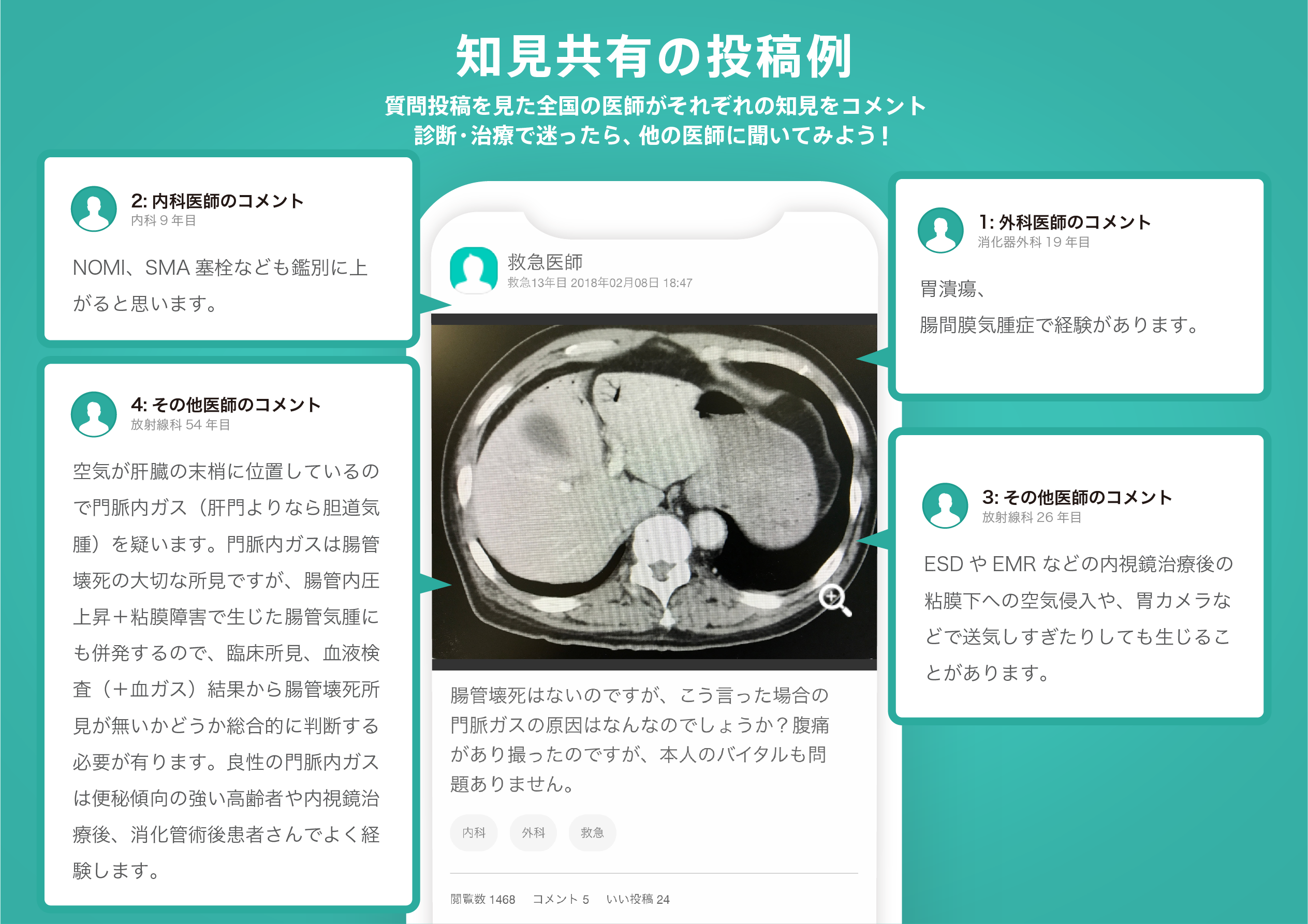
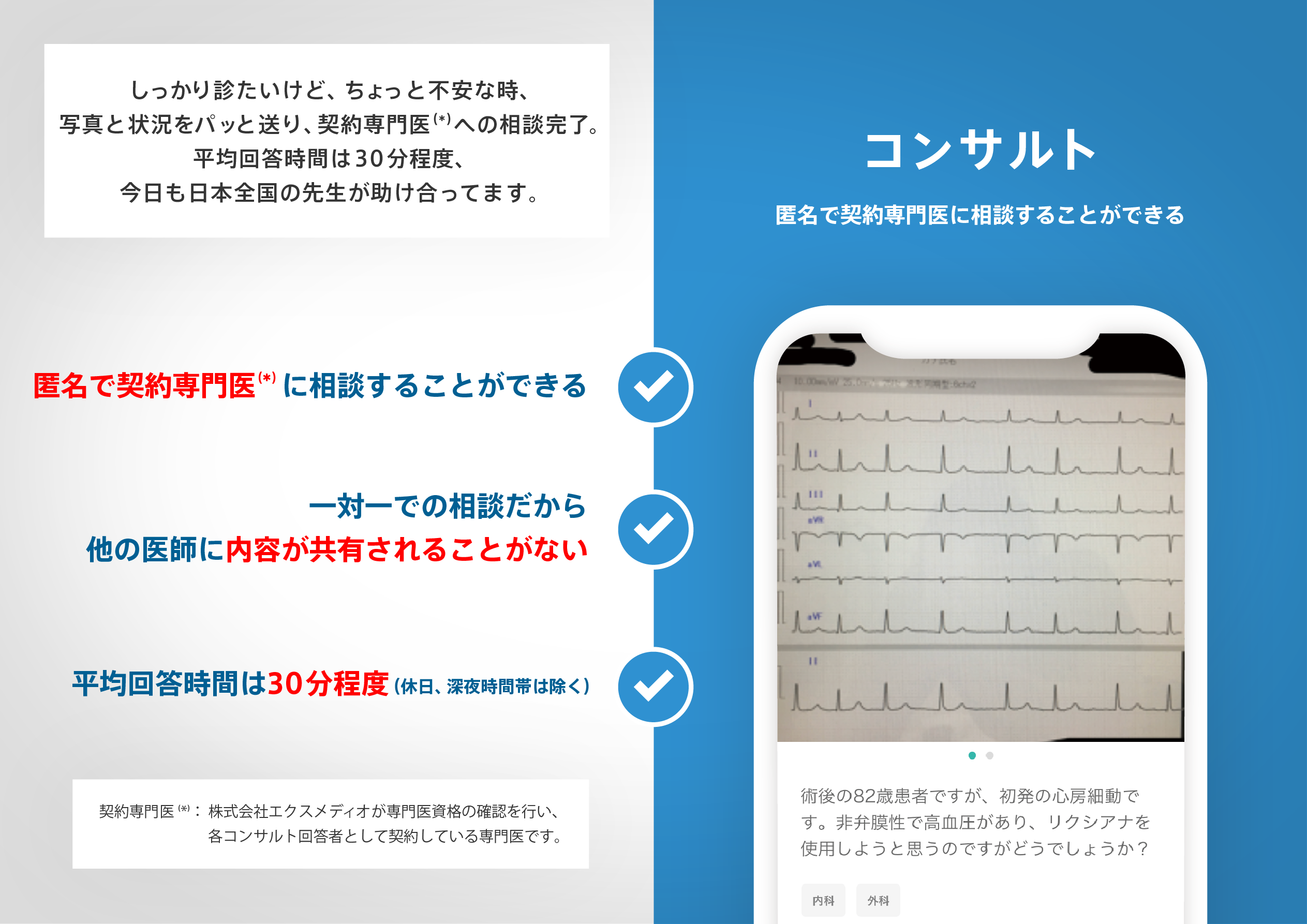
医師のための臨床サポートサービス
ヒポクラ x マイナビのご紹介
無料会員登録していただくと、さらに便利で効率的な検索が可能になります。