著名医師による解説が無料で読めます
すると翻訳の精度が向上します
従来、身体の分布のダイナミクスは、いわゆる分布半減期(T1/2、アルファなど)によって評価されます。しかし、その後、分布プロセスの平均時間は、除去の影響により過小評価されています。対照的に、薬物処分曲線に含まれる分布のダイナミクスに関する情報は、滞留時間分布の分散(VDRT)および歪度(SDRT)に関連するパラメーター、2番目と3番目の曲線モーメントによって抽出できます。一方、分布の量(VSS)を特徴とする平衡状態は、平均滞留時間(MDRT)または最初の曲線モーメントによって決定されます。このアプローチは、詳細な構造モデルまたは特定の気質関数に依存しない一般的な非補数分析を表します。薬物分布のダイナミクスを特徴付けるために2つのパラメーターが導入されています。(i)瞬間分布平衡(VDRTに関連する)の「よく混合された」挙動からの出発の程度(ii)平衡が達成されるまでの平均時間(平衡平衡時間、MEQT)。両方のパラメーターは、分布のダイナミクスの定量的尺度であり、対応する非除去システム内の分布の観点から明示的な物理的意義を表示します。さらに、双様体の性質曲線のいわゆる「分布段階」は、対応する非誘発システムとハーフタイムの混合、T1/2、M = T1/2、アルファ(Vベータ/vss*)とのミキシングとの単一補助混合曲線に関連していることが示されています。結果は、それぞれ肝臓に並んだラットと無傷のラットのアセトアミノフェンについて測定された混合および処分曲線に適用されます。
従来、身体の分布のダイナミクスは、いわゆる分布半減期(T1/2、アルファなど)によって評価されます。しかし、その後、分布プロセスの平均時間は、除去の影響により過小評価されています。対照的に、薬物処分曲線に含まれる分布のダイナミクスに関する情報は、滞留時間分布の分散(VDRT)および歪度(SDRT)に関連するパラメーター、2番目と3番目の曲線モーメントによって抽出できます。一方、分布の量(VSS)を特徴とする平衡状態は、平均滞留時間(MDRT)または最初の曲線モーメントによって決定されます。このアプローチは、詳細な構造モデルまたは特定の気質関数に依存しない一般的な非補数分析を表します。薬物分布のダイナミクスを特徴付けるために2つのパラメーターが導入されています。(i)瞬間分布平衡(VDRTに関連する)の「よく混合された」挙動からの出発の程度(ii)平衡が達成されるまでの平均時間(平衡平衡時間、MEQT)。両方のパラメーターは、分布のダイナミクスの定量的尺度であり、対応する非除去システム内の分布の観点から明示的な物理的意義を表示します。さらに、双様体の性質曲線のいわゆる「分布段階」は、対応する非誘発システムとハーフタイムの混合、T1/2、M = T1/2、アルファ(Vベータ/vss*)とのミキシングとの単一補助混合曲線に関連していることが示されています。結果は、それぞれ肝臓に並んだラットと無傷のラットのアセトアミノフェンについて測定された混合および処分曲線に適用されます。
Conventionally, the dynamics of distribution in the body is evaluated by the so-called distribution half-life (e.g., t1/2, alpha); but then the mean time of the distribution process is underestimated due to the influence of elimination. By contrast, information about the dynamics of distribution contained in drug disposition curves can be extracted by the second and third curve moments, parameters that are related to the variance (VDRT) and skewness (SDRT) of residence time distributions; whereas the equilibrium state characterized by the volume of distribution (Vss), is determined by the mean residence time (MDRT) or the first curve moment. The approach represents a general noncompartmental analysis that is independent of a detailed structural model or a particular disposition function. Two parameters are introduced to characterize the dynamics of drug distribution: (i) the degree of departure of the system from "well-mixed" behavior of instantaneous distribution equilibrium (related to VDRT) and (ii) the mean time until equilibration is achieved (mean equilibration time, MEQT), which additionally depends on SDRT. Both parameters are quantitative measures of the dynamics of distribution and display explicit physical significance in terms of distribution within the corresponding noneliminating system. It is further shown that the so-called "distribution phase" in biexponential disposition curves is related to a monoexponential mixing curve of its corresponding noneliminating system with an equilibration or mixing half-time, t1/2,M = t1/2,alpha (V beta/Vss*), where Vss* denotes the distribution volume of the noneliminating system. The results are applied to mixing and disposition curves measured for acetaminophen in liver-ligated and intact rats, respectively.
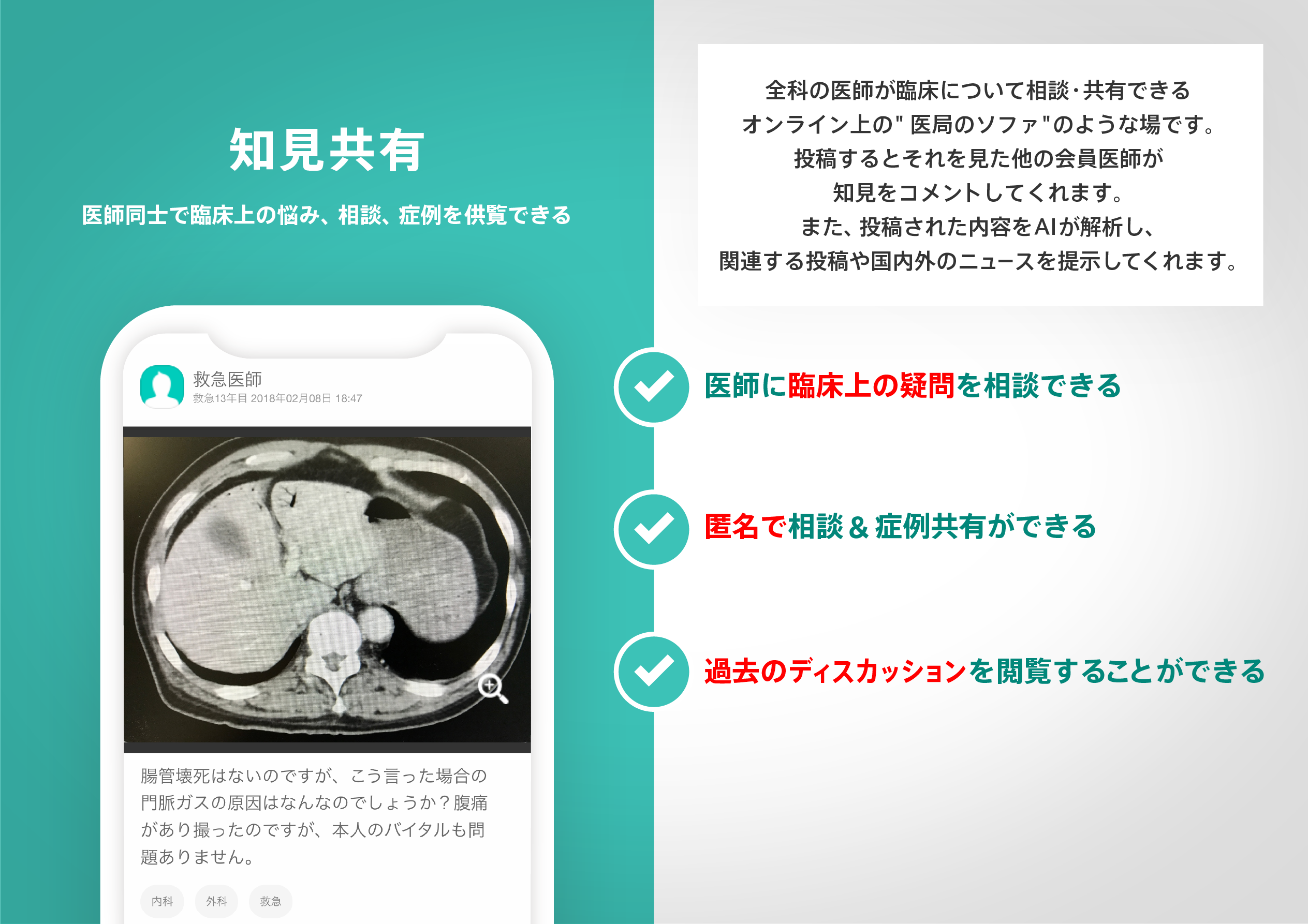
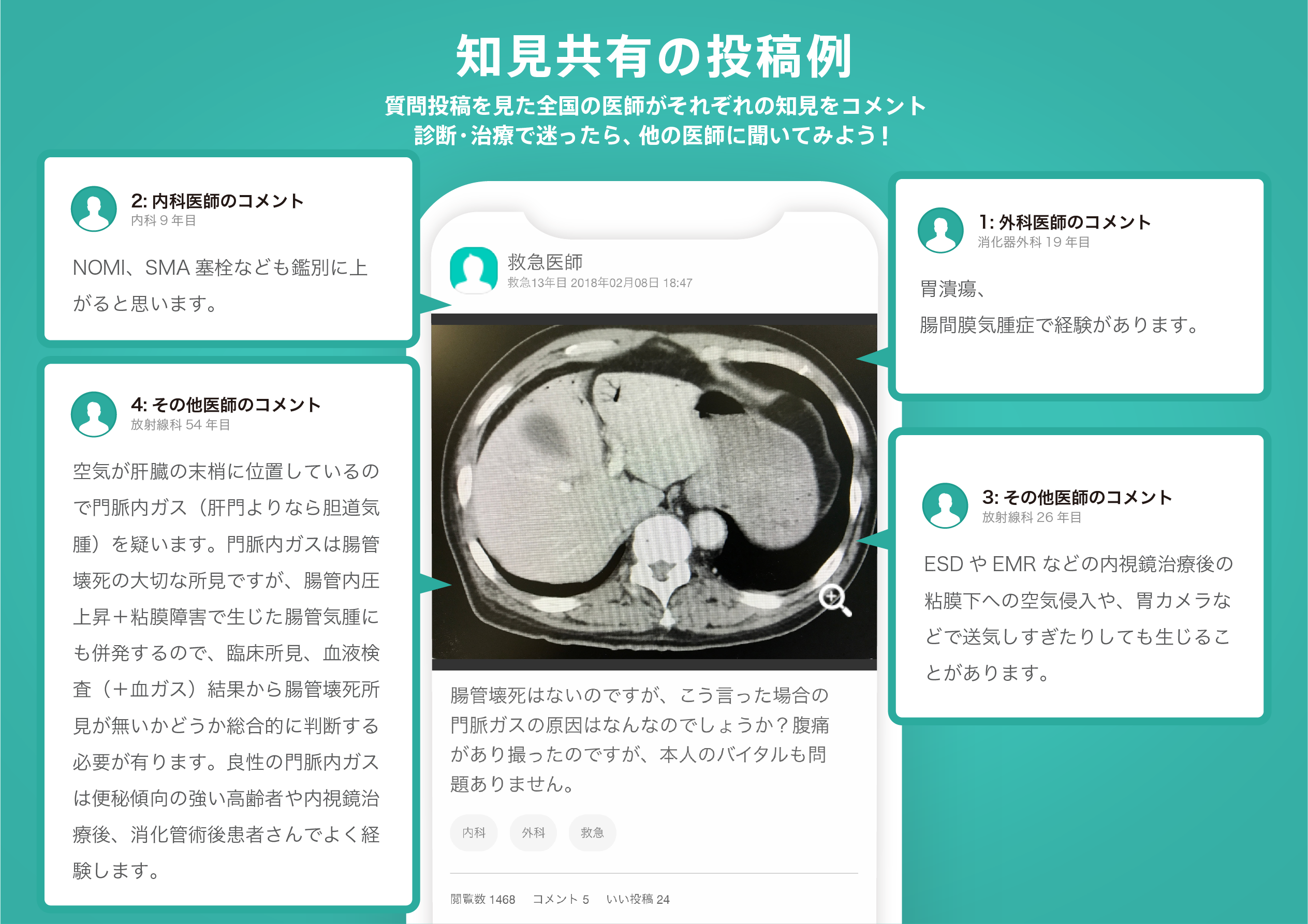
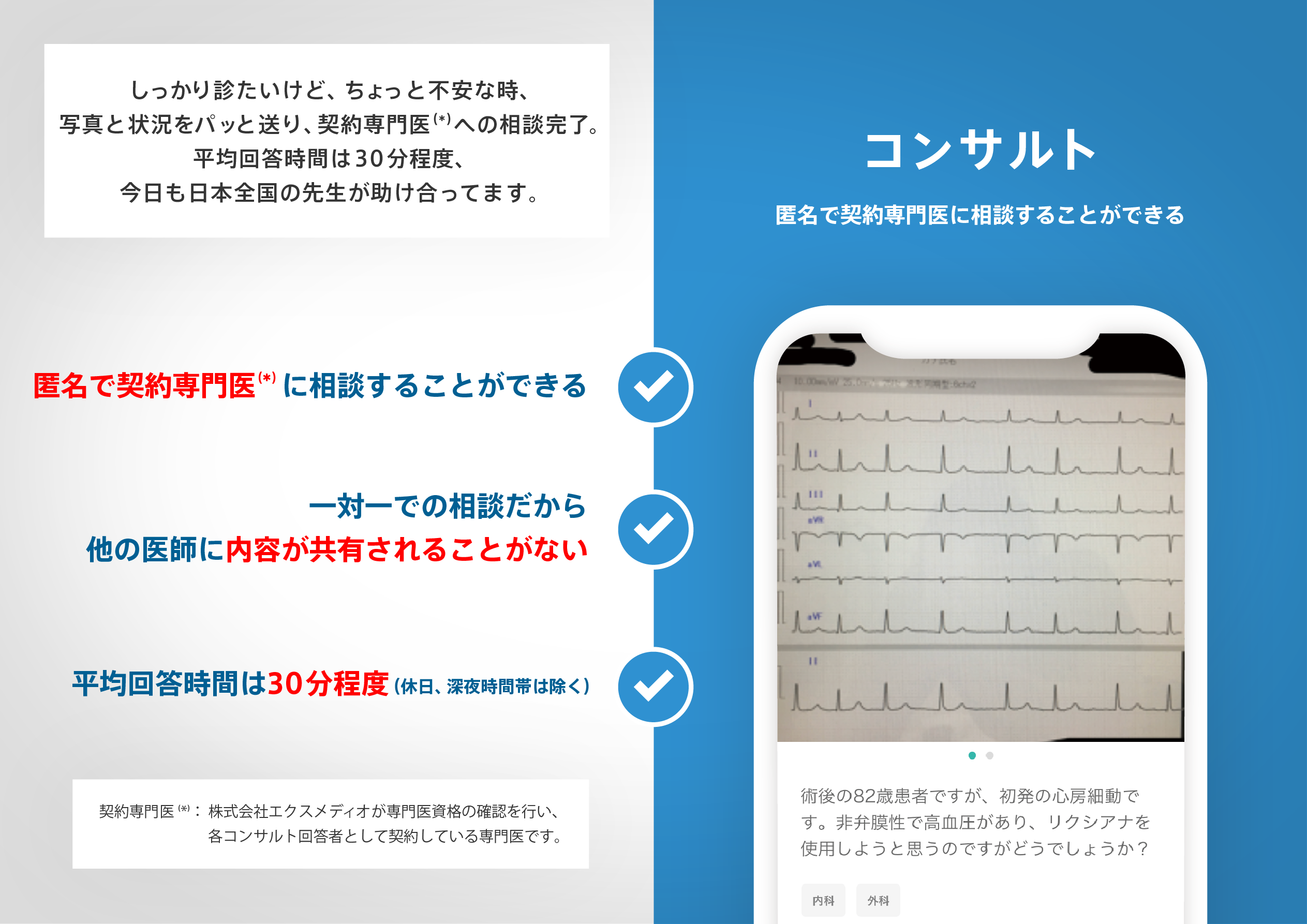
医師のための臨床サポートサービス
ヒポクラ x マイナビのご紹介
無料会員登録していただくと、さらに便利で効率的な検索が可能になります。