著名医師による解説が無料で読めます
すると翻訳の精度が向上します
ナノスケール半導体構造の電子輸送は、室温操作の下で弾道限界が実際に達成できるかどうかの問題に答えるために理論的に調査されています。半古典的なボルツマン輸送方程式は、n(+)-n-n(+)テスト構造の緩和時間近似で分析的に解決されます。ボルツマン輸送方程式の溶液は、潜在的な障壁の近くに境界層構造を示すため、活性領域の散乱はナノスケール構造でさえ、室温で室温で操作されている限り、無視できないことを実証します。
ナノスケール半導体構造の電子輸送は、室温操作の下で弾道限界が実際に達成できるかどうかの問題に答えるために理論的に調査されています。半古典的なボルツマン輸送方程式は、n(+)-n-n(+)テスト構造の緩和時間近似で分析的に解決されます。ボルツマン輸送方程式の溶液は、潜在的な障壁の近くに境界層構造を示すため、活性領域の散乱はナノスケール構造でさえ、室温で室温で操作されている限り、無視できないことを実証します。
Electron transport in nanoscale semiconductor structures is theoretically investigated to answer the question of whether or not the ballistic limit is really attainable under room temperature operation. The semiclassical Boltzmann transport equation is solved analytically under the relaxation time approximation for n(+)-n-n(+) test structures. We demonstrate that the solution of the Boltzmann transport equation exhibits a boundary layer structure near the potential barrier and thus the scatterings in the active region cannot be neglected even in nanoscale structures, as far as they are operated at room temperature under high applied voltages.
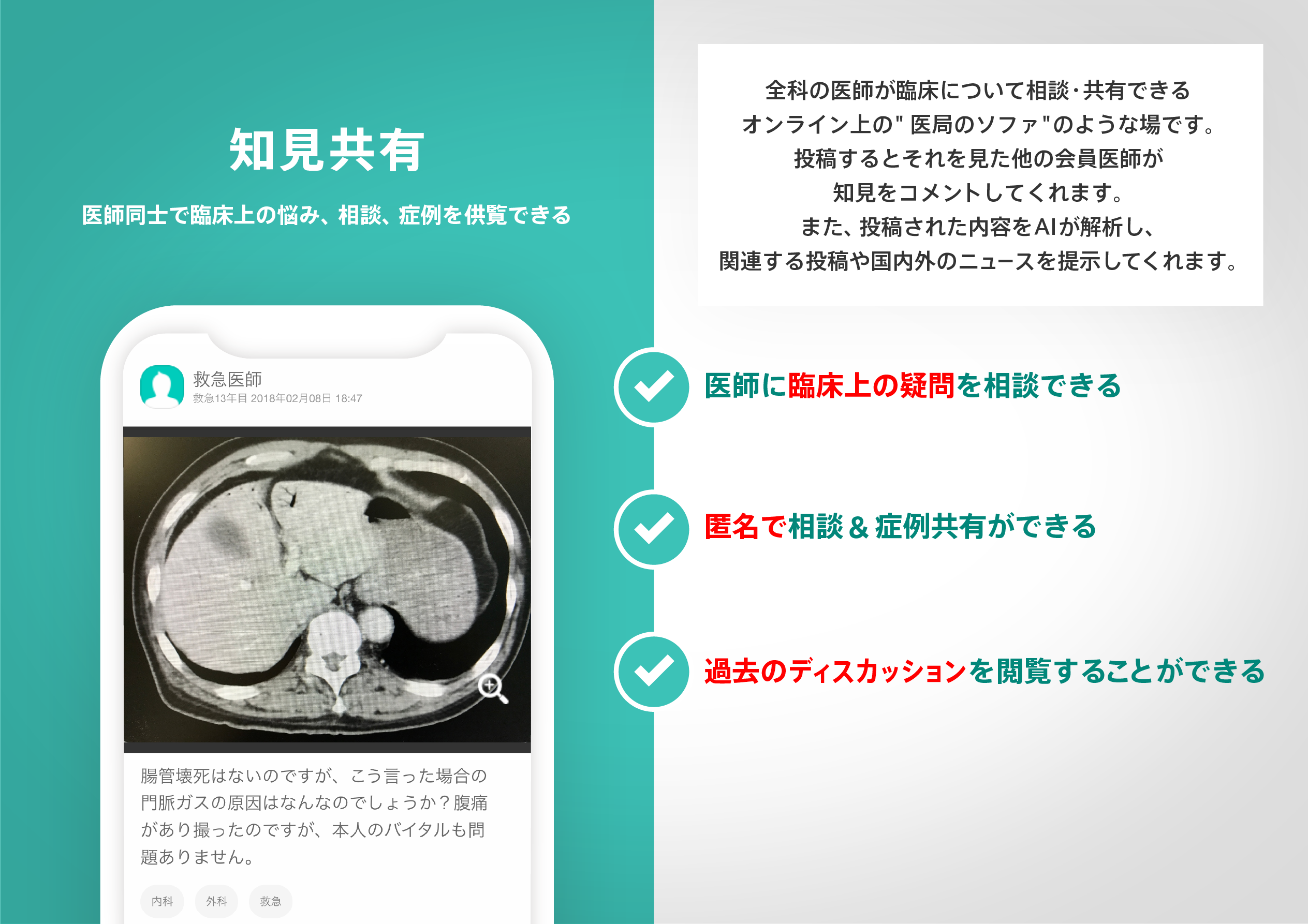
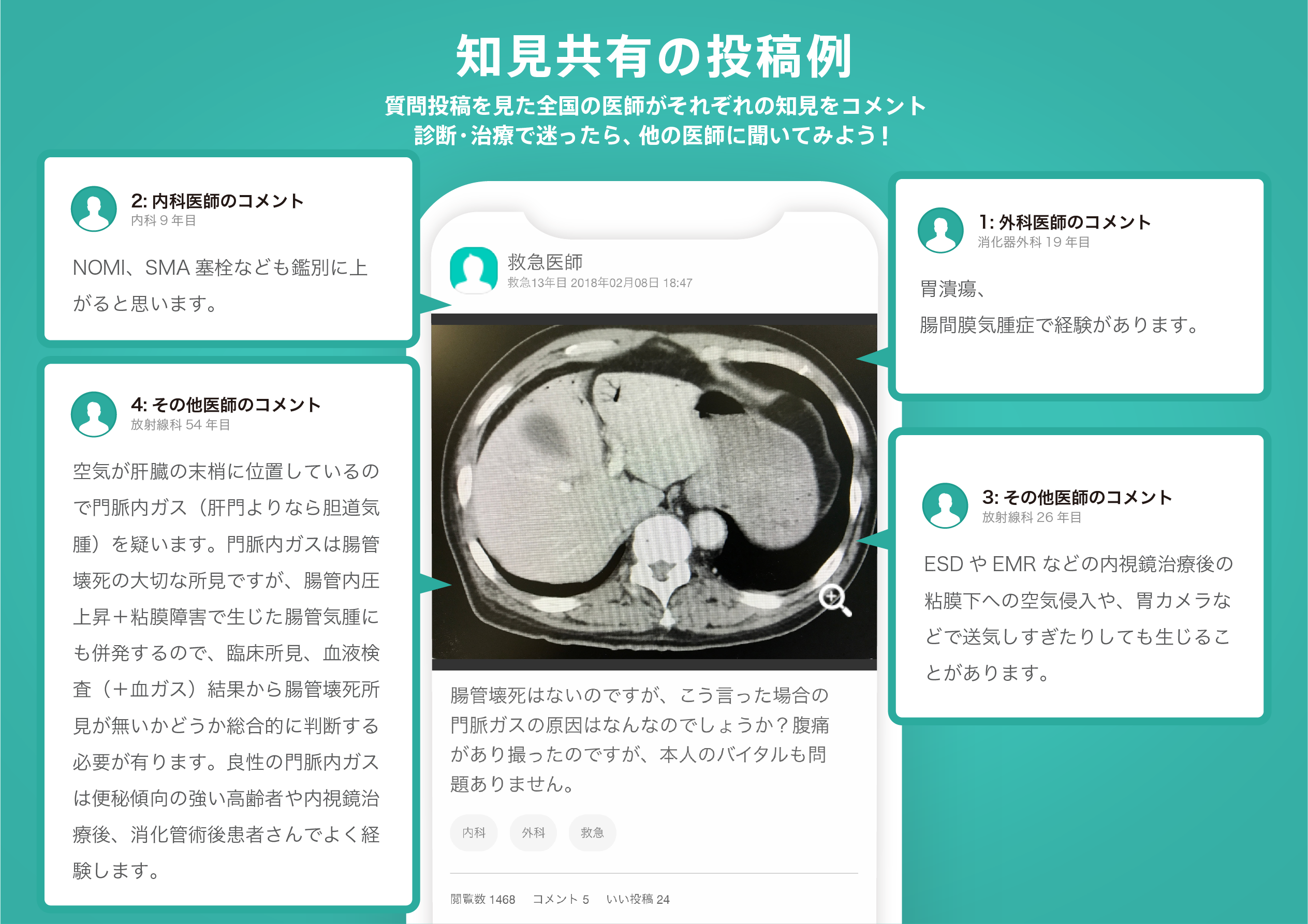
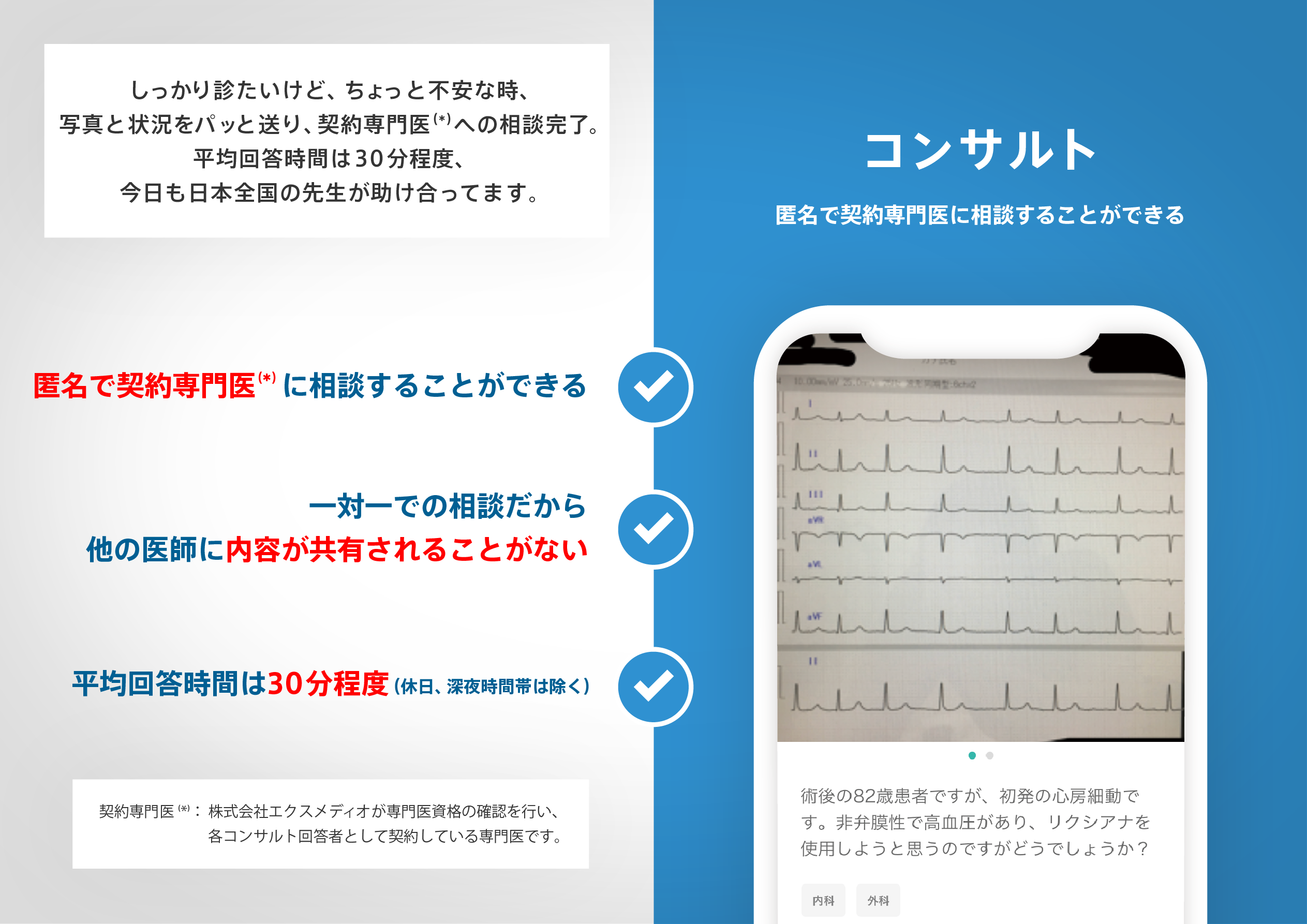
医師のための臨床サポートサービス
ヒポクラ x マイナビのご紹介
無料会員登録していただくと、さらに便利で効率的な検索が可能になります。