著名医師による解説が無料で読めます
すると翻訳の精度が向上します
発生率の用量反応関係は、量子応答測定に基づいています。定義された効果は、個人に存在するか、存在しないかのいずれかです。したがって、用量凝集曲線は、個々の感受性の違い(「耐性分布」)を反映しています。低用量では、より感受性のある個人のみが効果を示しますが、より耐性のある個人をグループの影響を受ける割合に募集するには、より高い用量が必要です。ここでは、そのような用量と瞬時の関係が、連続スケールで説明されている生物学的効果のメカニズムベースの用量反応関係にどのように関連しているかを分析します。例として、ホルモンまたはマイトジェンリガンド(L)による成長因子受容体(R)の占有によってトリガーされる量子効果「細胞分裂」を使用します。生物学的に効果的な用量(床)は受容体占有率(RL)です。線量床の関係は、双曲線Michaelis-Menten関数rl / rtot = l /(l + k(d))によって説明されます。用量床の関係を用量細胞分裂関係に変換するためには、用量床曲線と、有糸分裂に引き起こされる細胞間の感受性の分布を記述する関数と組み合わせる必要があります。この関数の対称的なS状の曲線を想定しました。これは、切り捨てられた正規分布によって近似されました。Michaelis-Menten関数の漸近飽和により、用量の関数として細胞分裂(発生率)を記述する複合曲線が右に歪んでいる複合曲線のために、上方線量床の関係のために。線量軸の対数変換はこの歪みを逆にし、中央の95%の入射範囲の正規分布にほぼ完全に適合します。この観察結果は、投与された用量の対数を使用して、累積正常曲線によって線量沈降関係がしばしば説明できる理由を説明するかもしれません。用量凝集関係に対する許容分布の支配的な役割は、ベッドとしてタンパク質またはDNAの付加物を使用して、線形用量床関係の例とともに示されています。個々の感受性のシグモイド分布によって重ねられ、シグモイドの用量凝集曲線の結果。直線性は観察されなくなりました。感受性の違いは、毒性のリスク評価と低用量への外挿のために常に考慮されるべきであると結論付けています。
発生率の用量反応関係は、量子応答測定に基づいています。定義された効果は、個人に存在するか、存在しないかのいずれかです。したがって、用量凝集曲線は、個々の感受性の違い(「耐性分布」)を反映しています。低用量では、より感受性のある個人のみが効果を示しますが、より耐性のある個人をグループの影響を受ける割合に募集するには、より高い用量が必要です。ここでは、そのような用量と瞬時の関係が、連続スケールで説明されている生物学的効果のメカニズムベースの用量反応関係にどのように関連しているかを分析します。例として、ホルモンまたはマイトジェンリガンド(L)による成長因子受容体(R)の占有によってトリガーされる量子効果「細胞分裂」を使用します。生物学的に効果的な用量(床)は受容体占有率(RL)です。線量床の関係は、双曲線Michaelis-Menten関数rl / rtot = l /(l + k(d))によって説明されます。用量床の関係を用量細胞分裂関係に変換するためには、用量床曲線と、有糸分裂に引き起こされる細胞間の感受性の分布を記述する関数と組み合わせる必要があります。この関数の対称的なS状の曲線を想定しました。これは、切り捨てられた正規分布によって近似されました。Michaelis-Menten関数の漸近飽和により、用量の関数として細胞分裂(発生率)を記述する複合曲線が右に歪んでいる複合曲線のために、上方線量床の関係のために。線量軸の対数変換はこの歪みを逆にし、中央の95%の入射範囲の正規分布にほぼ完全に適合します。この観察結果は、投与された用量の対数を使用して、累積正常曲線によって線量沈降関係がしばしば説明できる理由を説明するかもしれません。用量凝集関係に対する許容分布の支配的な役割は、ベッドとしてタンパク質またはDNAの付加物を使用して、線形用量床関係の例とともに示されています。個々の感受性のシグモイド分布によって重ねられ、シグモイドの用量凝集曲線の結果。直線性は観察されなくなりました。感受性の違いは、毒性のリスク評価と低用量への外挿のために常に考慮されるべきであると結論付けています。
Dose-response relationships for incidence are based on quantal response measures. A defined effect is either present or not present in an individual. The dose-incidence curve therefore reflects differences in individual susceptibility (the "tolerance distribution"). At low dose, only the more susceptible individuals manifest the effect, while higher doses are required for more resistant individuals to be recruited into the affected fraction of the group. Here, we analyze how such dose-incidence relationships are related to mechanism-based dose-response relationships for biological effects described on a continuous scale. As an example, we use the quantal effect "cell division" triggered by occupancy of growth factor receptors (R) by a hormone or mitogenic ligand (L). The biologically effective dose (BED) is receptor occupancy (RL). The dose-BED relationship is described by the hyperbolic Michaelis-Menten function, RL/Rtot = L / (L + K(D)). For the conversion of the dose-BED relationship to a dose-cell division relationship, the dose-BED curve has to be combined with a function that describes the distribution of susceptibilities among the cells to be triggered into mitosis. We assumed a symmetrical sigmoid curve for this function, approximated by a truncated normal distribution. Because of the supralinear dose-BED relationship due to the asymptotic saturation of the Michaelis-Menten function, the composite curve that describes cell division (incidence) as a function of dose becomes skewed to the right. Logarithmic transformation of the dose axis reverses this skewing and provides a nearly perfect fit to a normal distribution in the central 95% incidence range. This observation may explain why dose-incidence relationships can often be described by a cumulative normal curve using the logarithm of the administered dose. The dominant role of the tolerance distribution for dose-incidence relationships is also illustrated with the example of a linear dose-BED relationship, using adducts to protein or DNA as the BED. Superimposed by a sigmoid distribution of individual susceptibilities, a sigmoid dose-incidence curve results. Linearity is no longer observed. We conclude that differences in susceptibility should always be considered for toxicological risk assessment and extrapolation to low dose.
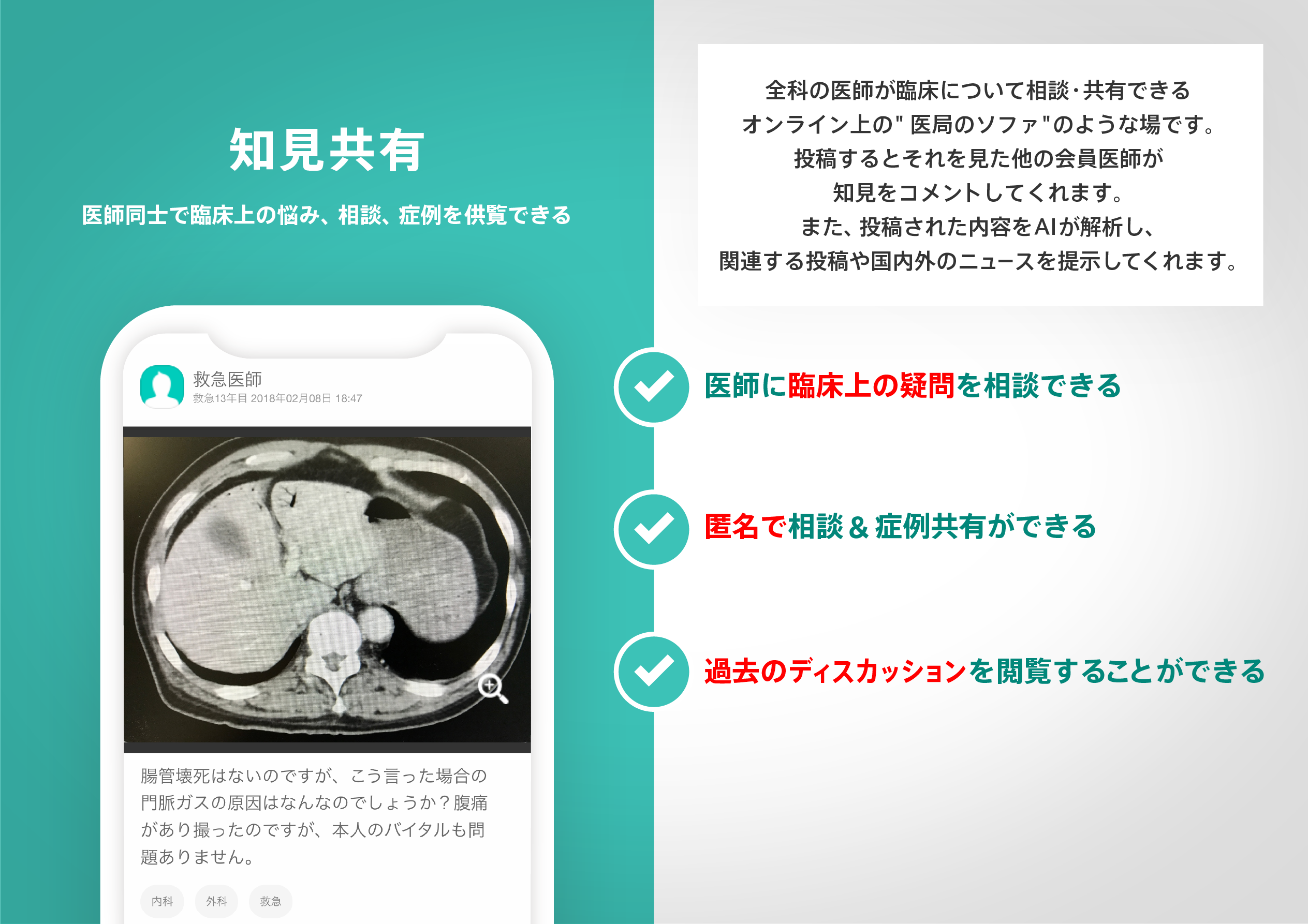
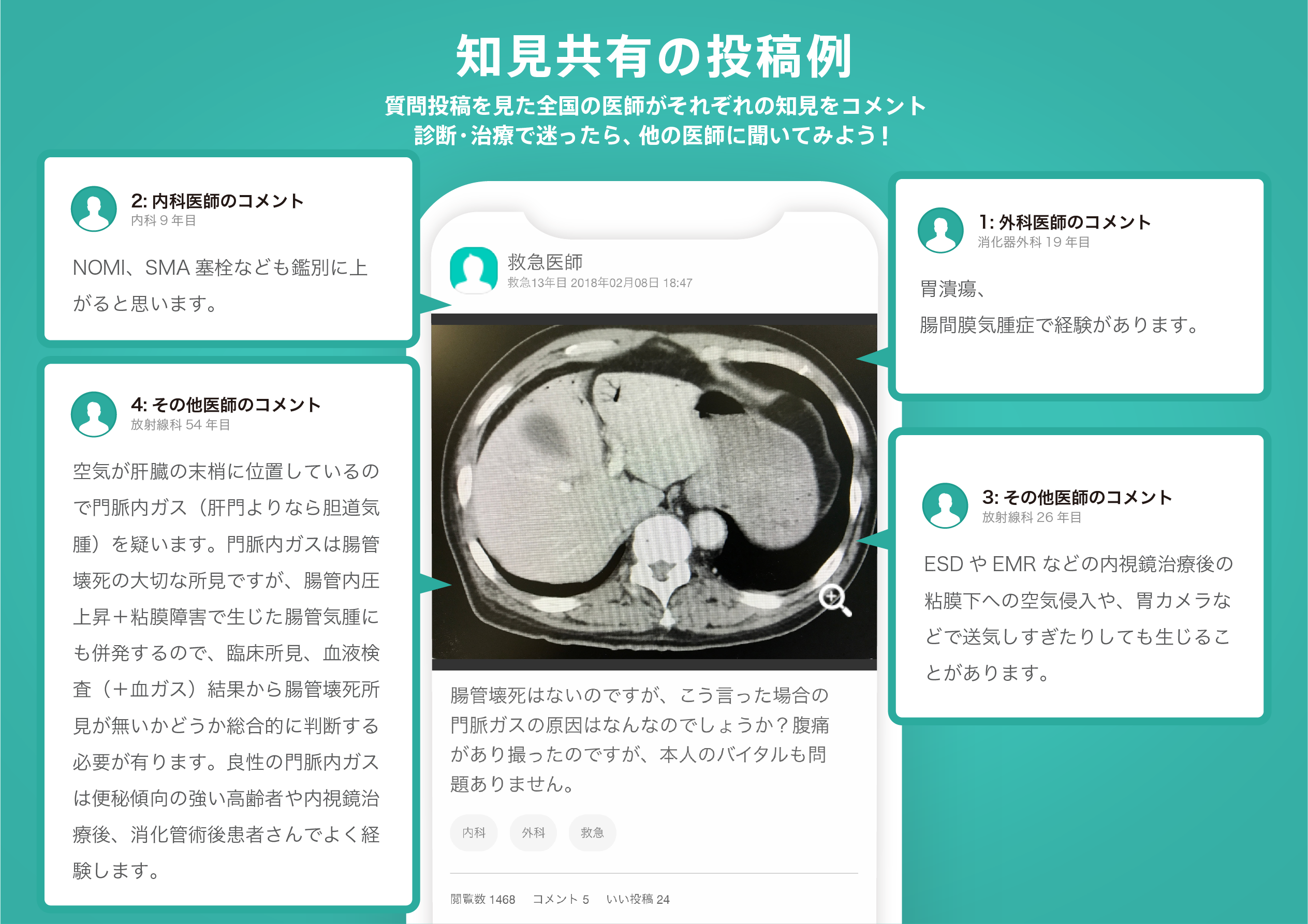
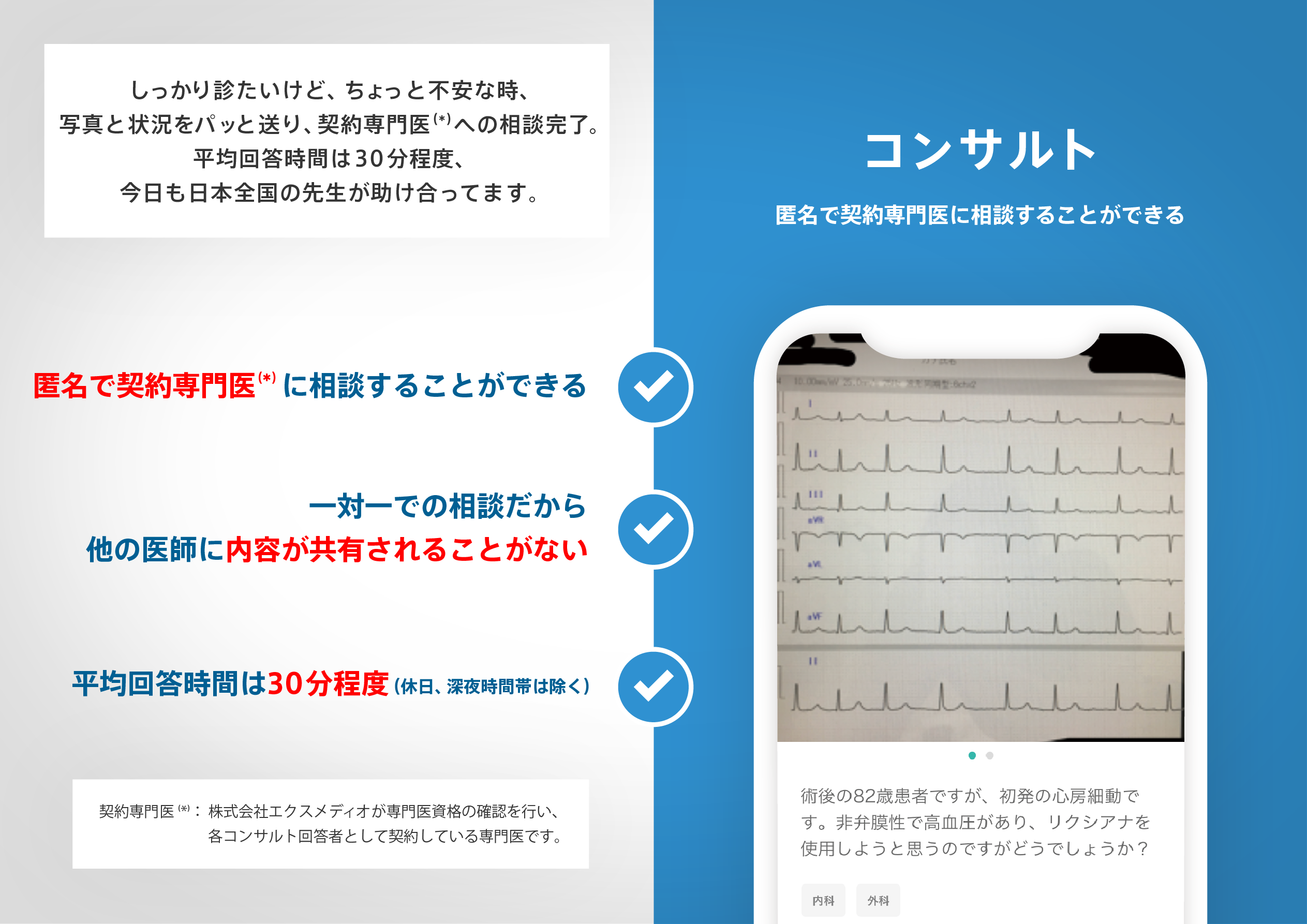
医師のための臨床サポートサービス
ヒポクラ x マイナビのご紹介
無料会員登録していただくと、さらに便利で効率的な検索が可能になります。