著名医師による解説が無料で読めます
すると翻訳の精度が向上します
磁気共鳴(MR)画像におけるバイアスフィールド補正のための新規の高速エントロピー最小化アルゴリズムは、フェーズドアレイコイルの使用に問題となっているMR画像の強度の不均一性分解を修正するために提案されています。従来のアルゴリズムに4つの重要な変更が行われました。(a)積み上げられた2D画像データセットの修正された2段階サンプリング戦略の実装。これには、数値を変更せずに各スライスの測定画像のサイズを単純な平均化方法で縮小することが含まれます。スライスの後、ヒストグラムしきい値法によって生成されたバイナリマスクを使用して、縮小画像でサンプリングされたボクセルを定義します。(b)Orthogonal Base関数多項式としてLegendRE多項式を使用することによる補正関数の効率の改善。(c)イメージデータから直接確率密度関数とシャノンエントロピーを計算するために、ガウスカーネルを使用したノンパラメトリックパルゼンウィンドウ推定器の使用。(d)コンジュゲート勾配法でエントロピー最小化を実行します。結果は、このアルゴリズムが、異なるフィールド強度で取得したさまざまなタイプのコイルから、非常に効率的に、そして計算負荷の減少により、さまざまなタイプのMR画像を修正できることを示しました。
磁気共鳴(MR)画像におけるバイアスフィールド補正のための新規の高速エントロピー最小化アルゴリズムは、フェーズドアレイコイルの使用に問題となっているMR画像の強度の不均一性分解を修正するために提案されています。従来のアルゴリズムに4つの重要な変更が行われました。(a)積み上げられた2D画像データセットの修正された2段階サンプリング戦略の実装。これには、数値を変更せずに各スライスの測定画像のサイズを単純な平均化方法で縮小することが含まれます。スライスの後、ヒストグラムしきい値法によって生成されたバイナリマスクを使用して、縮小画像でサンプリングされたボクセルを定義します。(b)Orthogonal Base関数多項式としてLegendRE多項式を使用することによる補正関数の効率の改善。(c)イメージデータから直接確率密度関数とシャノンエントロピーを計算するために、ガウスカーネルを使用したノンパラメトリックパルゼンウィンドウ推定器の使用。(d)コンジュゲート勾配法でエントロピー最小化を実行します。結果は、このアルゴリズムが、異なるフィールド強度で取得したさまざまなタイプのコイルから、非常に効率的に、そして計算負荷の減少により、さまざまなタイプのMR画像を修正できることを示しました。
A novel, fast entropy-minimization algorithm for bias field correction in magnetic resonance (MR) images is suggested to correct the intensity inhomogeneity degradation of MR images that has become an increasing problem with the use of phased-array coils. Four important modifications were made to the conventional algorithm: (a) implementation of a modified two-step sampling strategy for stacked 2D image data sets, which included reducing the size of the measured image on each slice with a simple averaging method without changing the number of slices and then using a binary mask generated by a histogram threshold method to define the sampled voxels in the reduced image; (b) improvement of the efficiency of the correction function by using a Legendre polynomial as an orthogonal base function polynomial; (c) use of a nonparametric Parzen window estimator with a Gaussian kernel to calculate the probability density function and Shannon entropy directly from the image data; and (d) performing entropy minimization with a conjugate gradient method. Results showed that this algorithm could correct different types of MR images from different types of coils acquired at different field strengths very efficiently and with decreased computational load.
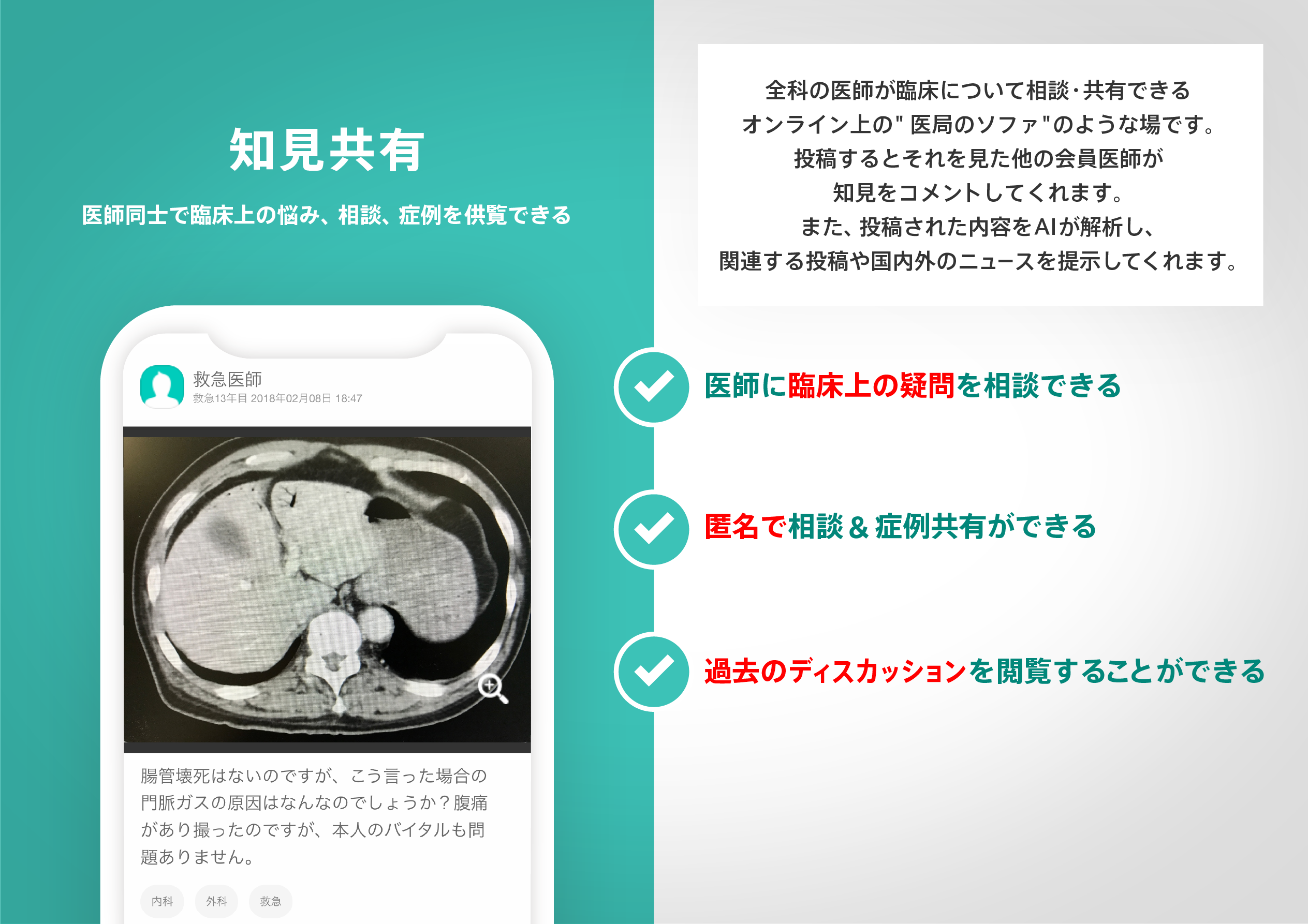
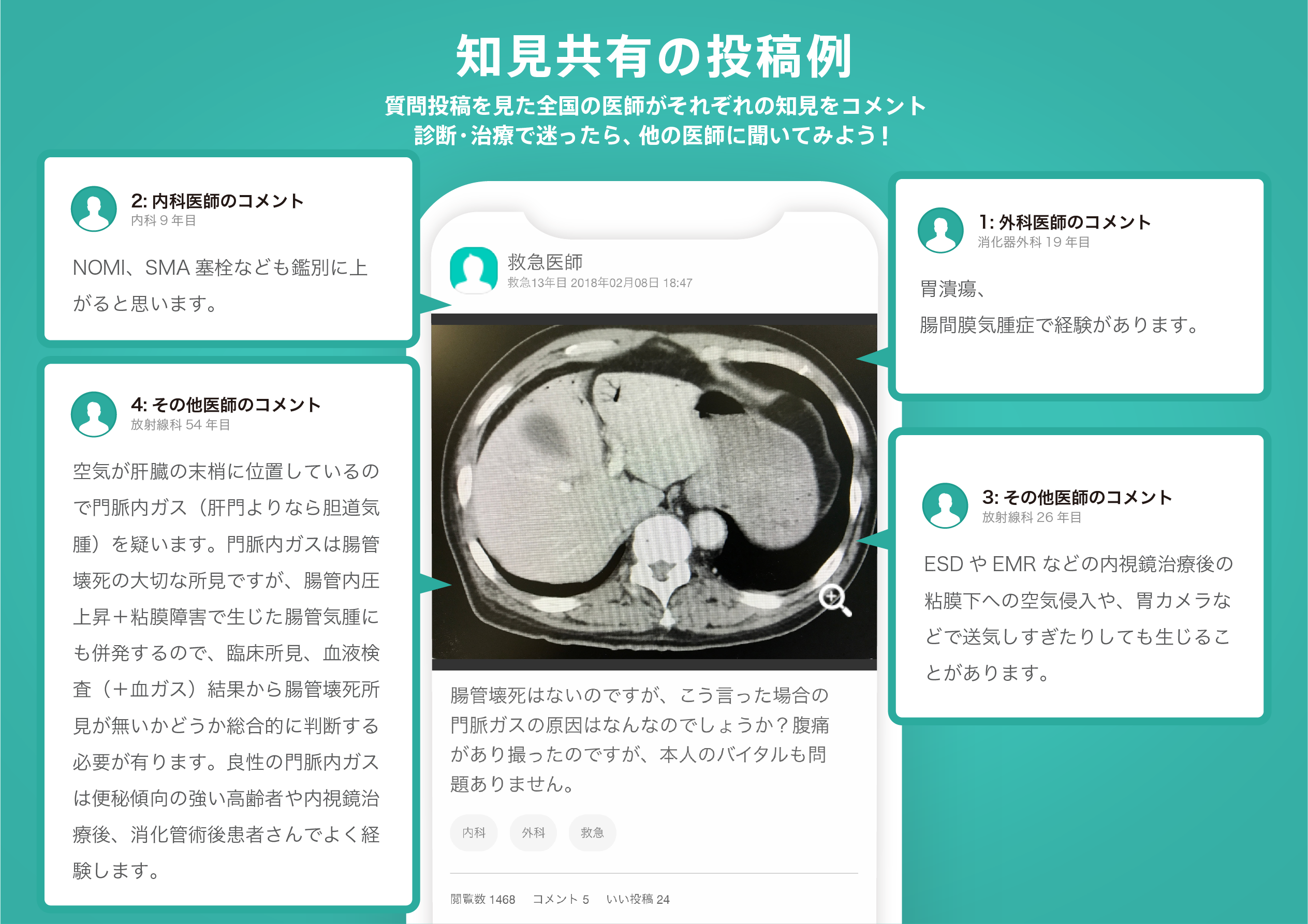
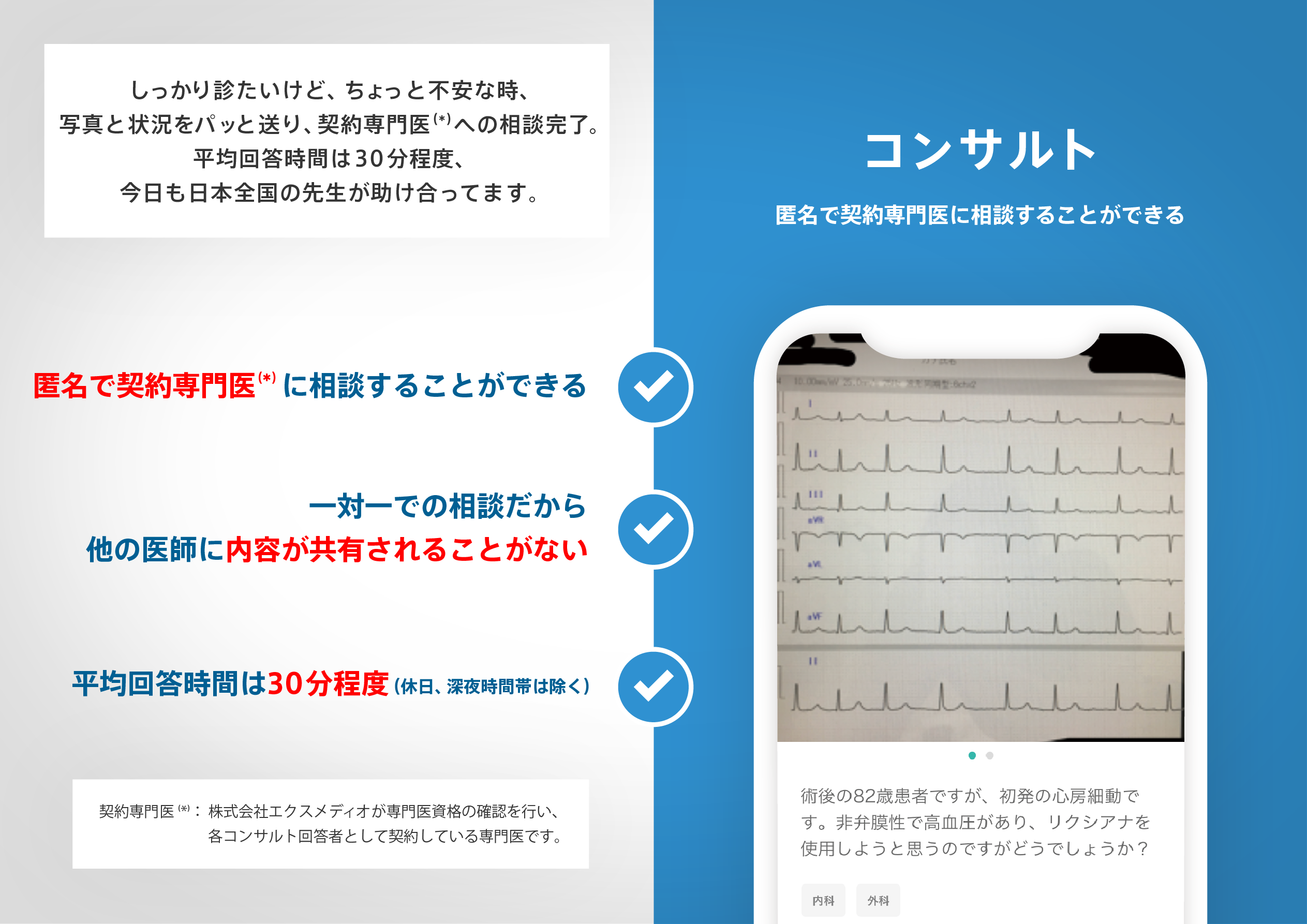
医師のための臨床サポートサービス
ヒポクラ x マイナビのご紹介
無料会員登録していただくと、さらに便利で効率的な検索が可能になります。