著名医師による解説が無料で読めます
すると翻訳の精度が向上します
Poisson Nernst-Planck(PNP)理論は、さまざまな化学的、物理的、生物学的応用の単純化された連続体モデルです。定量的な説明と実験測定のますます定性的な予測を提供する能力は、研究コミュニティで多くの認識を獲得しています。PNP方程式の解のために、多数の計算アルゴリズムが構築されています。ただし、現実的なイオンチャネルのコンテキストでは、不連続係数、特異電荷、幾何学的特異性、非線形結合など、多くの数値的障害のため、文献では2次収束PNPアルゴリズムは報告されていません。本研究では、上記の数値的課題を克服するために多くの数値アルゴリズムを導入し、イオンチャネルコンテキストで最初の2次収束PNPソルバーを構築します。第一に、タンパク質構造のために電荷特異点を軽減するように設計された、ノイマンマッピング(DNM)アルゴリズムが設計されています。さらに、PNP方程式を解くために、一致したインターフェイスと境界(MIB)メソッドが再定式化されます。MIBメソッドは、界面ジャンプ条件を体系的に強制し、分子表面の複雑なジオメトリと幾何学的特異点の存在下で2次精度を実現します。さらに、2つの反復スキームが使用され、結合された非線形方程式に対処します。さらに、現在の数値アルゴリズムの数値精度と収束順序を調べるために、球体、2つのタンパク質、イオンチャネルなど、多くの形状を介して広範かつ厳密な数値検証が実行されます。最後に、適用は実際の膜貫通タンパク質であるグラミシジンAチャネルタンパク質に考慮されます。提案された数値技術の性能は、メッシュサイズ、拡散係数プロファイル、反復スキーム、イオン濃度、適用電圧など、多くの要因に対してテストされます。数値予測は、実験測定と比較されます。
Poisson Nernst-Planck(PNP)理論は、さまざまな化学的、物理的、生物学的応用の単純化された連続体モデルです。定量的な説明と実験測定のますます定性的な予測を提供する能力は、研究コミュニティで多くの認識を獲得しています。PNP方程式の解のために、多数の計算アルゴリズムが構築されています。ただし、現実的なイオンチャネルのコンテキストでは、不連続係数、特異電荷、幾何学的特異性、非線形結合など、多くの数値的障害のため、文献では2次収束PNPアルゴリズムは報告されていません。本研究では、上記の数値的課題を克服するために多くの数値アルゴリズムを導入し、イオンチャネルコンテキストで最初の2次収束PNPソルバーを構築します。第一に、タンパク質構造のために電荷特異点を軽減するように設計された、ノイマンマッピング(DNM)アルゴリズムが設計されています。さらに、PNP方程式を解くために、一致したインターフェイスと境界(MIB)メソッドが再定式化されます。MIBメソッドは、界面ジャンプ条件を体系的に強制し、分子表面の複雑なジオメトリと幾何学的特異点の存在下で2次精度を実現します。さらに、2つの反復スキームが使用され、結合された非線形方程式に対処します。さらに、現在の数値アルゴリズムの数値精度と収束順序を調べるために、球体、2つのタンパク質、イオンチャネルなど、多くの形状を介して広範かつ厳密な数値検証が実行されます。最後に、適用は実際の膜貫通タンパク質であるグラミシジンAチャネルタンパク質に考慮されます。提案された数値技術の性能は、メッシュサイズ、拡散係数プロファイル、反復スキーム、イオン濃度、適用電圧など、多くの要因に対してテストされます。数値予測は、実験測定と比較されます。
The Poisson Nernst-Planck (PNP) theory is a simplified continuum model for a wide variety of chemical, physical and biological applications. Its ability of providing quantitative explanation and increasingly qualitative predictions of experimental measurements has earned itself much recognition in the research community. Numerous computational algorithms have been constructed for the solution of the PNP equations. However, in the realistic ion-channel context, no second order convergent PNP algorithm has ever been reported in the literature, due to many numerical obstacles, including discontinuous coefficients, singular charges, geometric singularities, and nonlinear couplings. The present work introduces a number of numerical algorithms to overcome the abovementioned numerical challenges and constructs the first second-order convergent PNP solver in the ion-channel context. First, a Dirichlet to Neumann mapping (DNM) algorithm is designed to alleviate the charge singularity due to the protein structure. Additionally, the matched interface and boundary (MIB) method is reformulated for solving the PNP equations. The MIB method systematically enforces the interface jump conditions and achieves the second order accuracy in the presence of complex geometry and geometric singularities of molecular surfaces. Moreover, two iterative schemes are utilized to deal with the coupled nonlinear equations. Furthermore, extensive and rigorous numerical validations are carried out over a number of geometries, including a sphere, two proteins and an ion channel, to examine the numerical accuracy and convergence order of the present numerical algorithms. Finally, application is considered to a real transmembrane protein, the Gramicidin A channel protein. The performance of the proposed numerical techniques is tested against a number of factors, including mesh sizes, diffusion coefficient profiles, iterative schemes, ion concentrations, and applied voltages. Numerical predictions are compared with experimental measurements.
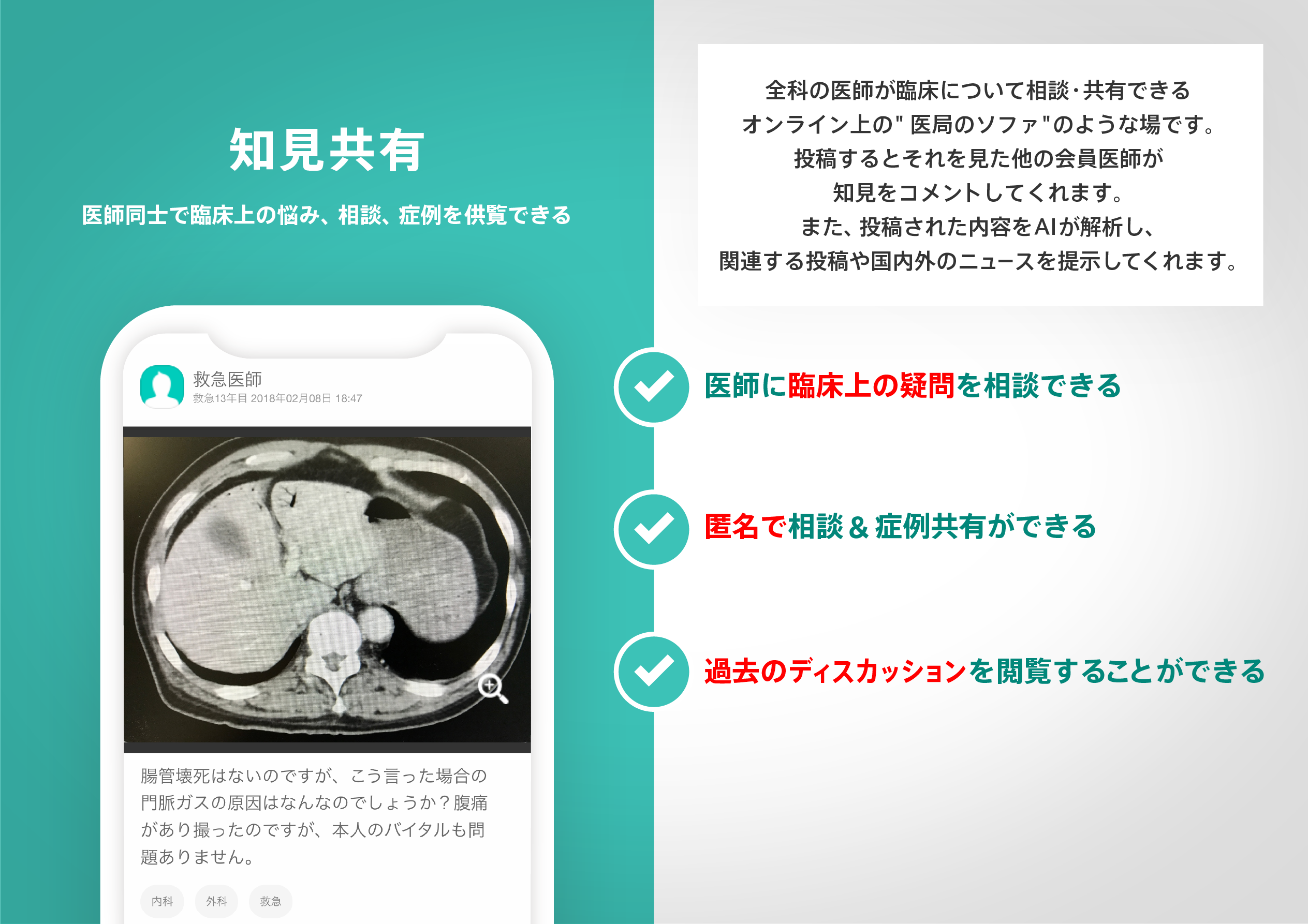
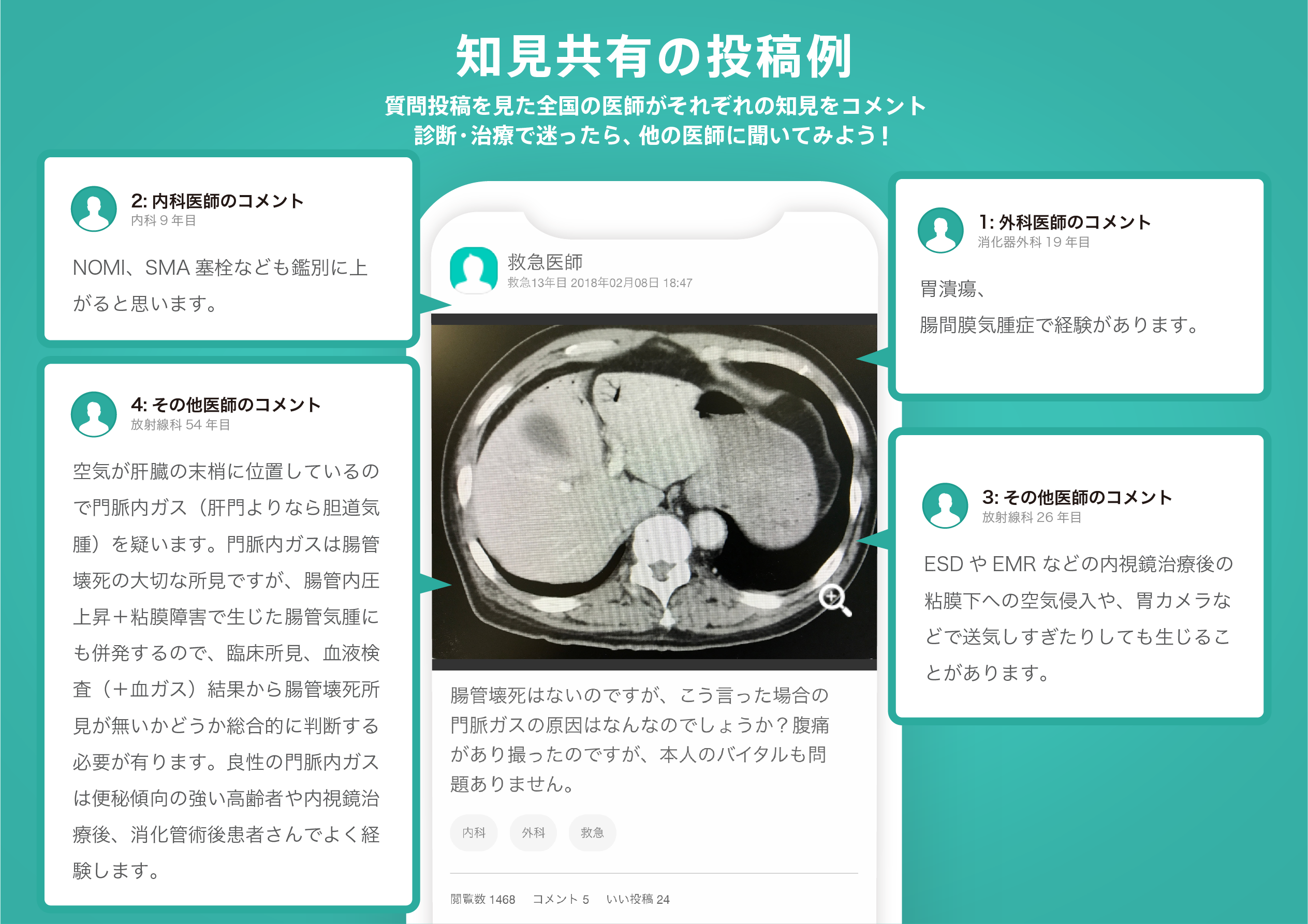
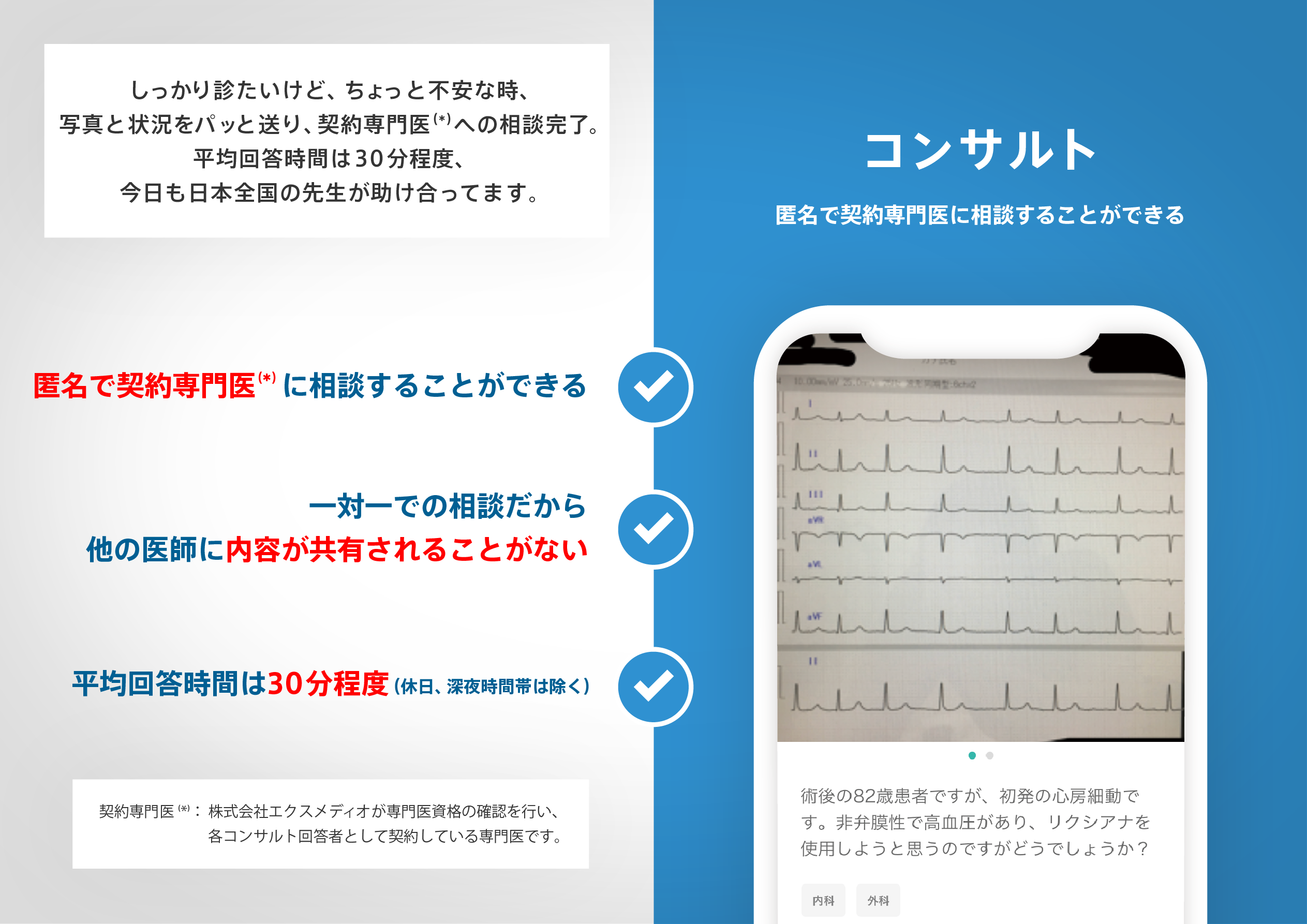
医師のための臨床サポートサービス
ヒポクラ x マイナビのご紹介
無料会員登録していただくと、さらに便利で効率的な検索が可能になります。