著名医師による解説が無料で読めます
すると翻訳の精度が向上します
生物学的動的システムにおける平衡点の分析は、人口遺伝学、理論生態学、進化的ゲーム理論など、生物学へのさまざまな数学的アプローチに非常に興味深いものです。平衡の最大数と安定性に基づくそれらの分類は、これらの研究の主要な主題であり、たとえば複数の戦略を備えた2人のゲームのコンテキストでの主題でした。ここでは、進化的ゲーム理論をツールとして使用して別の質問に対処します。ペイオフ行列が任意の分布からランダムに描画されている場合、一定数の(安定した)平衡を観察する確率は何ですか?複数の戦略を備えたマルチプレイヤーゲームの完全な複雑さに対処することにより、集団遺伝学の単一の二倍体軌跡に対応する2つのプレイヤーフレームワークの以前の結果のドメインを拡張します。最後に、アプリケーションについて説明し、生存率選択モデルの分離固定ポイントの最大数に関する有名なフェルドマンカルリンの推測など、平衡の数に関する以前の結果を説明し、結果に基づく特別なケースとして取得できることを説明します。マルチプレイヤーの進化ゲームについて。また、プレーヤーの数と戦略の数を増やすにつれて、特定の数の平衡が変化する可能性がどのように変化するかを示します。
生物学的動的システムにおける平衡点の分析は、人口遺伝学、理論生態学、進化的ゲーム理論など、生物学へのさまざまな数学的アプローチに非常に興味深いものです。平衡の最大数と安定性に基づくそれらの分類は、これらの研究の主要な主題であり、たとえば複数の戦略を備えた2人のゲームのコンテキストでの主題でした。ここでは、進化的ゲーム理論をツールとして使用して別の質問に対処します。ペイオフ行列が任意の分布からランダムに描画されている場合、一定数の(安定した)平衡を観察する確率は何ですか?複数の戦略を備えたマルチプレイヤーゲームの完全な複雑さに対処することにより、集団遺伝学の単一の二倍体軌跡に対応する2つのプレイヤーフレームワークの以前の結果のドメインを拡張します。最後に、アプリケーションについて説明し、生存率選択モデルの分離固定ポイントの最大数に関する有名なフェルドマンカルリンの推測など、平衡の数に関する以前の結果を説明し、結果に基づく特別なケースとして取得できることを説明します。マルチプレイヤーの進化ゲームについて。また、プレーヤーの数と戦略の数を増やすにつれて、特定の数の平衡が変化する可能性がどのように変化するかを示します。
The analysis of equilibrium points in biological dynamical systems has been of great interest in a variety of mathematical approaches to biology, such as population genetics, theoretical ecology or evolutionary game theory. The maximal number of equilibria and their classification based on stability have been the primary subjects of these studies, for example in the context of two-player games with multiple strategies. Herein, we address a different question using evolutionary game theory as a tool. If the payoff matrices are drawn randomly from an arbitrary distribution, what are the probabilities of observing a certain number of (stable) equilibria? We extend the domain of previous results for the two-player framework, which corresponds to a single diploid locus in population genetics, by addressing the full complexity of multi-player games with multiple strategies. In closing, we discuss an application and illustrate how previous results on the number of equilibria, such as the famous Feldman-Karlin conjecture on the maximal number of isolated fixed points in a viability selection model, can be obtained as special cases of our results based on multi-player evolutionary games. We also show how the probability of realizing a certain number of equilibria changes as we increase the number of players and number of strategies.
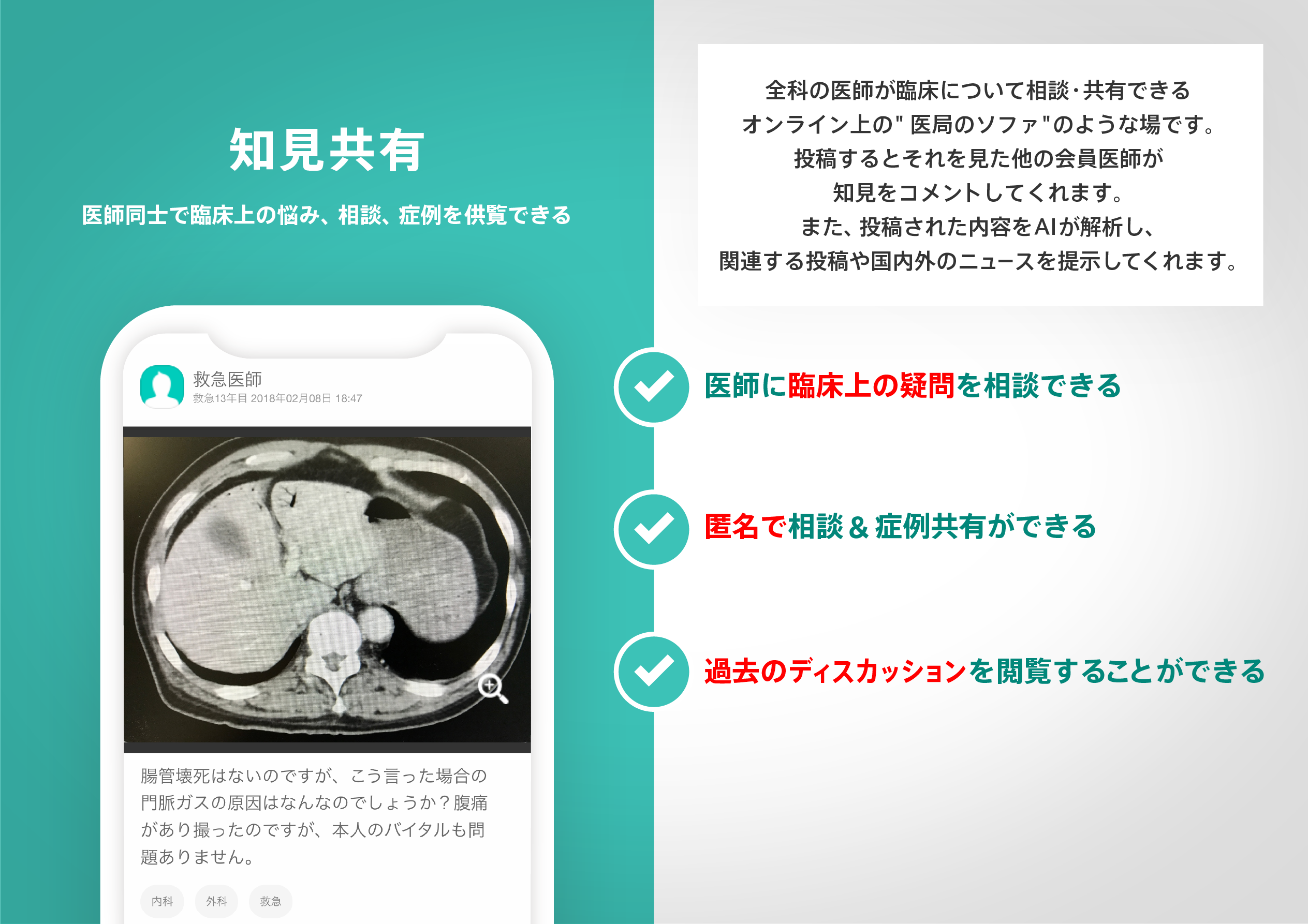
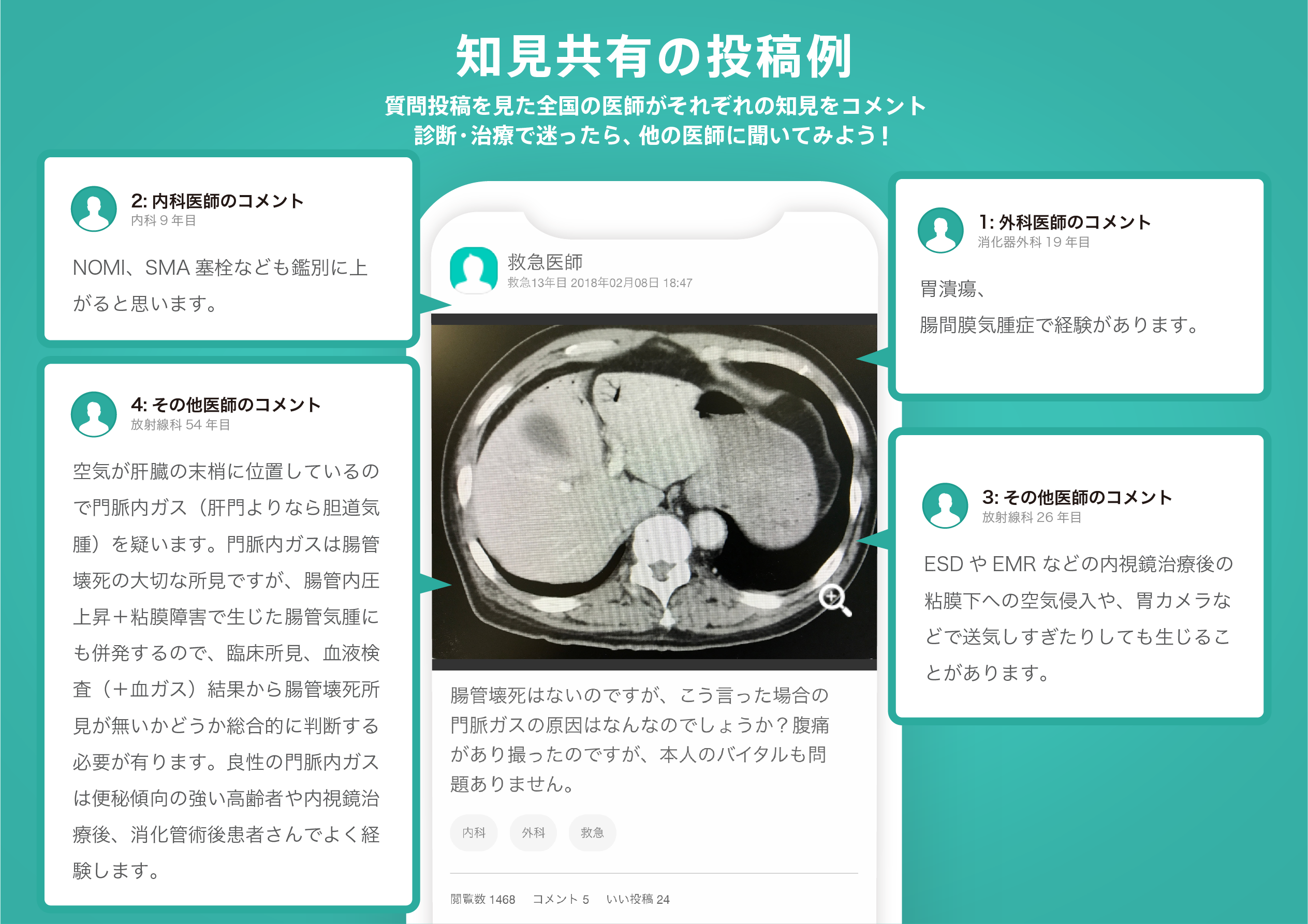
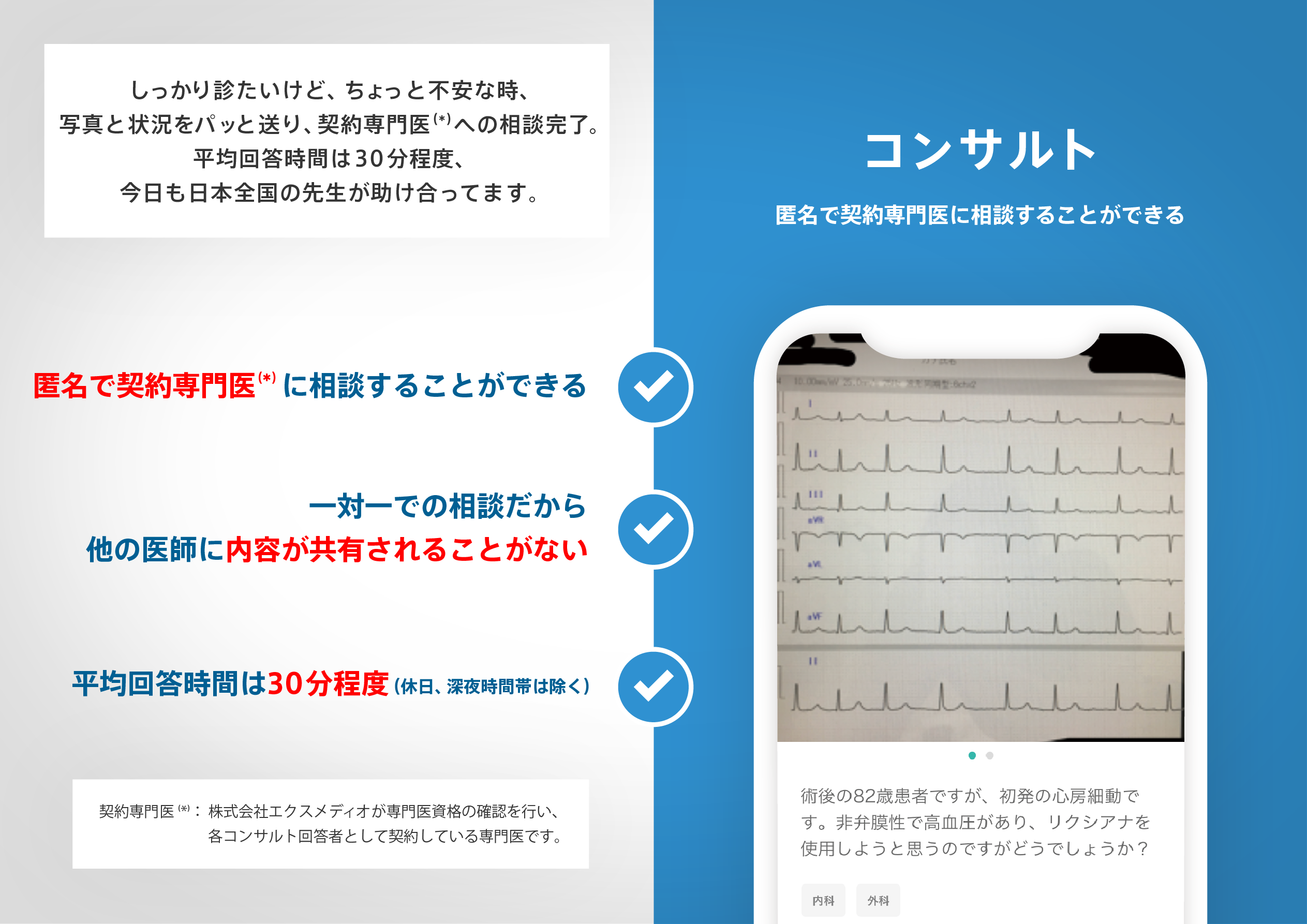
医師のための臨床サポートサービス
ヒポクラ x マイナビのご紹介
無料会員登録していただくと、さらに便利で効率的な検索が可能になります。