著名医師による解説が無料で読めます
すると翻訳の精度が向上します
ここでは、数値シミュレーションの結果と、重尾の確率分布関数を使用した1次元ランダム変動のスケーリング特性を示します。ランダム変動の分布関数が電力法のスケーリング指数を使用してレビー統計に従うと仮定すると、μ安定性レビーノイズの存在下での分数拡散方程式を調査します。グローバル幅と2点相関関数のスケーリング特性を研究し、成長指数βと粗さ指数αの分析結果と数値結果を比較します。また、重度の尾のランダム変動について、断片的なFokker-Planck方程式を調査します。μ安定性レビーノイズの存在下での分数拡散プロセスが、確率分布関数(PDF)に特別なスケーリング特性を表示することを示します。最後に、拡散エントロピー解析を使用して、重尾のランダム変動のスケーリング特性を数値的に研究します。この方法は、プロセスのグローバルな幅の測定ではなく、ランダム変動によって生成されたPDFのシャノンエントロピーの評価に基づいています。拡散エントロピー分析を適用して、成長指数βを抽出し、数値分析の妥当性を確認します。
ここでは、数値シミュレーションの結果と、重尾の確率分布関数を使用した1次元ランダム変動のスケーリング特性を示します。ランダム変動の分布関数が電力法のスケーリング指数を使用してレビー統計に従うと仮定すると、μ安定性レビーノイズの存在下での分数拡散方程式を調査します。グローバル幅と2点相関関数のスケーリング特性を研究し、成長指数βと粗さ指数αの分析結果と数値結果を比較します。また、重度の尾のランダム変動について、断片的なFokker-Planck方程式を調査します。μ安定性レビーノイズの存在下での分数拡散プロセスが、確率分布関数(PDF)に特別なスケーリング特性を表示することを示します。最後に、拡散エントロピー解析を使用して、重尾のランダム変動のスケーリング特性を数値的に研究します。この方法は、プロセスのグローバルな幅の測定ではなく、ランダム変動によって生成されたPDFのシャノンエントロピーの評価に基づいています。拡散エントロピー分析を適用して、成長指数βを抽出し、数値分析の妥当性を確認します。
Here, we present results of numerical simulations and the scaling characteristics of one-dimensional random fluctuations with heavy-tailed probability distribution functions. Assuming that the distribution function of the random fluctuations obeys Lévy statistics with a power-law scaling exponent, we investigate the fractional diffusion equation in the presence of μ-stable Lévy noise. We study the scaling properties of the global width and two-point correlation functions and then compare the analytical and numerical results for the growth exponent β and the roughness exponent α. We also investigate the fractional Fokker-Planck equation for heavy-tailed random fluctuations. We show that the fractional diffusion processes in the presence of μ-stable Lévy noise display special scaling properties in the probability distribution function (PDF). Finally, we numerically study the scaling properties of the heavy-tailed random fluctuations by using the diffusion entropy analysis. This method is based on the evaluation of the Shannon entropy of the PDF generated by the random fluctuations, rather than on the measurement of the global width of the process. We apply the diffusion entropy analysis to extract the growth exponent β and to confirm the validity of our numerical analysis.
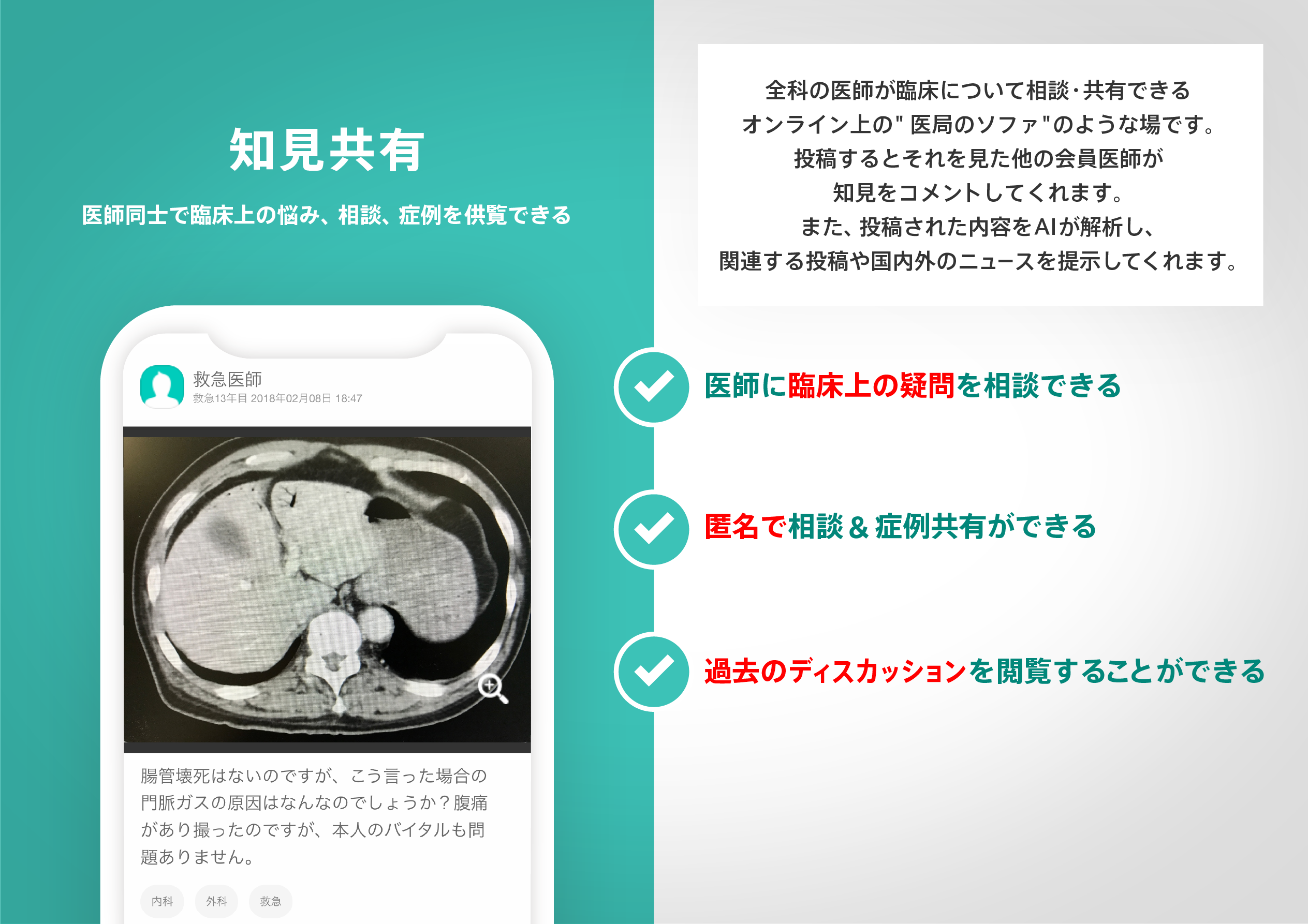
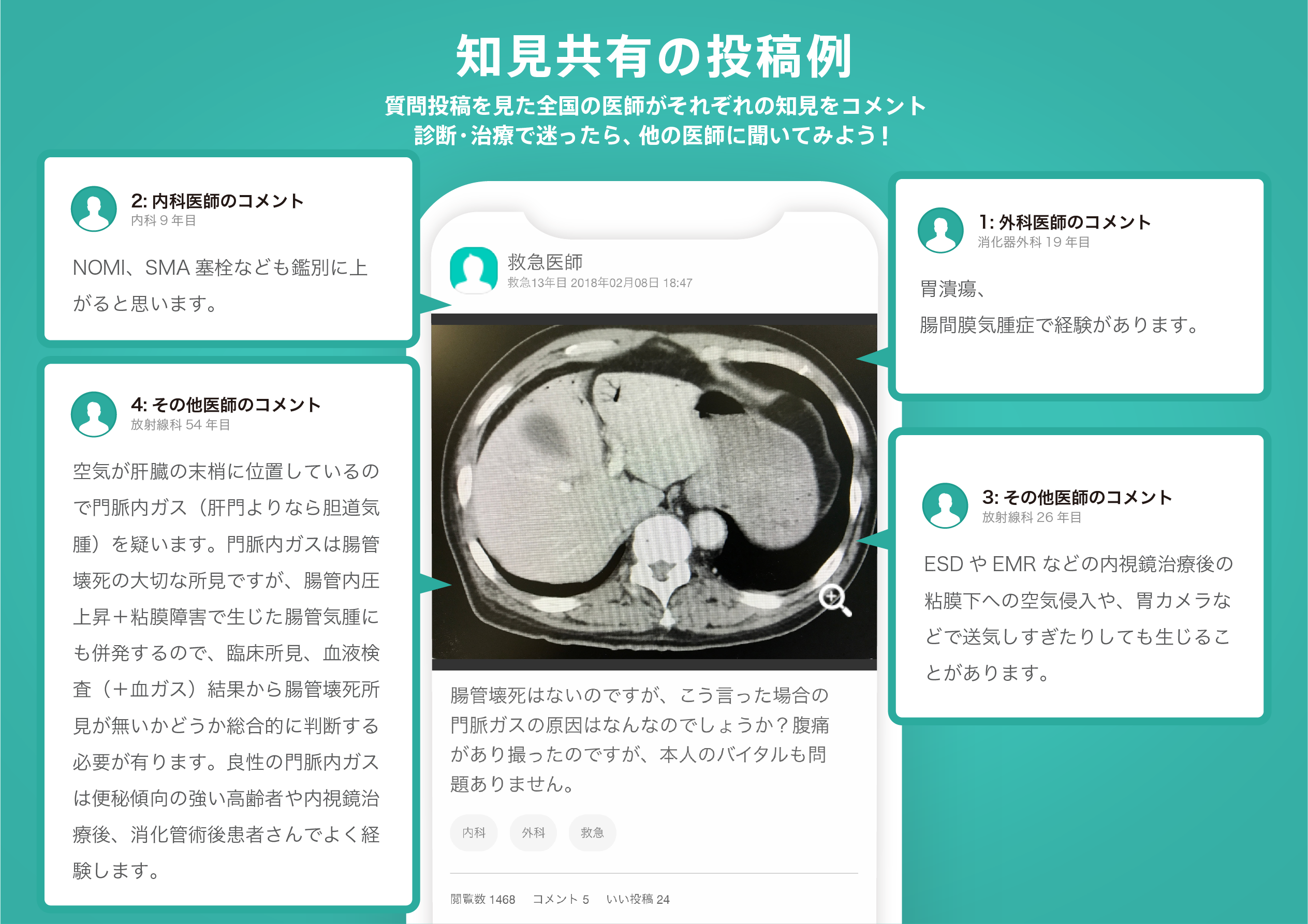
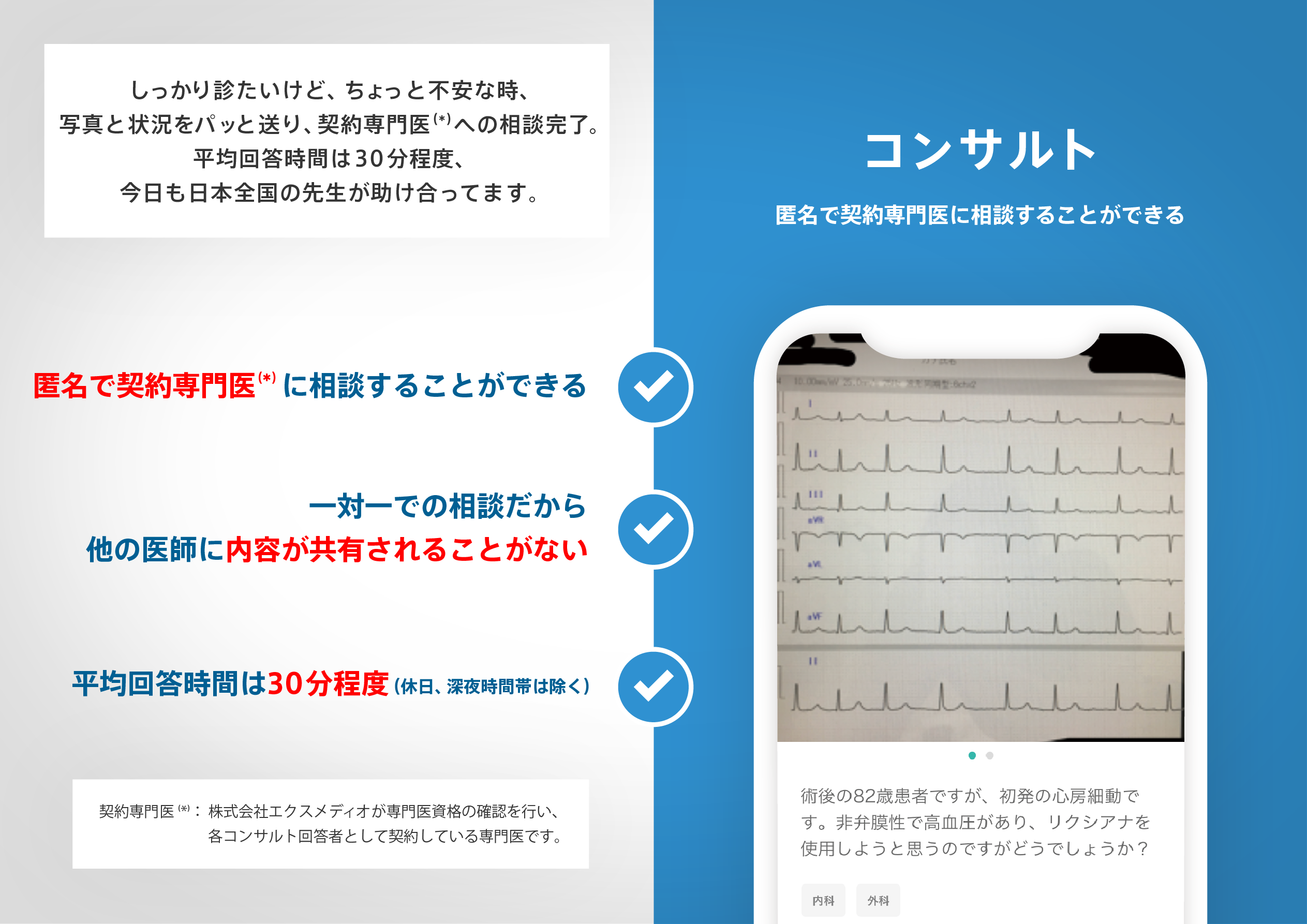
医師のための臨床サポートサービス
ヒポクラ x マイナビのご紹介
無料会員登録していただくと、さらに便利で効率的な検索が可能になります。