著名医師による解説が無料で読めます
すると翻訳の精度が向上します
背景:厳密で有益なメタ分析は、適切な要約統計または個々の参加者データの可用性に依存しています。継続的な結果、特に自然に歪んだ分布を持つ結果の場合、平均または変動性に関する要約情報はしばしば報告されません。元の試験データの完全な報告が理想的ですが、メタ分析の報告されていない平均または変動の要約統計を処理する方法を特定しようとしました。 方法:2つの体系的な文献レビューを実施して、欠落している平均または変動の要約統計に対処するために使用される方法論的アプローチを特定しました。Cochrane Colloquium Abstract BooksとCochrane Statistics Methods Group Mailing List Archiveに加えて、5つの電子データベースが検索されました。また、引用された参照検索を実施し、最近の方法論的発展を特定するためにトピックの専門家に電子メールを送信しました。記録された詳細には、メソッドの説明、メソッドの実装に必要な情報、根本的な仮定、および標準統計ソフトウェアにメソッドを容易に適用できるかどうかが含まれていました。特定された方法の要約説明を提供し、メタ分析シナリオの例で選択された方法を示しました。 結果:503件の記事のスクリーニングに続いて、標準偏差(SDS)の欠落については、以前のレビューで報告されたものに加えて15の方法が特定されました。これらには、メタ分析レベルでのベイジアン階層モデリングが含まれていました。概要統計レベル代入メタ分析における他の試験からの観測されたSD値に基づいて。範囲に基づく実用的な近似。他の要約統計に基づくSDの代数推定。平均を推定する方法の1124の記事のスクリーニングに続いて、1つの近似ベイジアン計算アプローチと、代替概要統計に基づく3つの論文が特定されました。実例メタ分析は、欠落しているSDを置き換えると、範囲を最小限に抑えた精度の損失を最小限に抑え、一般に試験を省略するよりも優れたパフォーマンスを使用して近似を示しました。欠落平均を推定すると、中央値、下部四分位、および上部四分位を使用した式がメタ分析の調査結果の精度を維持するのに最適でしたが、いくつかのシナリオでは、試験を省略することで優れた結果が得られました。 結論:文献で報告されている概要統計統計(最小、最大、下部四分位、上部四分位、中央値、中央値)に基づく方法は、メタ分析内の平均または変動の概要統計が欠落しているランダム化比較試験をより包括的に含めることを促進します。
背景:厳密で有益なメタ分析は、適切な要約統計または個々の参加者データの可用性に依存しています。継続的な結果、特に自然に歪んだ分布を持つ結果の場合、平均または変動性に関する要約情報はしばしば報告されません。元の試験データの完全な報告が理想的ですが、メタ分析の報告されていない平均または変動の要約統計を処理する方法を特定しようとしました。 方法:2つの体系的な文献レビューを実施して、欠落している平均または変動の要約統計に対処するために使用される方法論的アプローチを特定しました。Cochrane Colloquium Abstract BooksとCochrane Statistics Methods Group Mailing List Archiveに加えて、5つの電子データベースが検索されました。また、引用された参照検索を実施し、最近の方法論的発展を特定するためにトピックの専門家に電子メールを送信しました。記録された詳細には、メソッドの説明、メソッドの実装に必要な情報、根本的な仮定、および標準統計ソフトウェアにメソッドを容易に適用できるかどうかが含まれていました。特定された方法の要約説明を提供し、メタ分析シナリオの例で選択された方法を示しました。 結果:503件の記事のスクリーニングに続いて、標準偏差(SDS)の欠落については、以前のレビューで報告されたものに加えて15の方法が特定されました。これらには、メタ分析レベルでのベイジアン階層モデリングが含まれていました。概要統計レベル代入メタ分析における他の試験からの観測されたSD値に基づいて。範囲に基づく実用的な近似。他の要約統計に基づくSDの代数推定。平均を推定する方法の1124の記事のスクリーニングに続いて、1つの近似ベイジアン計算アプローチと、代替概要統計に基づく3つの論文が特定されました。実例メタ分析は、欠落しているSDを置き換えると、範囲を最小限に抑えた精度の損失を最小限に抑え、一般に試験を省略するよりも優れたパフォーマンスを使用して近似を示しました。欠落平均を推定すると、中央値、下部四分位、および上部四分位を使用した式がメタ分析の調査結果の精度を維持するのに最適でしたが、いくつかのシナリオでは、試験を省略することで優れた結果が得られました。 結論:文献で報告されている概要統計統計(最小、最大、下部四分位、上部四分位、中央値、中央値)に基づく方法は、メタ分析内の平均または変動の概要統計が欠落しているランダム化比較試験をより包括的に含めることを促進します。
BACKGROUND: Rigorous, informative meta-analyses rely on availability of appropriate summary statistics or individual participant data. For continuous outcomes, especially those with naturally skewed distributions, summary information on the mean or variability often goes unreported. While full reporting of original trial data is the ideal, we sought to identify methods for handling unreported mean or variability summary statistics in meta-analysis. METHODS: We undertook two systematic literature reviews to identify methodological approaches used to deal with missing mean or variability summary statistics. Five electronic databases were searched, in addition to the Cochrane Colloquium abstract books and the Cochrane Statistics Methods Group mailing list archive. We also conducted cited reference searching and emailed topic experts to identify recent methodological developments. Details recorded included the description of the method, the information required to implement the method, any underlying assumptions and whether the method could be readily applied in standard statistical software. We provided a summary description of the methods identified, illustrating selected methods in example meta-analysis scenarios. RESULTS: For missing standard deviations (SDs), following screening of 503 articles, fifteen methods were identified in addition to those reported in a previous review. These included Bayesian hierarchical modelling at the meta-analysis level; summary statistic level imputation based on observed SD values from other trials in the meta-analysis; a practical approximation based on the range; and algebraic estimation of the SD based on other summary statistics. Following screening of 1124 articles for methods estimating the mean, one approximate Bayesian computation approach and three papers based on alternative summary statistics were identified. Illustrative meta-analyses showed that when replacing a missing SD the approximation using the range minimised loss of precision and generally performed better than omitting trials. When estimating missing means, a formula using the median, lower quartile and upper quartile performed best in preserving the precision of the meta-analysis findings, although in some scenarios, omitting trials gave superior results. CONCLUSIONS: Methods based on summary statistics (minimum, maximum, lower quartile, upper quartile, median) reported in the literature facilitate more comprehensive inclusion of randomised controlled trials with missing mean or variability summary statistics within meta-analyses.
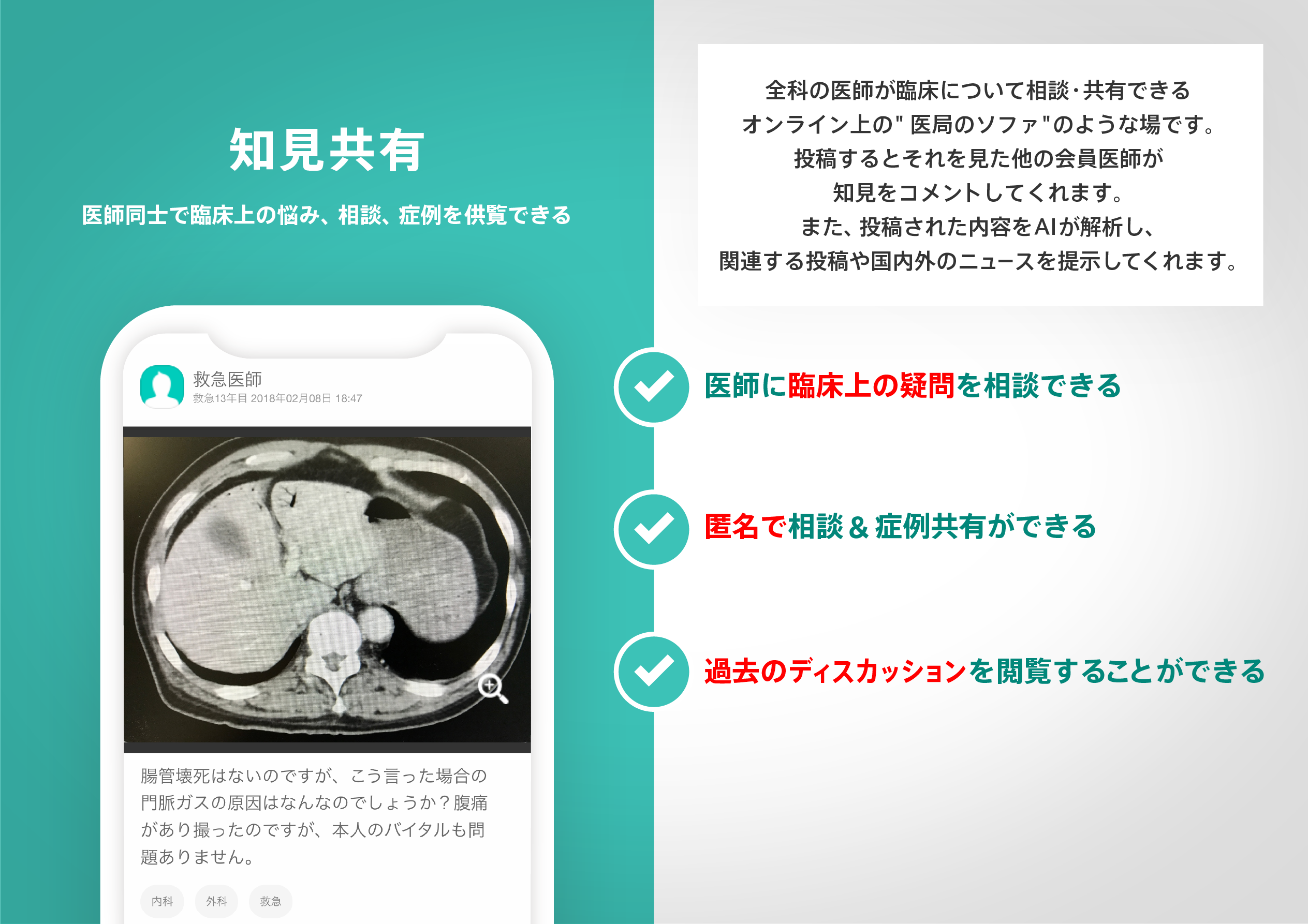
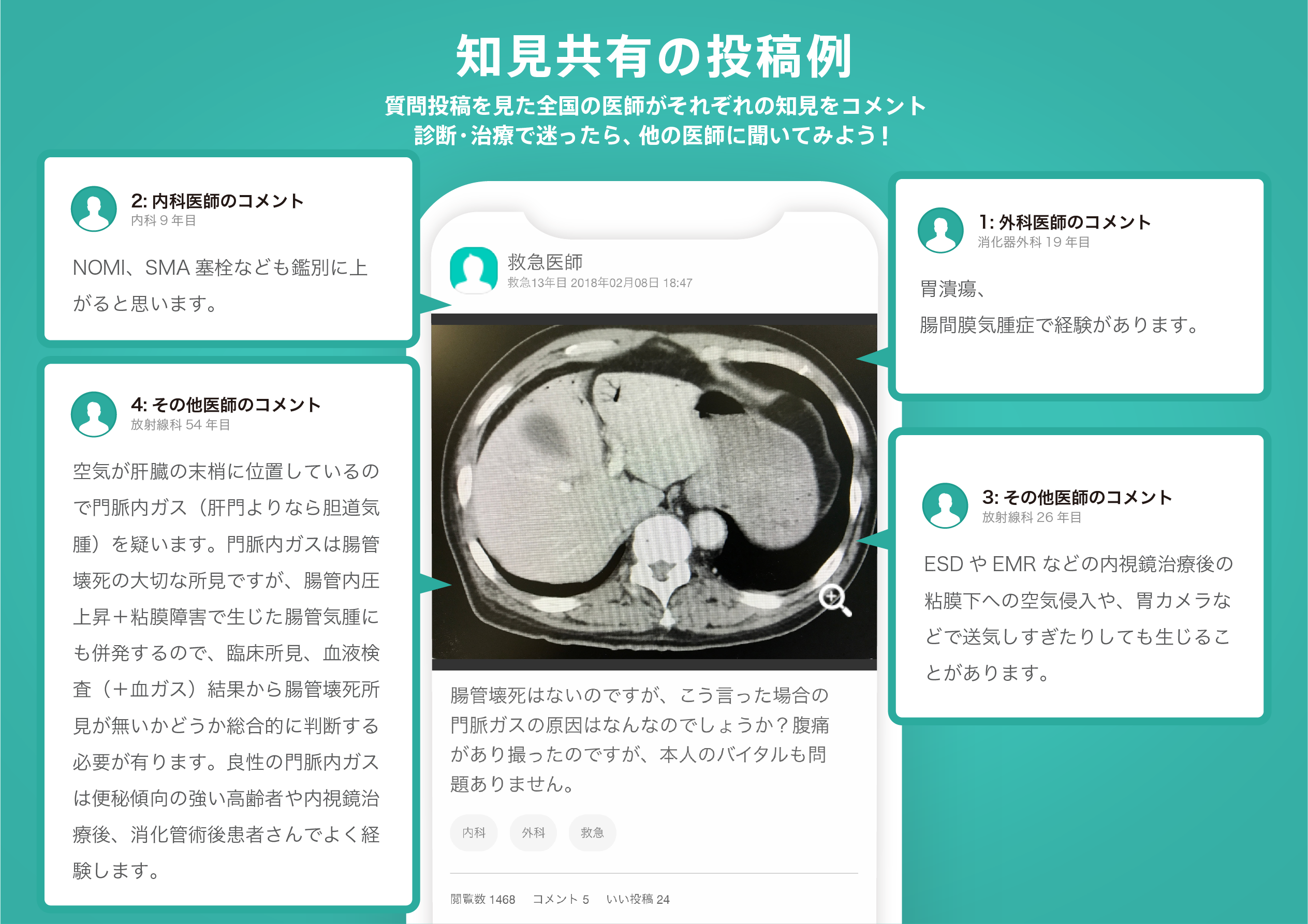
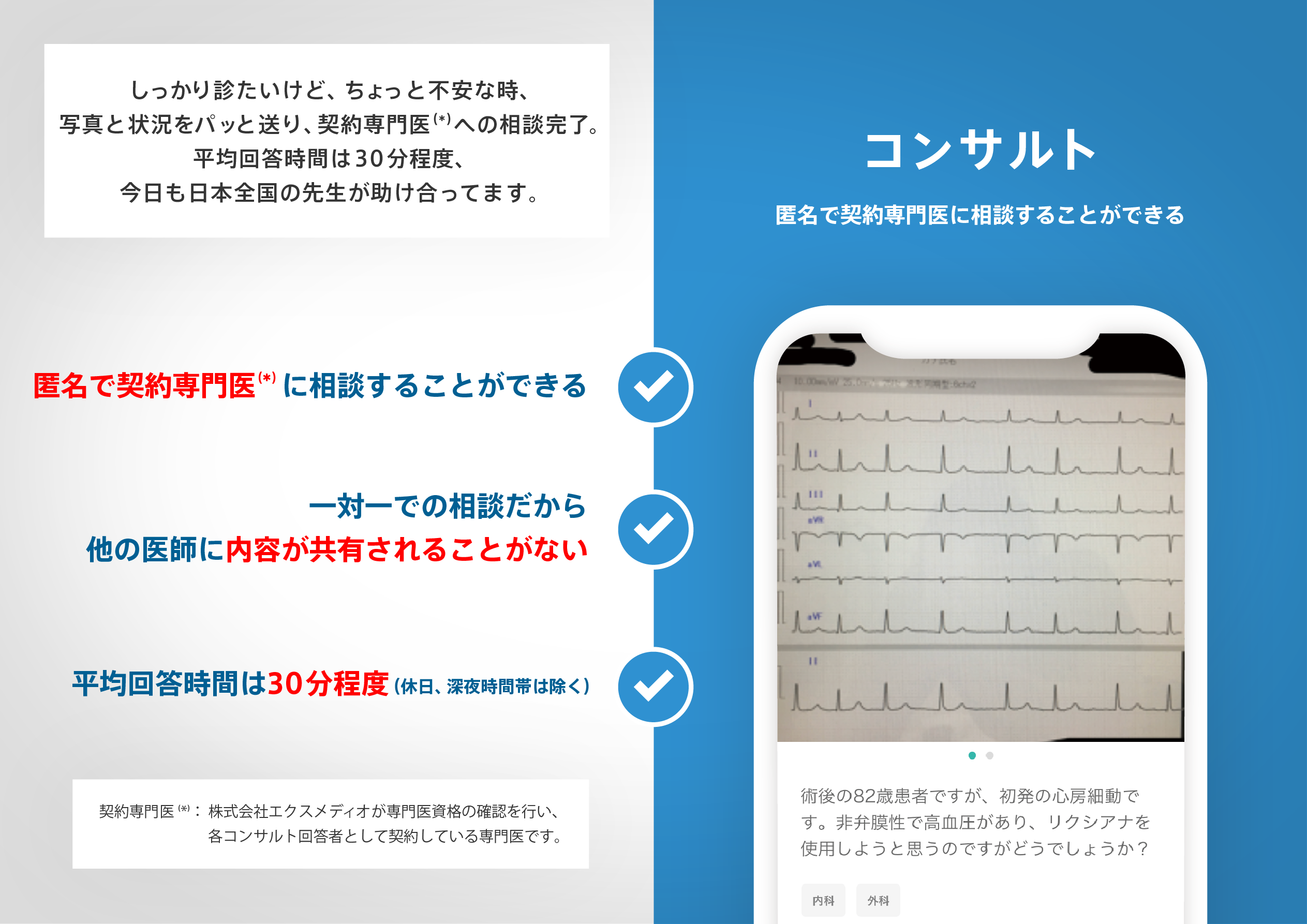
医師のための臨床サポートサービス
ヒポクラ x マイナビのご紹介
無料会員登録していただくと、さらに便利で効率的な検索が可能になります。