著名医師による解説が無料で読めます
すると翻訳の精度が向上します
逆温度の関数としての最小電子スピンまたは常磁性共鳴(ESRおよびEPR)の幅を示す最近の実験に触発され、キャリア遷移の組み合わせモデルと角周波数の静的分散を考慮することにより、運動狭窄効果を研究しました。スペクトルの不均一な広がりに。角周波数の分散は、局所フィールドの分布に起因します。不均一な静的局所フィールドの下でのサイト間の遷移は、スピンの断熱緩和を誘発します。また、スピンのオンサイトの固有の(非断熱)弛緩を検討しました。マルコフと非マルコビアの両方の遷移プロセスの2つのサイト間の遷移を明示的に考慮することにより、スピン相関関数の正確な解を取得しました。吸収線の形状は、ガウス関数とローレンツ関数の畳み込みであるVoigt関数の観点から表されます。VoIGT関数の既知の特性を使用して、半幅の全幅の変化とライン形状の変化との相関関係について説明します。どちらも運動狭窄によって誘導されます。ホッピング遷移とオンサイトの固有の緩和の両方の熱活性化プロセスを仮定することにより、幅の最小値が有機材料で実験的に観察されるように逆温度の関数として現れることを示します。一般的な信念に反して、局所的なランダムフィールドの下でガウス線の形状の狭窄が、ホッピング遷移の待機時間分布における重い尾の特性の存在下で特にローレンツの線形に必ずしもつながっていないことを示しています。
逆温度の関数としての最小電子スピンまたは常磁性共鳴(ESRおよびEPR)の幅を示す最近の実験に触発され、キャリア遷移の組み合わせモデルと角周波数の静的分散を考慮することにより、運動狭窄効果を研究しました。スペクトルの不均一な広がりに。角周波数の分散は、局所フィールドの分布に起因します。不均一な静的局所フィールドの下でのサイト間の遷移は、スピンの断熱緩和を誘発します。また、スピンのオンサイトの固有の(非断熱)弛緩を検討しました。マルコフと非マルコビアの両方の遷移プロセスの2つのサイト間の遷移を明示的に考慮することにより、スピン相関関数の正確な解を取得しました。吸収線の形状は、ガウス関数とローレンツ関数の畳み込みであるVoigt関数の観点から表されます。VoIGT関数の既知の特性を使用して、半幅の全幅の変化とライン形状の変化との相関関係について説明します。どちらも運動狭窄によって誘導されます。ホッピング遷移とオンサイトの固有の緩和の両方の熱活性化プロセスを仮定することにより、幅の最小値が有機材料で実験的に観察されるように逆温度の関数として現れることを示します。一般的な信念に反して、局所的なランダムフィールドの下でガウス線の形状の狭窄が、ホッピング遷移の待機時間分布における重い尾の特性の存在下で特にローレンツの線形に必ずしもつながっていないことを示しています。
Inspired by recent experiments showing a minimum of electron spin or paramagnetic resonance (ESR and EPR) line width as a function of inverse temperature, we studied the motional narrowing effect by considering a combined model of carrier transitions and static dispersion of the angular frequency giving rise to an inhomogeneous broadening in the spectrum. The dispersion of the angular frequency results from the distribution of the local field. The transition between the sites under inhomogeneous static local field induces adiabatic relaxation of the spin. We also considered the on-site inherent (nonadiabatic) relaxation of the spin. We obtained the exact solution of the spin correlation function by explicitly considering transitions between two sites for both Markovian and non-Markovian transition processes. The absorption line shape is expressed in terms of the Voigt function, which is a convolution of a Gaussian function and a Lorentzian function. Using the known properties of the Voigt function, we discuss the correlation between the change in the full-width at half-maximum and the change in line shape, both of which are induced by motional narrowing. By assuming thermal activation processes for both the hopping transition and the on-site inherent relaxation, we show that the minimum of the width appears as a function of inverse temperature as observed experimentally in organic materials. Contrary to the general belief, we also show that the narrowing of the Gaussian line shape under a local random field did not necessarily lead to a Lorentzian line shape in particular under the presence of heavy tail property in the waiting time distribution of hopping transitions.
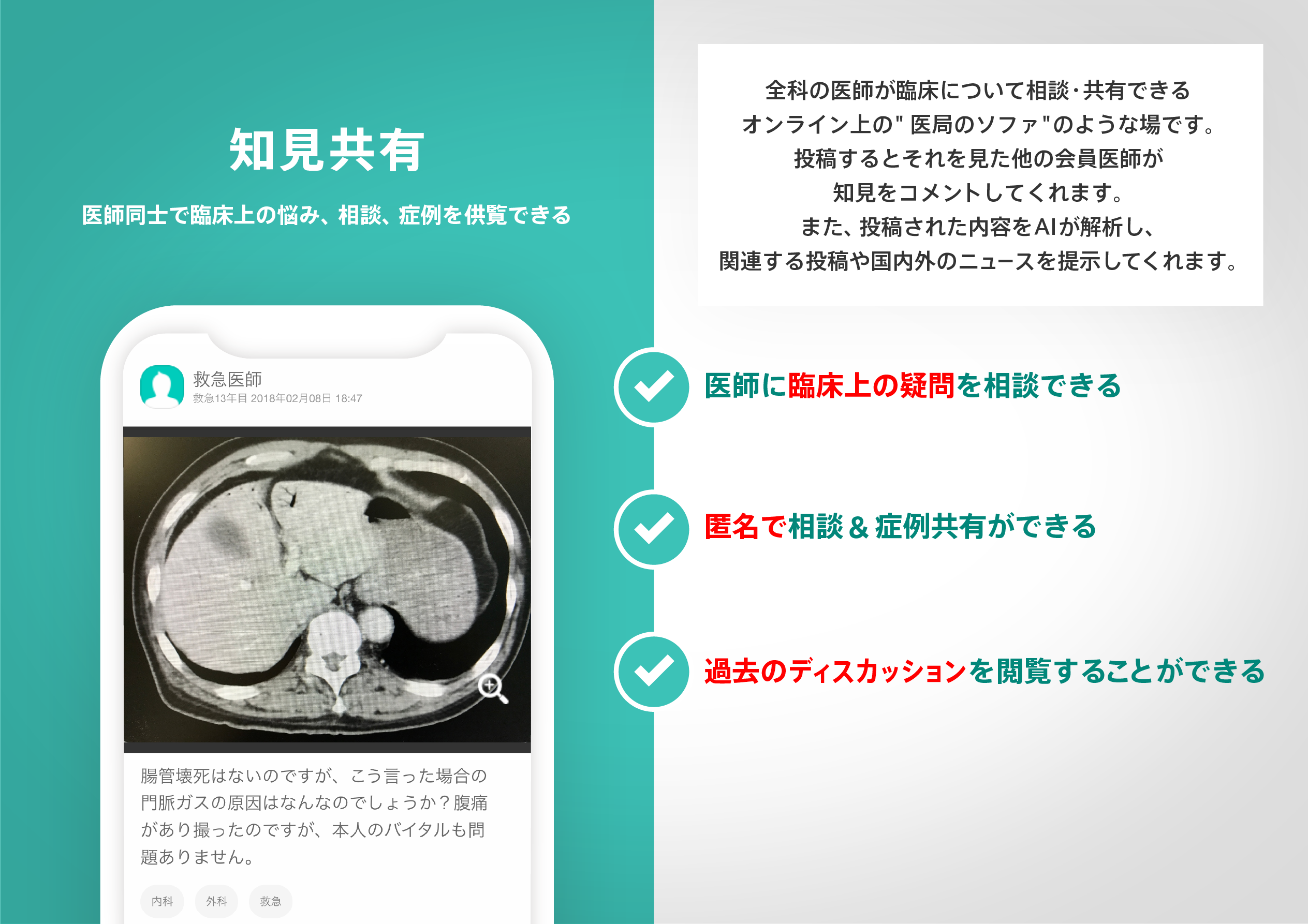
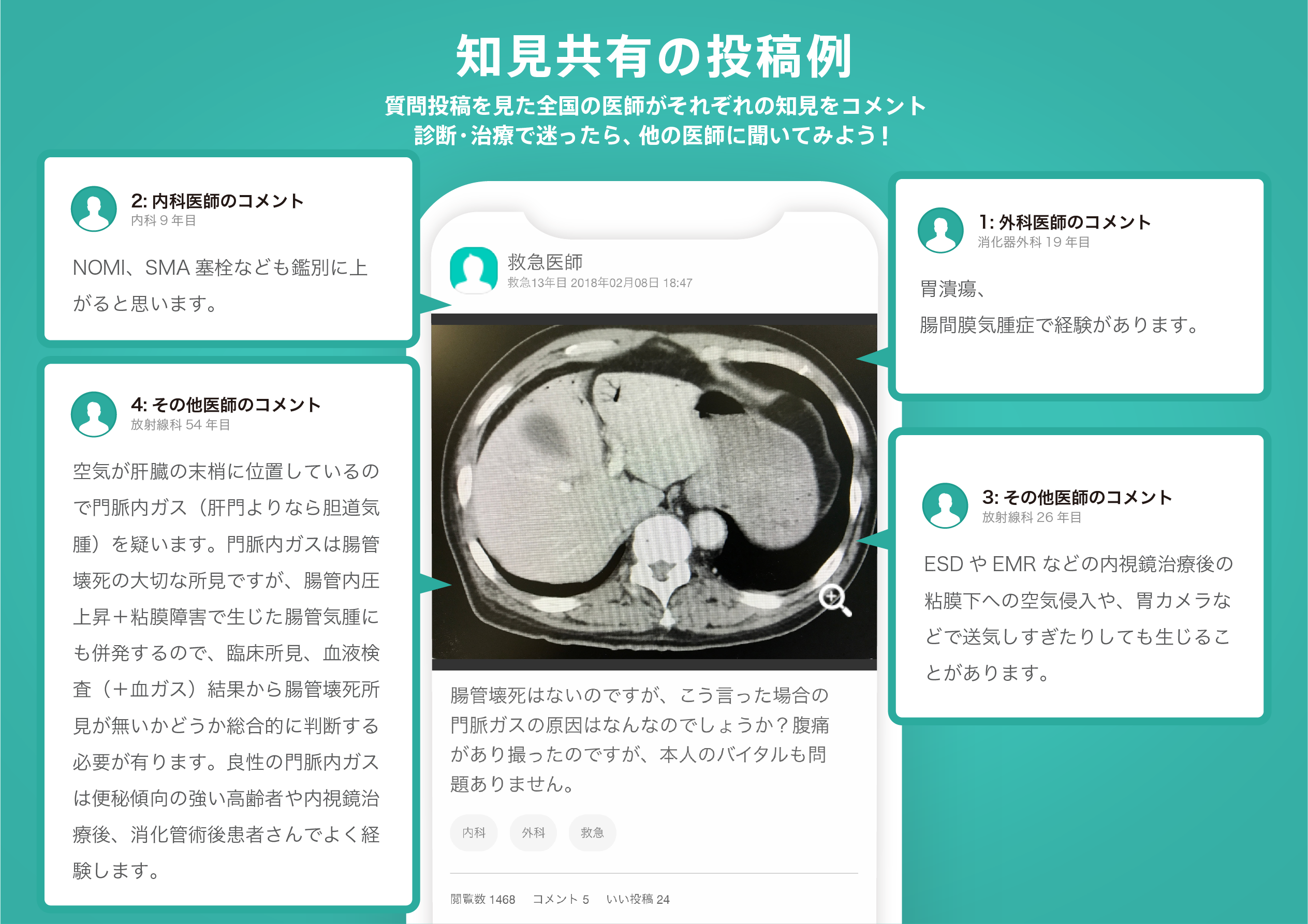
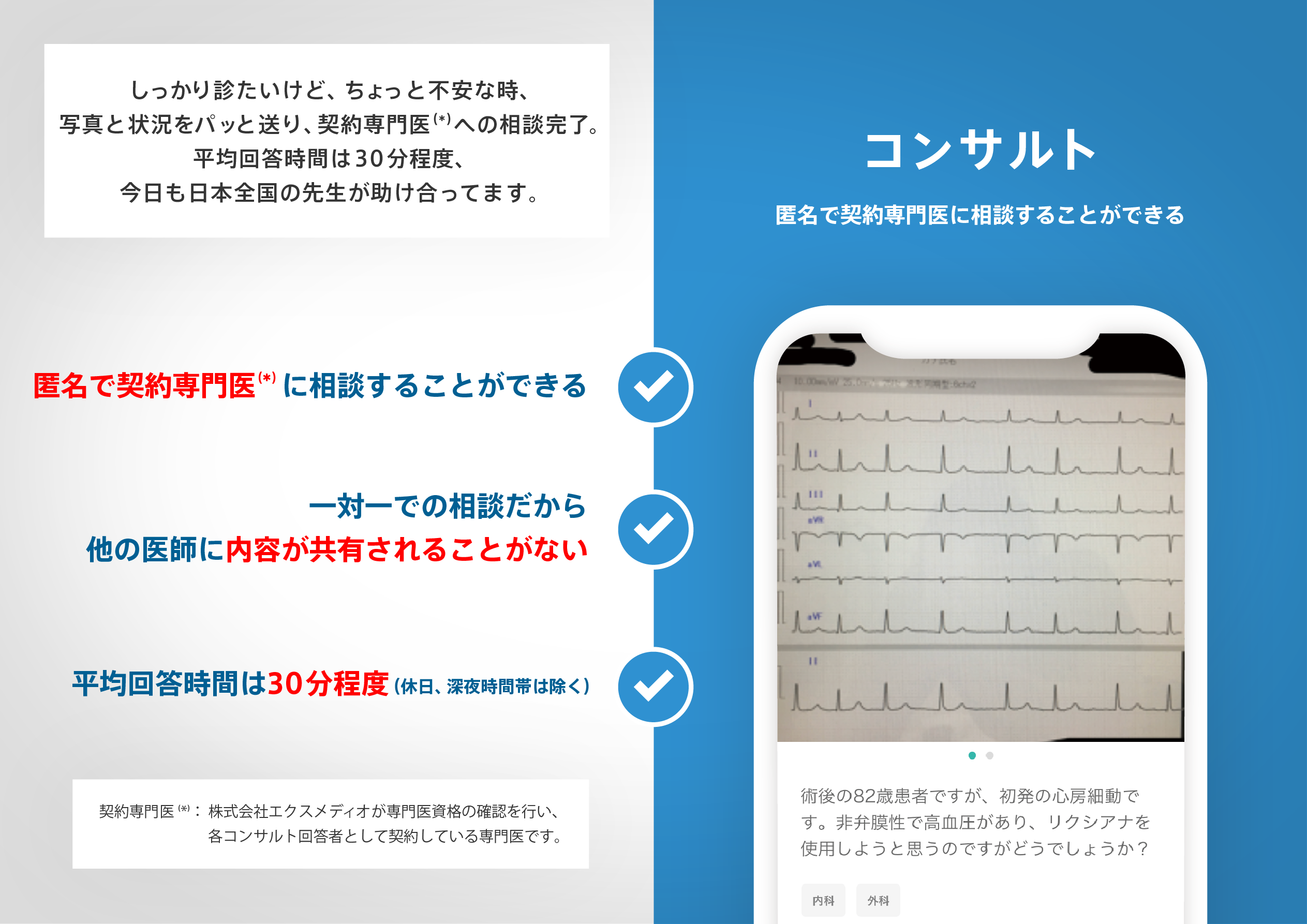
医師のための臨床サポートサービス
ヒポクラ x マイナビのご紹介
無料会員登録していただくと、さらに便利で効率的な検索が可能になります。