著名医師による解説が無料で読めます
すると翻訳の精度が向上します
生物学と医学における壊滅的な現象は、サドルノード分岐の観点から数学的に表現できるという認識が高まっています。特に、「転換」という用語、または批判的な移行という用語は、近年、生態学、医学、公衆衛生に関する一般大衆の言説に入っています。ThomとZeemanが提示したサドルノードの分岐とそれに関連する大惨事の理論は、分子生物物理学、中鏡物理学、気候科学を含む幅広い分野で応用を見てきました。この論文では、非自動動的システムの現代理論による、時間依存パラメーターP(τ)とその対応する「動的」(時間依存)サドルノード分岐を使用して、非自律システムの単純なモデルを調査します。。転倒しているシステムの実際のリターンの実際のポイントは、時間に依存しないパラメーターp a = p(τ ^)を使用して対応する自律システムが分岐を受ける破損時間τ ^と比較して大幅に遅れることができることを示します。無次元パラメーターα=λp0 3 v -2が導入されます。λは、パラメーターp(τ)に従って自律的なサドルノード分岐の曲率です。V.破損時間τ ^は常に戻りのないτ∗の実際のポイントよりも低いことがわかります。その後、臨界遷移が不可逆的であることがわかります。具体的には、関係τ *-τ ^≃2.338(λV)-1 3が分析的に取得されます。小さなλVを備えたシステムの場合、環境の急速な逆転が大惨事からシステムを救うことができるかなりの機会(τ ^、τ∗)が存在します。
生物学と医学における壊滅的な現象は、サドルノード分岐の観点から数学的に表現できるという認識が高まっています。特に、「転換」という用語、または批判的な移行という用語は、近年、生態学、医学、公衆衛生に関する一般大衆の言説に入っています。ThomとZeemanが提示したサドルノードの分岐とそれに関連する大惨事の理論は、分子生物物理学、中鏡物理学、気候科学を含む幅広い分野で応用を見てきました。この論文では、非自動動的システムの現代理論による、時間依存パラメーターP(τ)とその対応する「動的」(時間依存)サドルノード分岐を使用して、非自律システムの単純なモデルを調査します。。転倒しているシステムの実際のリターンの実際のポイントは、時間に依存しないパラメーターp a = p(τ ^)を使用して対応する自律システムが分岐を受ける破損時間τ ^と比較して大幅に遅れることができることを示します。無次元パラメーターα=λp0 3 v -2が導入されます。λは、パラメーターp(τ)に従って自律的なサドルノード分岐の曲率です。V.破損時間τ ^は常に戻りのないτ∗の実際のポイントよりも低いことがわかります。その後、臨界遷移が不可逆的であることがわかります。具体的には、関係τ *-τ ^≃2.338(λV)-1 3が分析的に取得されます。小さなλVを備えたシステムの場合、環境の急速な逆転が大惨事からシステムを救うことができるかなりの機会(τ ^、τ∗)が存在します。
There is a growing awareness that catastrophic phenomena in biology and medicine can be mathematically represented in terms of saddle-node bifurcations. In particular, the term "tipping", or critical transition has in recent years entered the discourse of the general public in relation to ecology, medicine, and public health. The saddle-node bifurcation and its associated theory of catastrophe as put forth by Thom and Zeeman has seen applications in a wide range of fields including molecular biophysics, mesoscopic physics, and climate science. In this paper, we investigate a simple model of a non-autonomous system with a time-dependent parameter p(τ) and its corresponding "dynamic" (time-dependent) saddle-node bifurcation by the modern theory of non-autonomous dynamical systems. We show that the actual point of no return for a system undergoing tipping can be significantly delayed in comparison to the breaking time τ ^ at which the corresponding autonomous system with a time-independent parameter p a = p ( τ ^ ) undergoes a bifurcation. A dimensionless parameter α = λ p 0 3 V - 2 is introduced, in which λ is the curvature of the autonomous saddle-node bifurcation according to parameter p(τ), which has an initial value of p 0 and a constant rate of change V. We find that the breaking time τ ^ is always less than the actual point of no return τ ∗ after which the critical transition is irreversible; specifically, the relation τ * - τ ^ ≃ 2.338 ( λ V ) - 1 3 is analytically obtained. For a system with a small λV, there exists a significant window of opportunity ( τ ^ , τ ∗) during which rapid reversal of the environment can save the system from catastrophe.
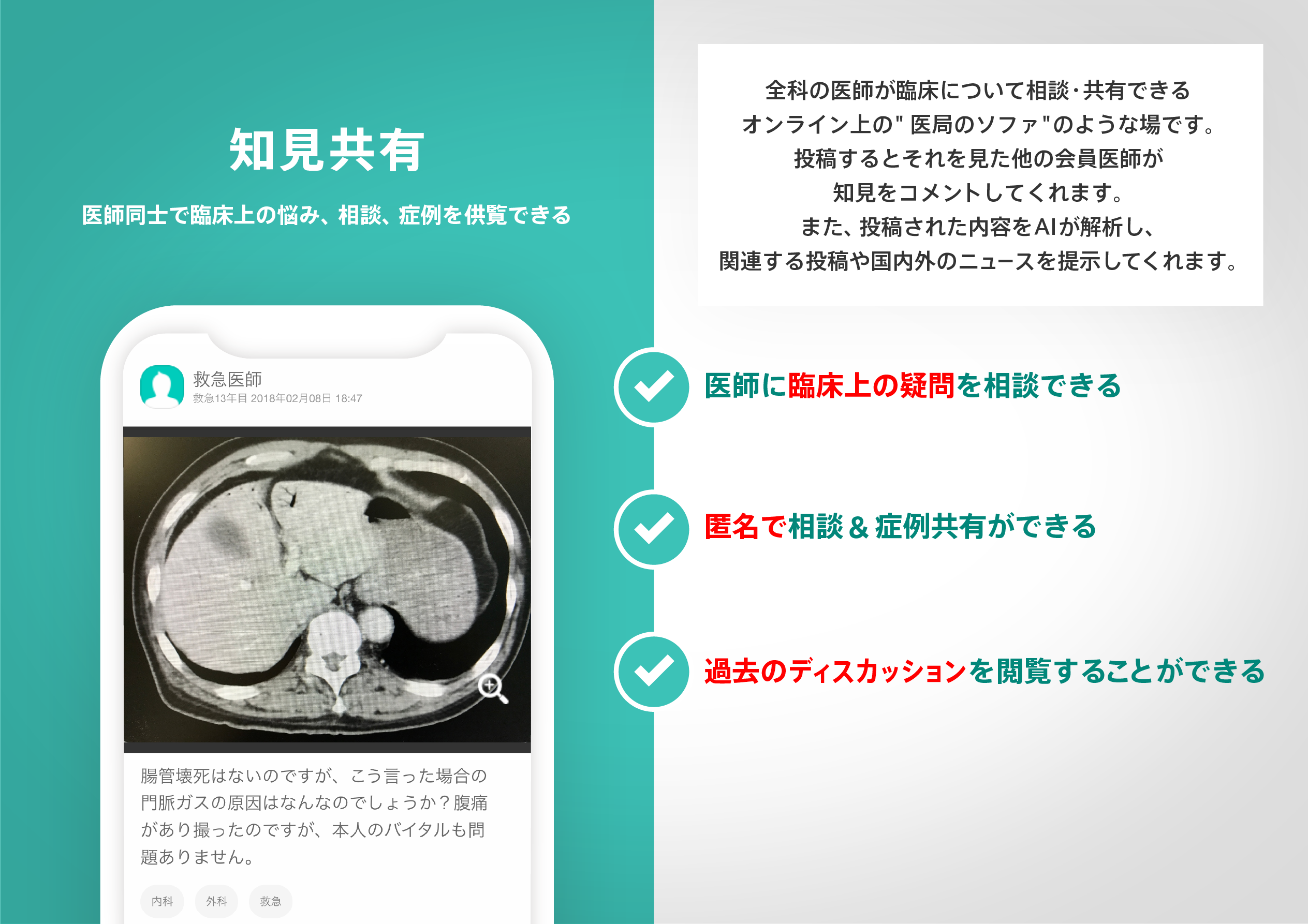
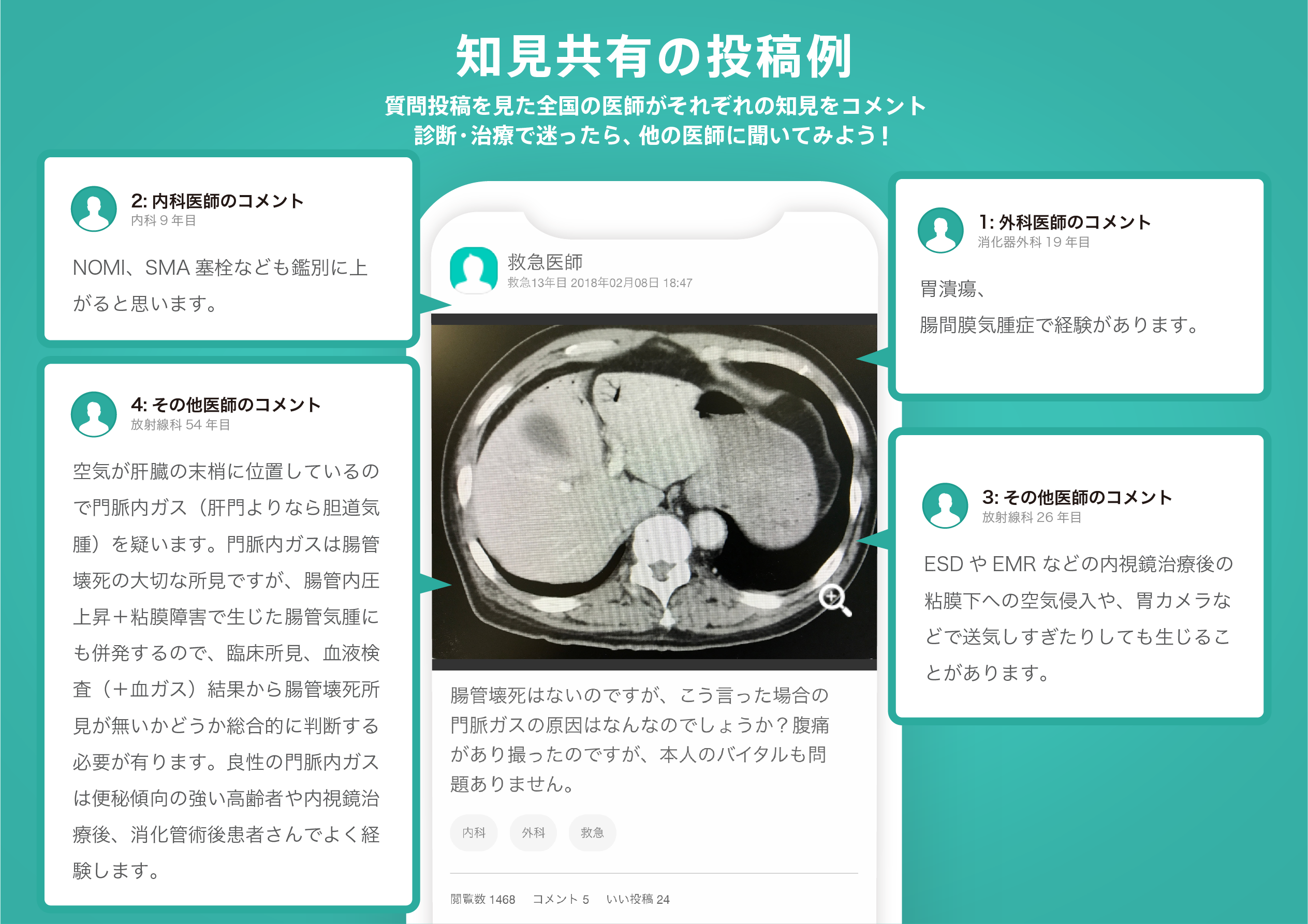
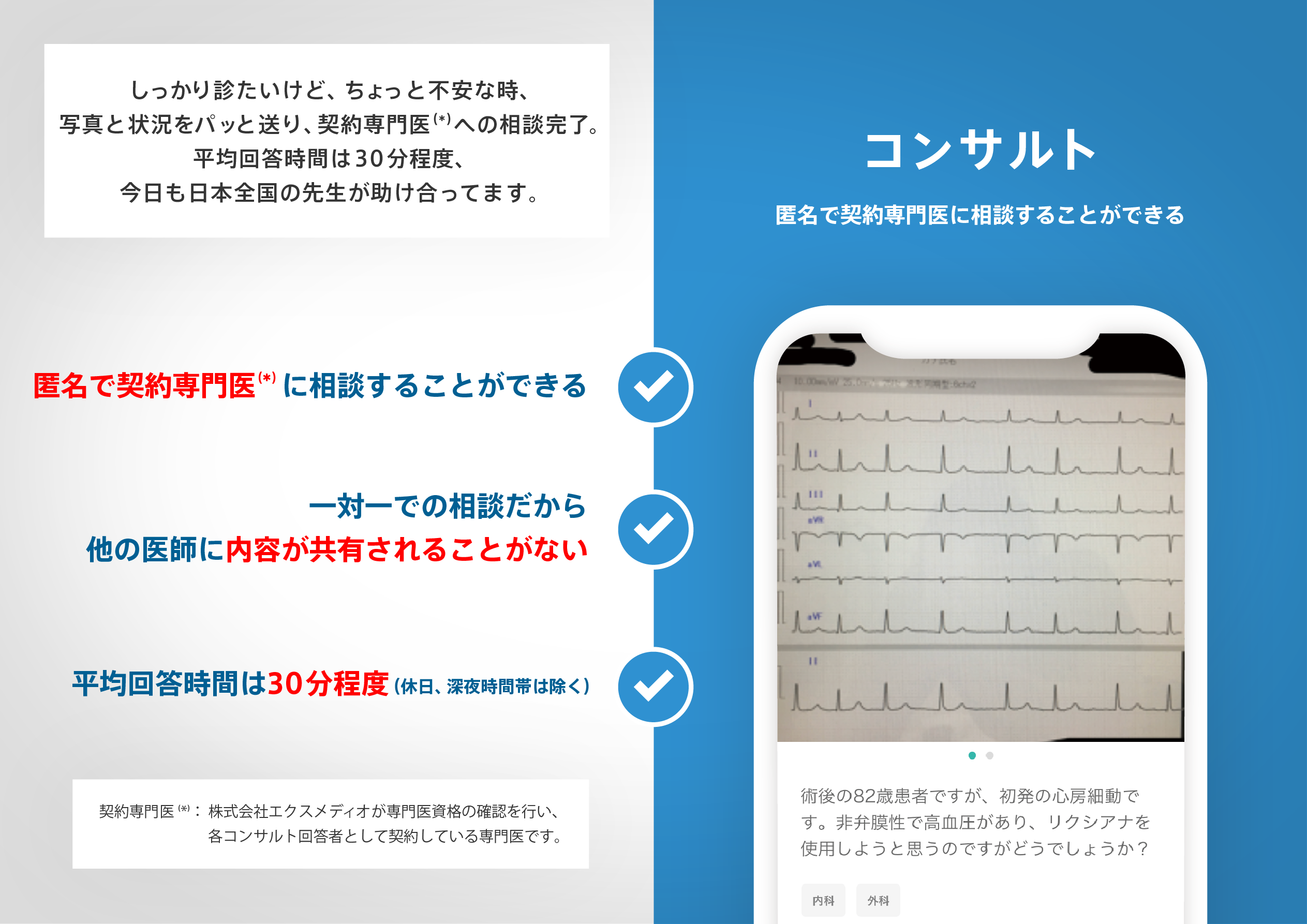
医師のための臨床サポートサービス
ヒポクラ x マイナビのご紹介
無料会員登録していただくと、さらに便利で効率的な検索が可能になります。