著名医師による解説が無料で読めます
すると翻訳の精度が向上します
任意のイオン移動度とバリンスの振動偏光下での平行電極間の1次元ポアソン - ナネストプランク(PNP)方程式に対する摂動ソリューションを導き出します。適用された電位を摂動パラメーターとして処理すると、2次溶液が電極から遠く離れたゼロの時間平均電界を生成することを示し、数値溶液を介して非対称整流電界(AREF)の最近の発見を裏付けています。非線形PNP方程式[Hashemi Amrei et al。、Phys。Rev. Lett。、2018、121、185504]。重要なことに、1次ソリューションは分析的であり、2次AREFは半分析的であり、単一の線形の通常の微分方程式を数値的に解くことによって得られ、PNP方程式に対する完全な数値解の必要性を除外することによって得られます。十分に高い周波数と電極間隔で、半分析AREFが、AREFの複雑な形状と大きさの両方を正確にキャプチャすることを実証します。
任意のイオン移動度とバリンスの振動偏光下での平行電極間の1次元ポアソン - ナネストプランク(PNP)方程式に対する摂動ソリューションを導き出します。適用された電位を摂動パラメーターとして処理すると、2次溶液が電極から遠く離れたゼロの時間平均電界を生成することを示し、数値溶液を介して非対称整流電界(AREF)の最近の発見を裏付けています。非線形PNP方程式[Hashemi Amrei et al。、Phys。Rev. Lett。、2018、121、185504]。重要なことに、1次ソリューションは分析的であり、2次AREFは半分析的であり、単一の線形の通常の微分方程式を数値的に解くことによって得られ、PNP方程式に対する完全な数値解の必要性を除外することによって得られます。十分に高い周波数と電極間隔で、半分析AREFが、AREFの複雑な形状と大きさの両方を正確にキャプチャすることを実証します。
We derive a perturbation solution to the one-dimensional Poisson-Nernst-Planck (PNP) equations between parallel electrodes under oscillatory polarization for arbitrary ionic mobilities and valences. Treating the applied potential as the perturbation parameter, we show that the second-order solution yields a nonzero time-average electric field at large distances from the electrodes, corroborating the recent discovery of Asymmetric Rectified Electric Fields (AREFs) via numerical solution to the full nonlinear PNP equations [Hashemi Amrei et al., Phys. Rev. Lett., 2018, 121, 185504]. Importantly, the first-order solution is analytic, while the second-order AREF is semi-analytic and obtained by numerically solving a single linear ordinary differential equation, obviating the need for full numerical solutions to the PNP equations. We demonstrate that at sufficiently high frequencies and electrode spacings the semi-analytical AREF accurately captures both the complicated shape and the magnitude of the AREF, even at large applied potentials.
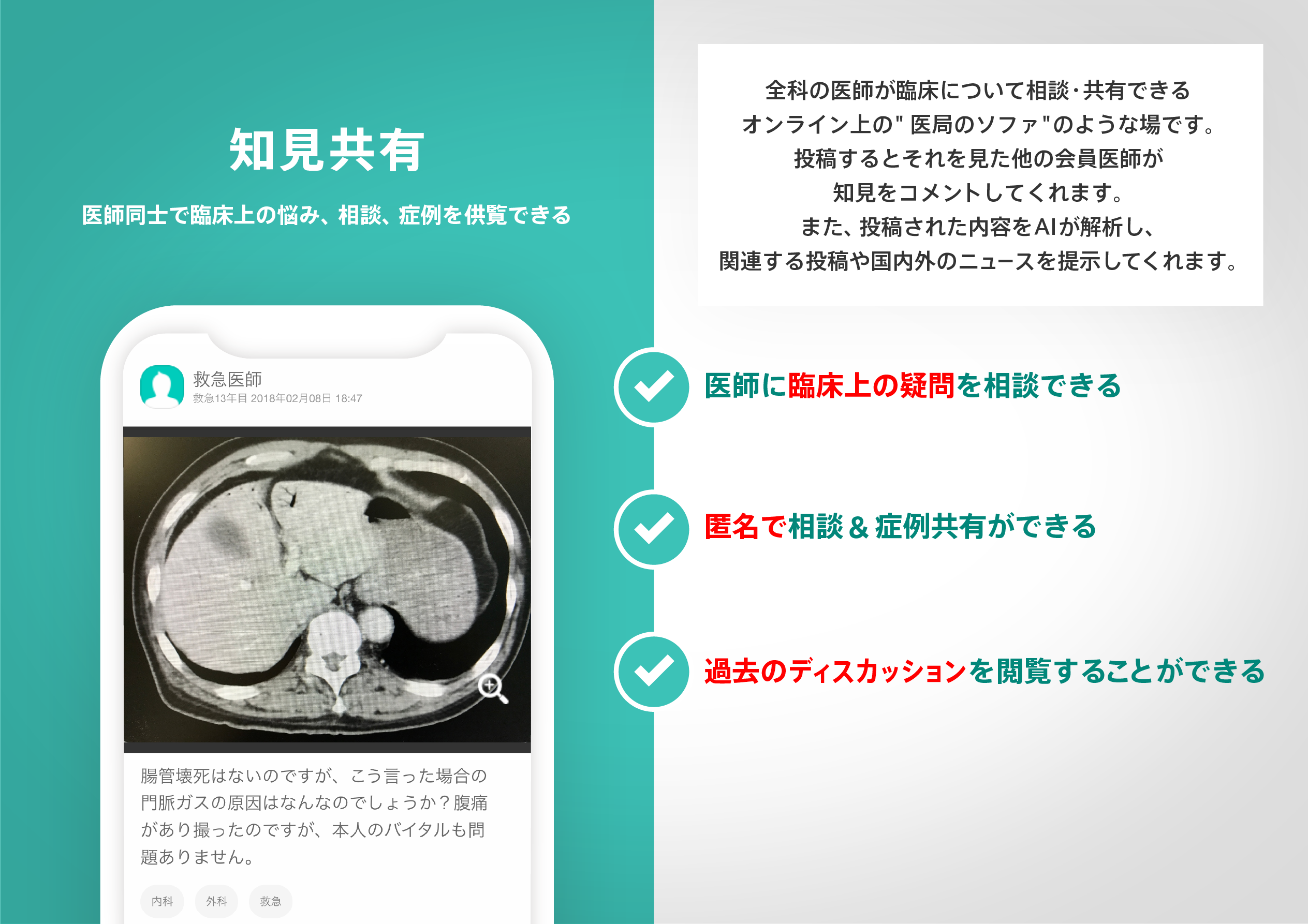
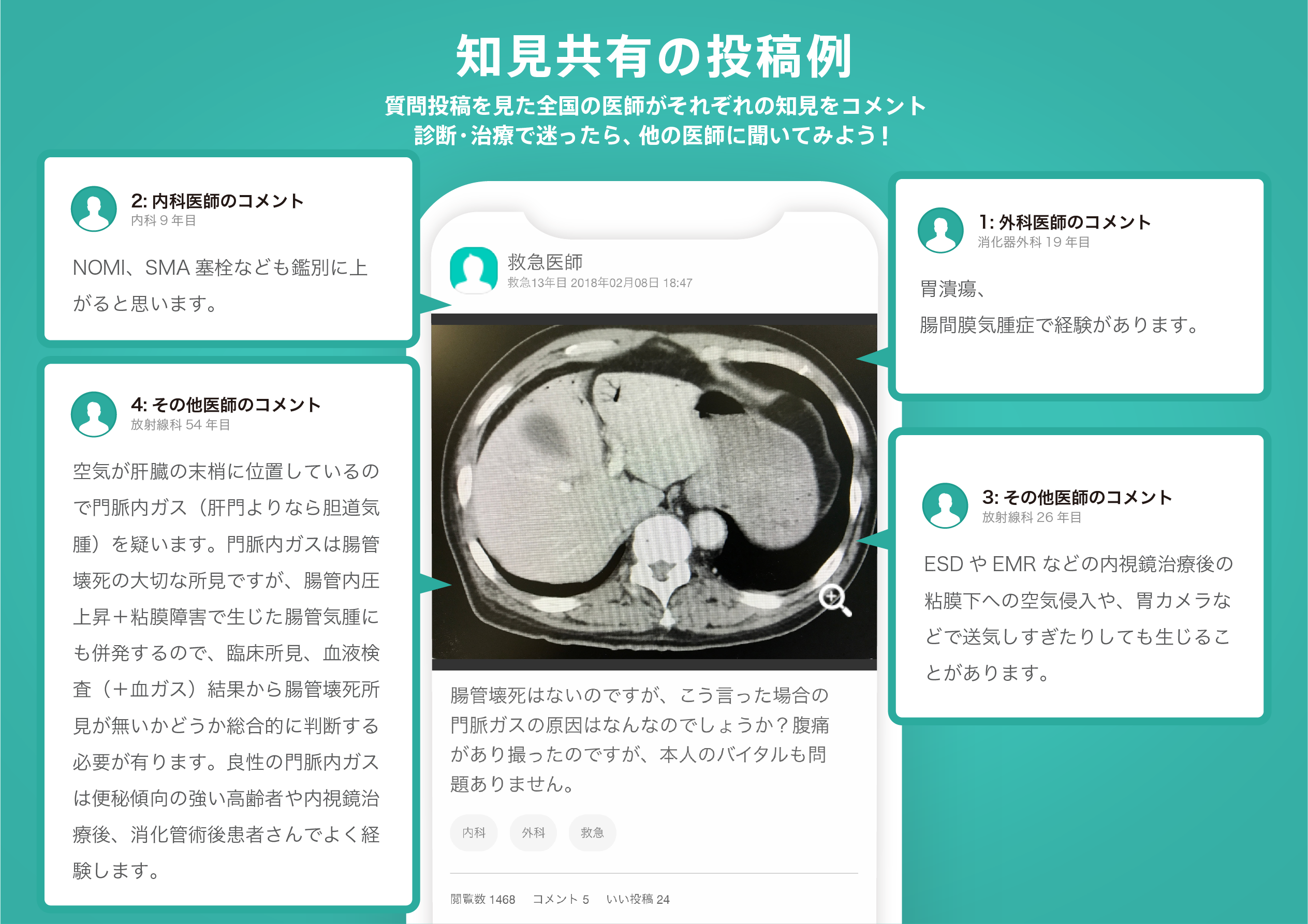
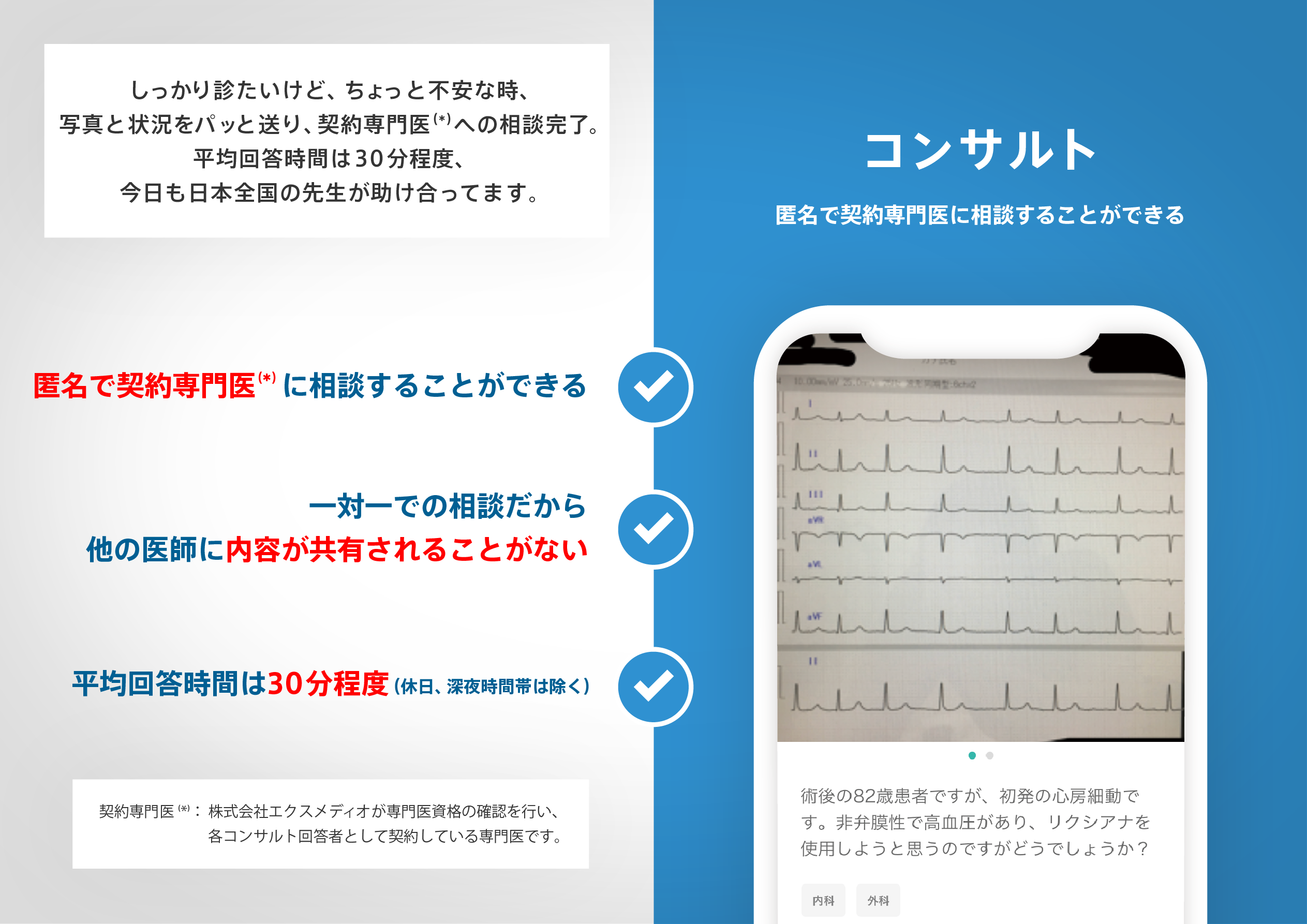
医師のための臨床サポートサービス
ヒポクラ x マイナビのご紹介
無料会員登録していただくと、さらに便利で効率的な検索が可能になります。