著名医師による解説が無料で読めます
すると翻訳の精度が向上します
この作業では、密度マトリックス復体化グループ(DMRG)アルゴリズムによるマトリックス産物状態の最適化手順におけるトランス相関電子ハミルトニアンの最初の実装を提示します。トランス相関であるアンサッツでは、電子ハミルトニアンは、電子電子合体での波動関数の尖を記述するために、jastrow因子と類似しています。その結果、波動関数は、1粒子基底関数とスレーター決定要因の観点から、従来の拡張により正確に近似する方が簡単です。最初の量子化におけるトランス相関ハミルトニアンは、最大3体相互作用を含みます。これは、このハミルトニアンの2番目の定量化された表現に入る2倍および3体積分に堅牢な密度密度フィッティングを適用することで標準的な方法で対処します。トランス相関ハミルトニアンのherの不足は、トランス相関DMRGの最初の研究の線に沿って世話をされます[J. Chem。Phys。2020、153、164115]マトリックス製品演算子としてエンコードし、想像上の時間依存DMRGで対応する基底状態波関数を最適化することにより。いくつかの原子と第一列式二原子分子の例で、量子化学相関DMRGアプローチを示します。伝統相関が、従来のDMRGと比較して、収束率を完全な基底セット制限に改善することを示します。さらに、私たちは、トランス相関ハミルトニアンのマトリックス製品オペレーターの表現を処理するコストを削減することを目的としたアプローチの拡張を研究します。
この作業では、密度マトリックス復体化グループ(DMRG)アルゴリズムによるマトリックス産物状態の最適化手順におけるトランス相関電子ハミルトニアンの最初の実装を提示します。トランス相関であるアンサッツでは、電子ハミルトニアンは、電子電子合体での波動関数の尖を記述するために、jastrow因子と類似しています。その結果、波動関数は、1粒子基底関数とスレーター決定要因の観点から、従来の拡張により正確に近似する方が簡単です。最初の量子化におけるトランス相関ハミルトニアンは、最大3体相互作用を含みます。これは、このハミルトニアンの2番目の定量化された表現に入る2倍および3体積分に堅牢な密度密度フィッティングを適用することで標準的な方法で対処します。トランス相関ハミルトニアンのherの不足は、トランス相関DMRGの最初の研究の線に沿って世話をされます[J. Chem。Phys。2020、153、164115]マトリックス製品演算子としてエンコードし、想像上の時間依存DMRGで対応する基底状態波関数を最適化することにより。いくつかの原子と第一列式二原子分子の例で、量子化学相関DMRGアプローチを示します。伝統相関が、従来のDMRGと比較して、収束率を完全な基底セット制限に改善することを示します。さらに、私たちは、トランス相関ハミルトニアンのマトリックス製品オペレーターの表現を処理するコストを削減することを目的としたアプローチの拡張を研究します。
In this work, we present the first implementation of the transcorrelated electronic Hamiltonian in an optimization procedure for matrix product states by the density matrix renormalization group (DMRG) algorithm. In the transcorrelation ansatz, the electronic Hamiltonian is similarity-transformed with a Jastrow factor to describe the cusp in the wave function at electron-electron coalescence. As a result, the wave function is easier to approximate accurately with the conventional expansion in terms of one-particle basis functions and Slater determinants. The transcorrelated Hamiltonian in first quantization comprises up to three-body interactions, which we deal with in the standard way by applying robust density fitting to two- and three-body integrals entering the second-quantized representation of this Hamiltonian. The lack of hermiticity of the transcorrelated Hamiltonian is taken care of along the lines of the first work on transcorrelated DMRG [ J. Chem. Phys. 2020, 153, 164115] by encoding it as a matrix product operator and optimizing the corresponding ground state wave function with imaginary-time time-dependent DMRG. We demonstrate our quantum chemical transcorrelated DMRG approach at the example of several atoms and first-row diatomic molecules. We show that transcorrelation improves the convergence rate to the complete basis set limit in comparison to conventional DMRG. Moreover, we study extensions of our approach that aim at reducing the cost of handling the matrix product operator representation of the transcorrelated Hamiltonian.
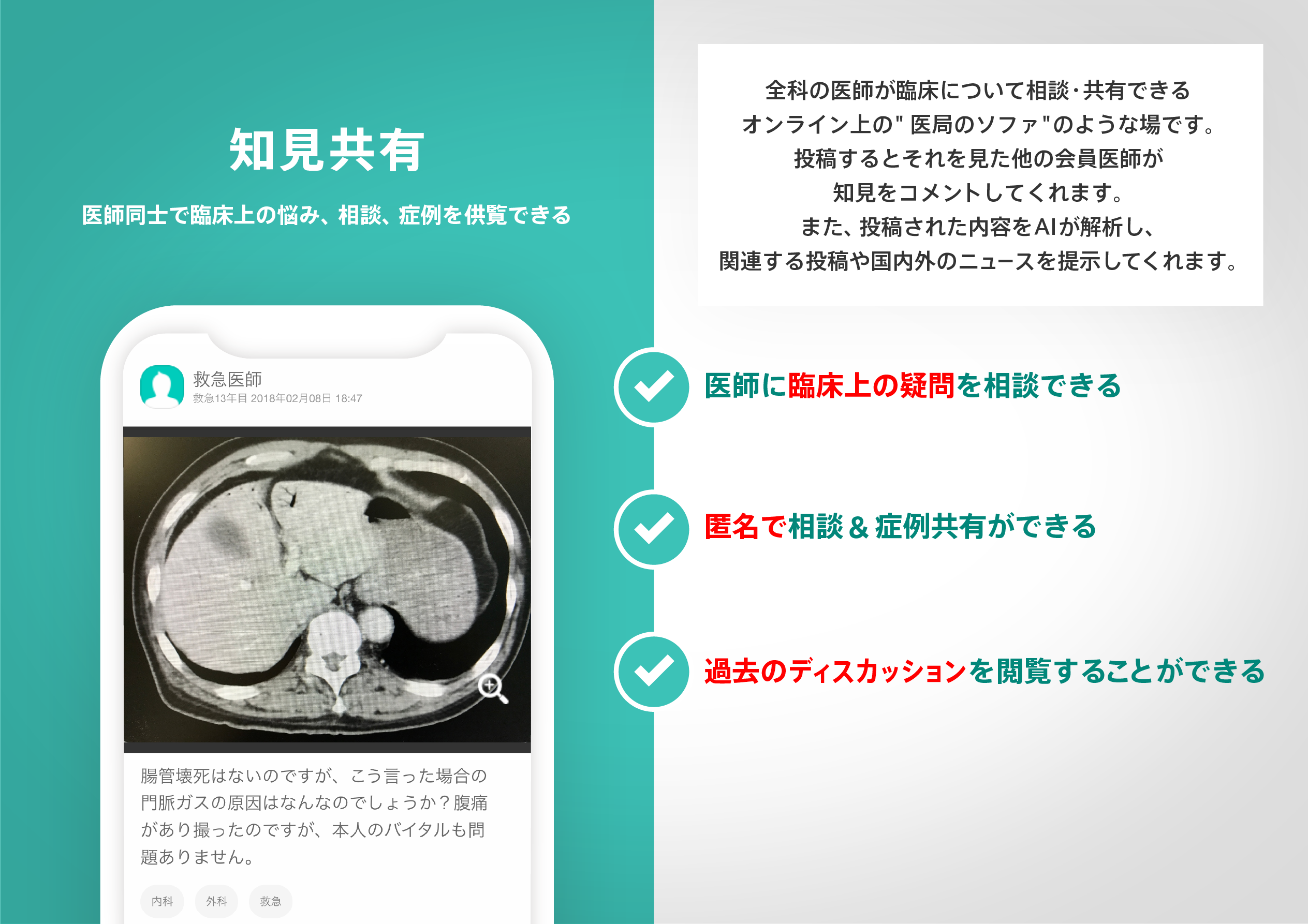
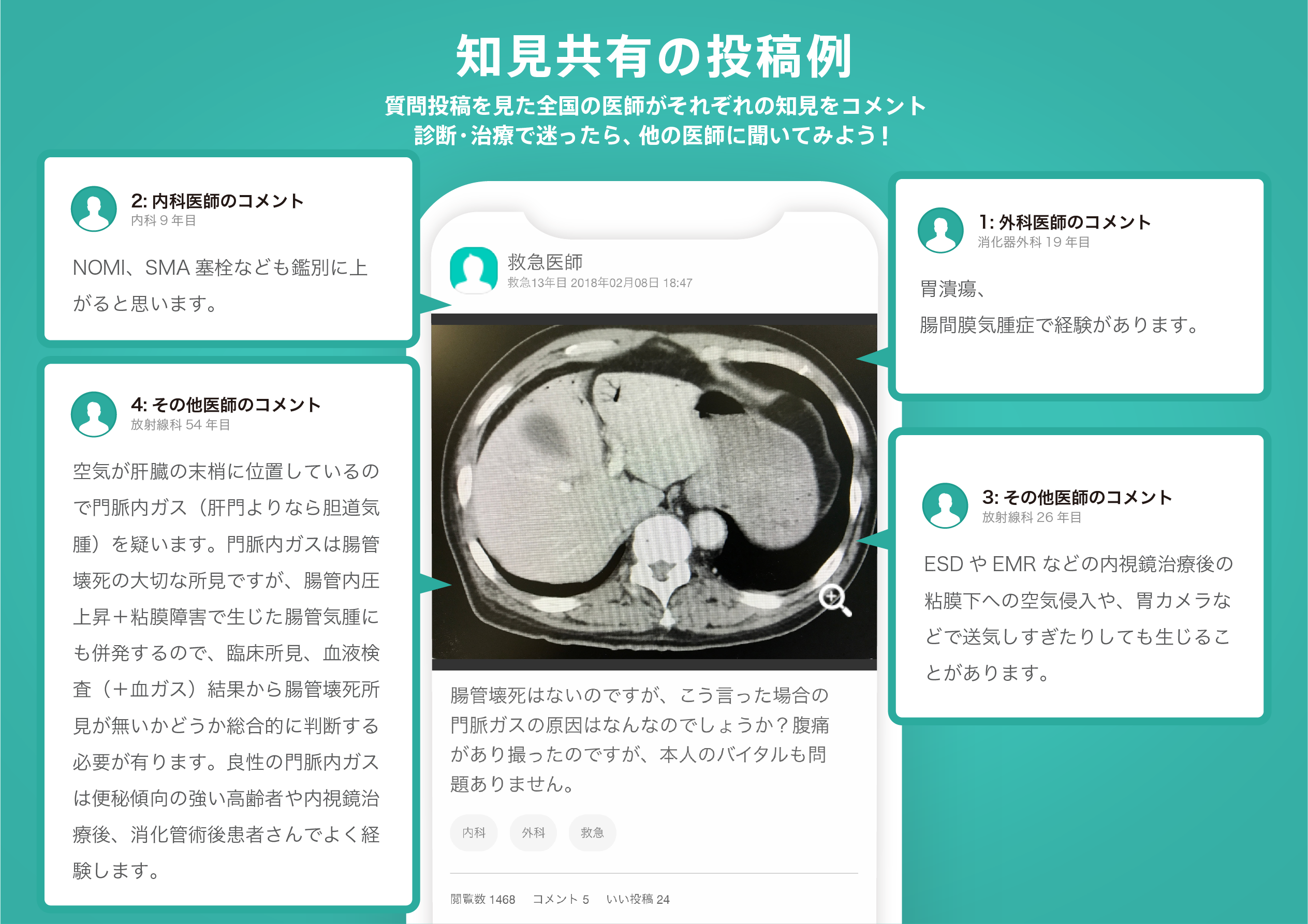
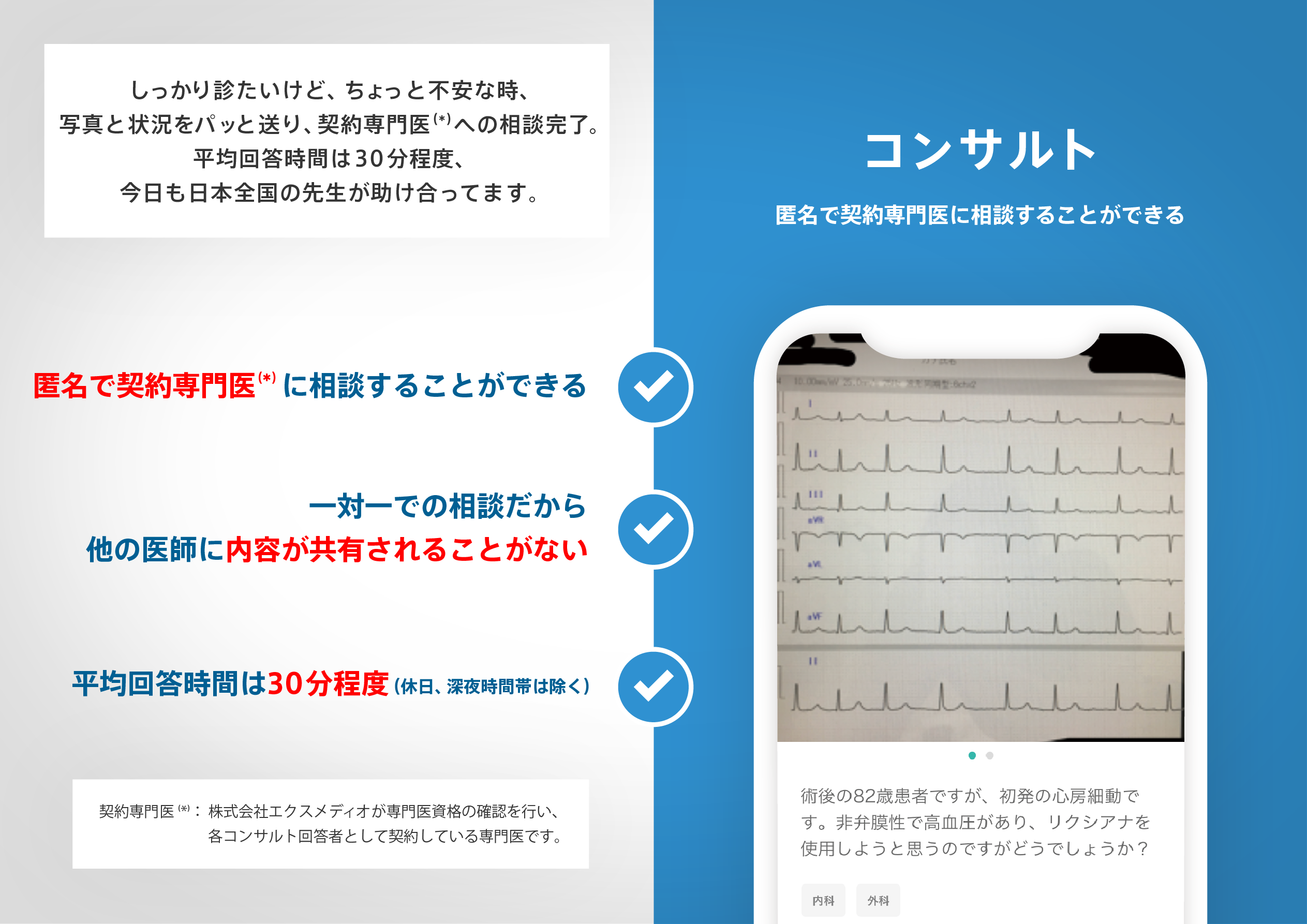
医師のための臨床サポートサービス
ヒポクラ x マイナビのご紹介
無料会員登録していただくと、さらに便利で効率的な検索が可能になります。