著名医師による解説が無料で読めます
すると翻訳の精度が向上します
ガラスと米によって提案された古典的な単原子液体の分子運動の顕微鏡理論[Phys。Rev. 176、239(1968)10.1103/Physrev.176.239]が再検討され、拡張され、平均時間依存性高調波力場における原子拡散のブラウン記述に動的摩擦を組み込みます。修正された非マルコビアンランゲビン方程式は、時間依存性摩擦係数を持つ速度自己相関関数の運動方程式を導出するために利用されます。方程式の数値溶液は、Lennard-Jones液体、液体アルカリ、および広範な密度と温度にわたる遷移金属の速度自己相関関数を優れた説明を提供します。運動方程式の導出は、摩擦係数の時間依存性の自己整合的な発現につながります。我々の結果は、摩擦係数の時間依存性の性質が液体密度と劇的に変化することを示しています。低密度と中程度の密度では、動的摩擦は指数関数的に減衰しますが、高液密度で指数関数的に増加します。私たちの調査結果は、液体中の原子動力学のブラウン説明の異なる見通しの機会を提供します。
ガラスと米によって提案された古典的な単原子液体の分子運動の顕微鏡理論[Phys。Rev. 176、239(1968)10.1103/Physrev.176.239]が再検討され、拡張され、平均時間依存性高調波力場における原子拡散のブラウン記述に動的摩擦を組み込みます。修正された非マルコビアンランゲビン方程式は、時間依存性摩擦係数を持つ速度自己相関関数の運動方程式を導出するために利用されます。方程式の数値溶液は、Lennard-Jones液体、液体アルカリ、および広範な密度と温度にわたる遷移金属の速度自己相関関数を優れた説明を提供します。運動方程式の導出は、摩擦係数の時間依存性の自己整合的な発現につながります。我々の結果は、摩擦係数の時間依存性の性質が液体密度と劇的に変化することを示しています。低密度と中程度の密度では、動的摩擦は指数関数的に減衰しますが、高液密度で指数関数的に増加します。私たちの調査結果は、液体中の原子動力学のブラウン説明の異なる見通しの機会を提供します。
A microscopic theory of molecular motion in classical monatomic liquids, proposed by Glass and Rice [Phys. Rev. 176, 239 (1968)10.1103/PhysRev.176.239], is revisited and extended to incorporate the dynamic friction in the Brownian description of the atomic diffusion in a mean-time-dependent harmonic force field. A modified, non-Markovian Langevin equation is utilized to derive an equation of motion for the velocity autocorrelation function with time-dependent friction coefficient. Numerical solution of the equation gives an excellent account of the velocity autocorrelation function in Lennard-Jones liquids, liquid alkali, and transition metals over a broad range of density and temperature. Derivation of the equation of motion leads to a self-consistent expression for the time dependence of friction coefficient. Our results demonstrate that the nature of time dependence of the friction coefficient changes dramatically with the liquid density. At low and moderate densities, the dynamic friction decays exponentially whereas it increases exponentially at high liquid densities. Our findings provide an opportunity for a different outlook of the Brownian description of atomic dynamics in liquids.
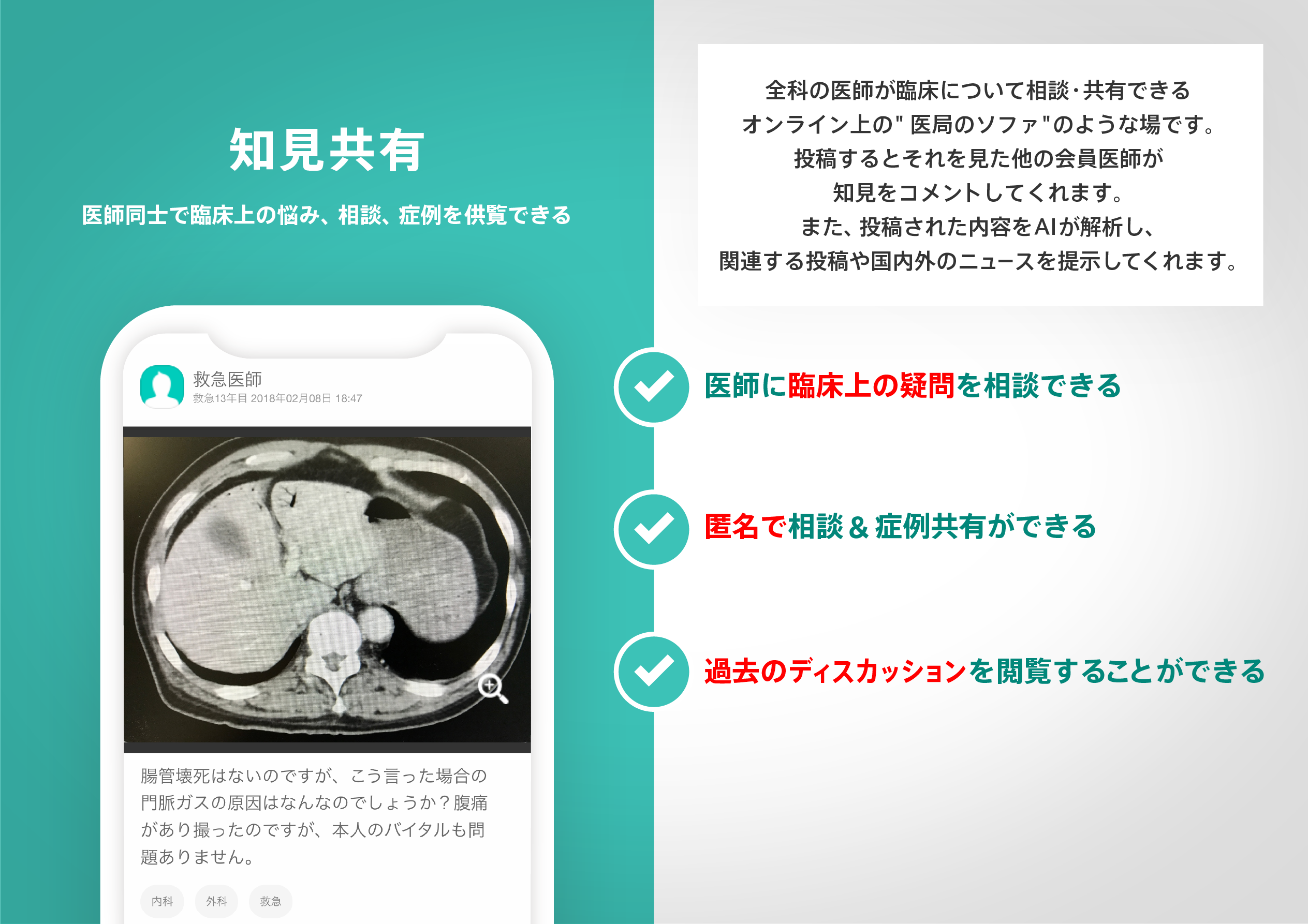
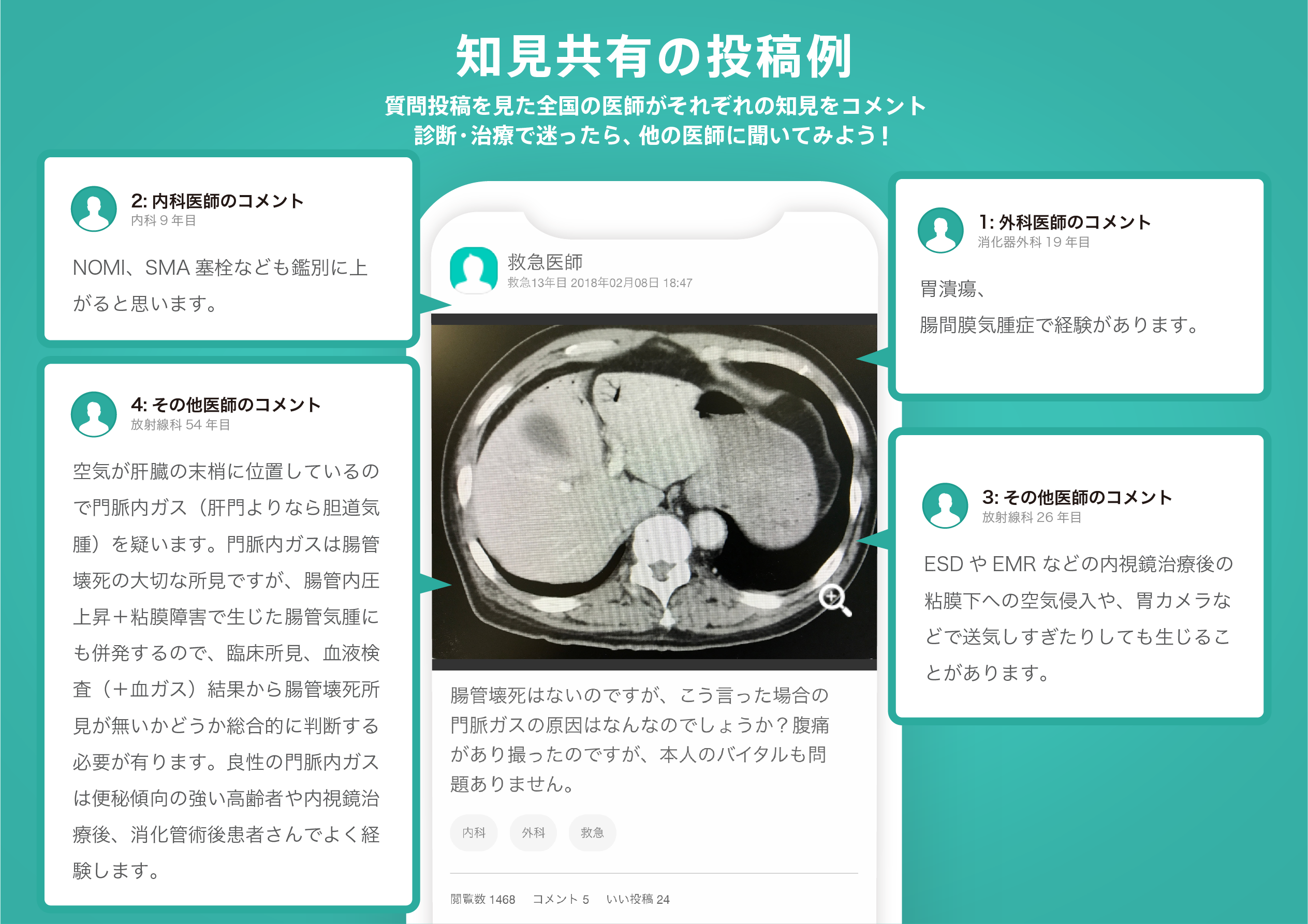
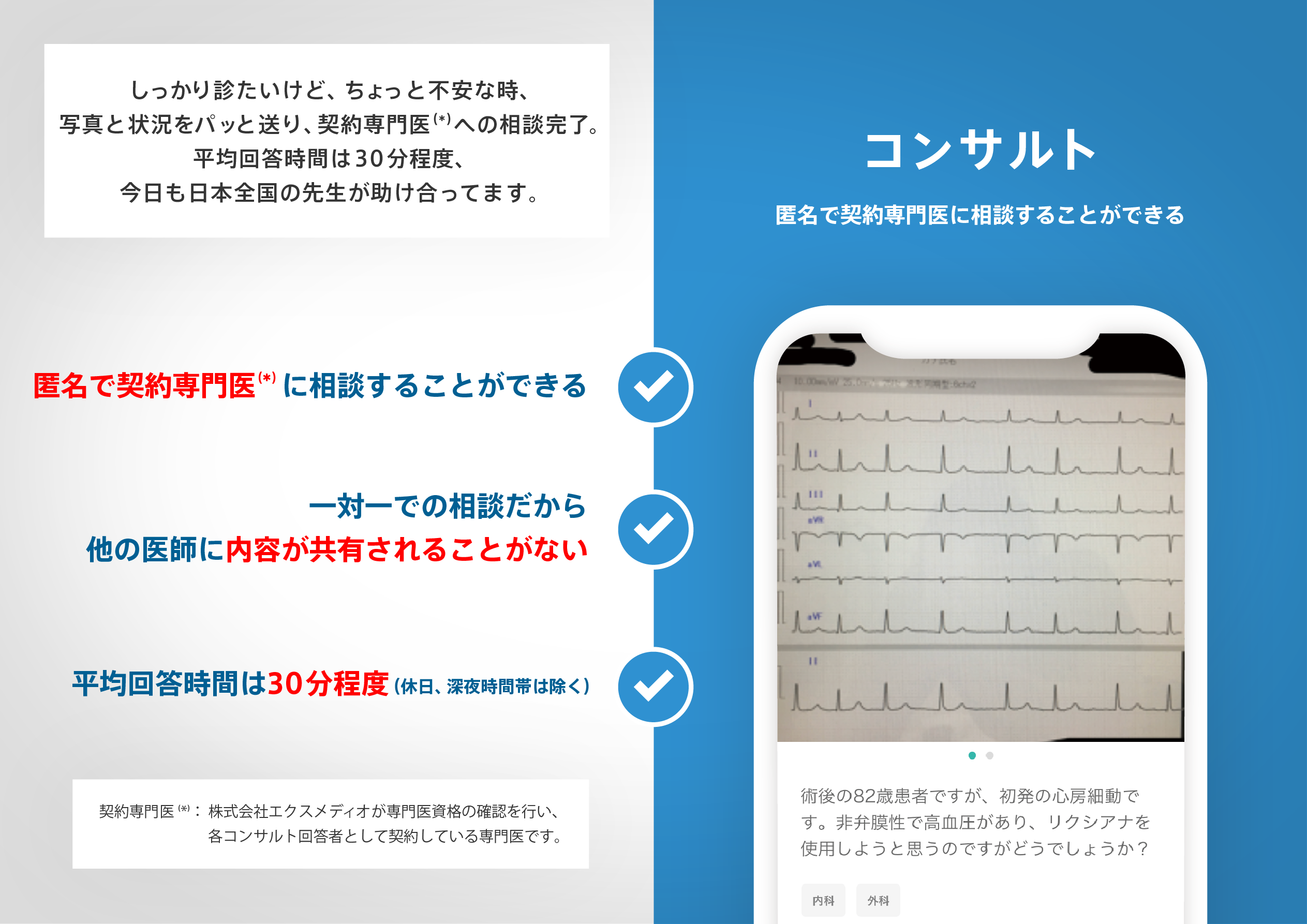
医師のための臨床サポートサービス
ヒポクラ x マイナビのご紹介
無料会員登録していただくと、さらに便利で効率的な検索が可能になります。