著名医師による解説が無料で読めます
すると翻訳の精度が向上します
このホワイトペーパーでは、レシーバー動作特性(ROC)曲線下の領域の分析方法に焦点を当てています。ROC領域の分析には、同じ症例のセットで取られた繰り返し測定の相関構造と、症例の効果的な要約から生じる治療あたりの測定の不足を診断精度のいくつかの領域測定値に組み込む必要があります。ROCデータの繰り返しの性質は、Swets and Pickett(1982、診断システムの評価:信号検出理論からの方法)によって以前に提案された分析方法で考慮されています。Delong、Delong、およびClarke-Pearson(1988、Biometrics 44、837-845)。Delong et al。の手順は、診断テストの一般的な状況のためのWALDテストに拡張されています。Jackknifeの擬似価値をデータとして扱うことによって分析する方法は、テストするエリアメジャーの数が非常に少ない場合に非常に役立ちます。多変量マルチサンプルU統計の共分散に基づくWALDテストは、繰り返し測定のための単変量分散分析(ANOVA)および3方向の要因ANOVAの2つのアプローチと比較されます。モンテカルロシミュレーションは、3つのテストが大規模なサンプルサイズの5%レベルで公称サイズに良好な近似を与えることを示していますが、データとしてROC領域を使用したペアのt検定には、他の3つのテストのパワーがあり、ハンリーとマクニールの方法は診断精度のテストには不適切です。WALD統計は、擬似価値のANOVAよりも優れています。通常と病気の分布の異なる構造が説明されている複数の削除のジャックナイフスキームは、単純な複数削除スキームよりもわずかに優れているように見えるが、かなりのパワーの違いは明らかではなく、一度に多すぎるケースの削除が犠牲になる可能性がある。これらの方法には、放射線科と一般的な医学のROC研究における診断テストにおいて重要な用途があります。
このホワイトペーパーでは、レシーバー動作特性(ROC)曲線下の領域の分析方法に焦点を当てています。ROC領域の分析には、同じ症例のセットで取られた繰り返し測定の相関構造と、症例の効果的な要約から生じる治療あたりの測定の不足を診断精度のいくつかの領域測定値に組み込む必要があります。ROCデータの繰り返しの性質は、Swets and Pickett(1982、診断システムの評価:信号検出理論からの方法)によって以前に提案された分析方法で考慮されています。Delong、Delong、およびClarke-Pearson(1988、Biometrics 44、837-845)。Delong et al。の手順は、診断テストの一般的な状況のためのWALDテストに拡張されています。Jackknifeの擬似価値をデータとして扱うことによって分析する方法は、テストするエリアメジャーの数が非常に少ない場合に非常に役立ちます。多変量マルチサンプルU統計の共分散に基づくWALDテストは、繰り返し測定のための単変量分散分析(ANOVA)および3方向の要因ANOVAの2つのアプローチと比較されます。モンテカルロシミュレーションは、3つのテストが大規模なサンプルサイズの5%レベルで公称サイズに良好な近似を与えることを示していますが、データとしてROC領域を使用したペアのt検定には、他の3つのテストのパワーがあり、ハンリーとマクニールの方法は診断精度のテストには不適切です。WALD統計は、擬似価値のANOVAよりも優れています。通常と病気の分布の異なる構造が説明されている複数の削除のジャックナイフスキームは、単純な複数削除スキームよりもわずかに優れているように見えるが、かなりのパワーの違いは明らかではなく、一度に多すぎるケースの削除が犠牲になる可能性がある。これらの方法には、放射線科と一般的な医学のROC研究における診断テストにおいて重要な用途があります。
This paper focuses on methods of analysis of areas under receiver operating characteristic (ROC) curves. Analysis of ROC areas should incorporate the correlation structure of repeated measurements taken on the same set of cases and the paucity of measurements per treatment resulting from an effective summarization of cases into a few area measures of diagnostic accuracy. The repeated nature of ROC data has been taken into consideration in the analysis methods previously suggested by Swets and Pickett (1982, Evaluation of Diagnostic Systems: Methods from Signal Detection Theory), Hanley and McNeil (1983, Radiology 148, 839-843), and DeLong, DeLong, and Clarke-Pearson (1988, Biometrics 44, 837-845). DeLong et al.'s procedure is extended to a Wald test for general situations of diagnostic testing. The method of analyzing jackknife pseudovalues by treating them as data is extremely useful when the number of area measures to be tested is quite small. The Wald test based on covariances of multivariate multisample U-statistics is compared with two approaches of analyzing pseudovalues, the univariate mixed-model analysis of variance (ANOVA) for repeated measurements and the three-way factorial ANOVA. Monte Carlo simulations demonstrate that the three tests give good approximation to the nominal size at the 5% levels for large sample sizes, but the paired t-test using ROC areas as data lacks the power of the other three tests and Hanley and McNeil's method is inappropriate for testing diagnostic accuracies. The Wald statistic performs better than the ANOVAs of pseudovalues. Jackknifing schemes of multiple deletion where different structures of normal and diseased distributions are accounted for appear to perform slightly better than simple multiple-deletion schemes but no appreciable power difference is apparent, and deletion of too many cases at a time may sacrifice power. These methods have important applications in diagnostic testing in ROC studies of radiology and of medicine in general.
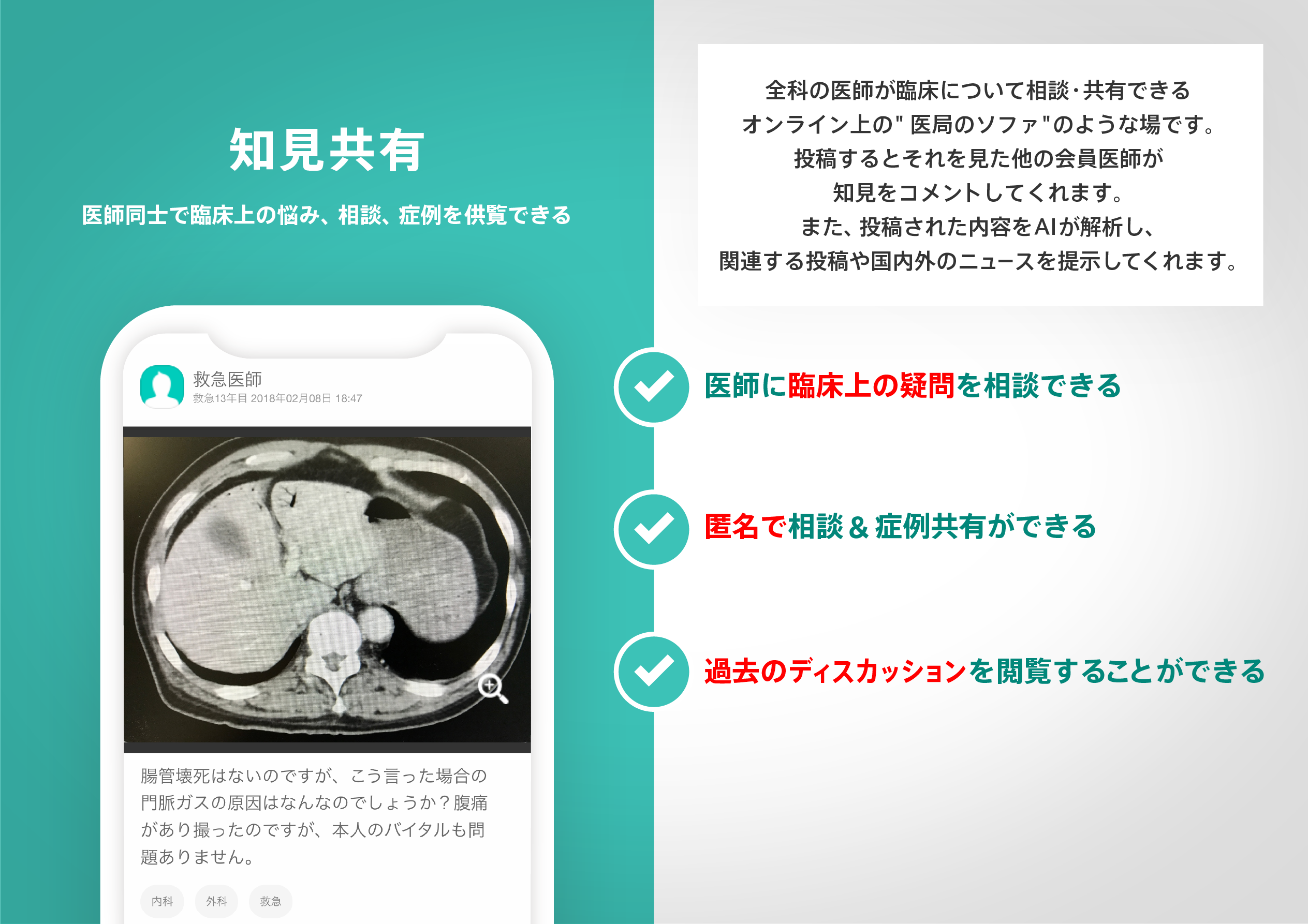
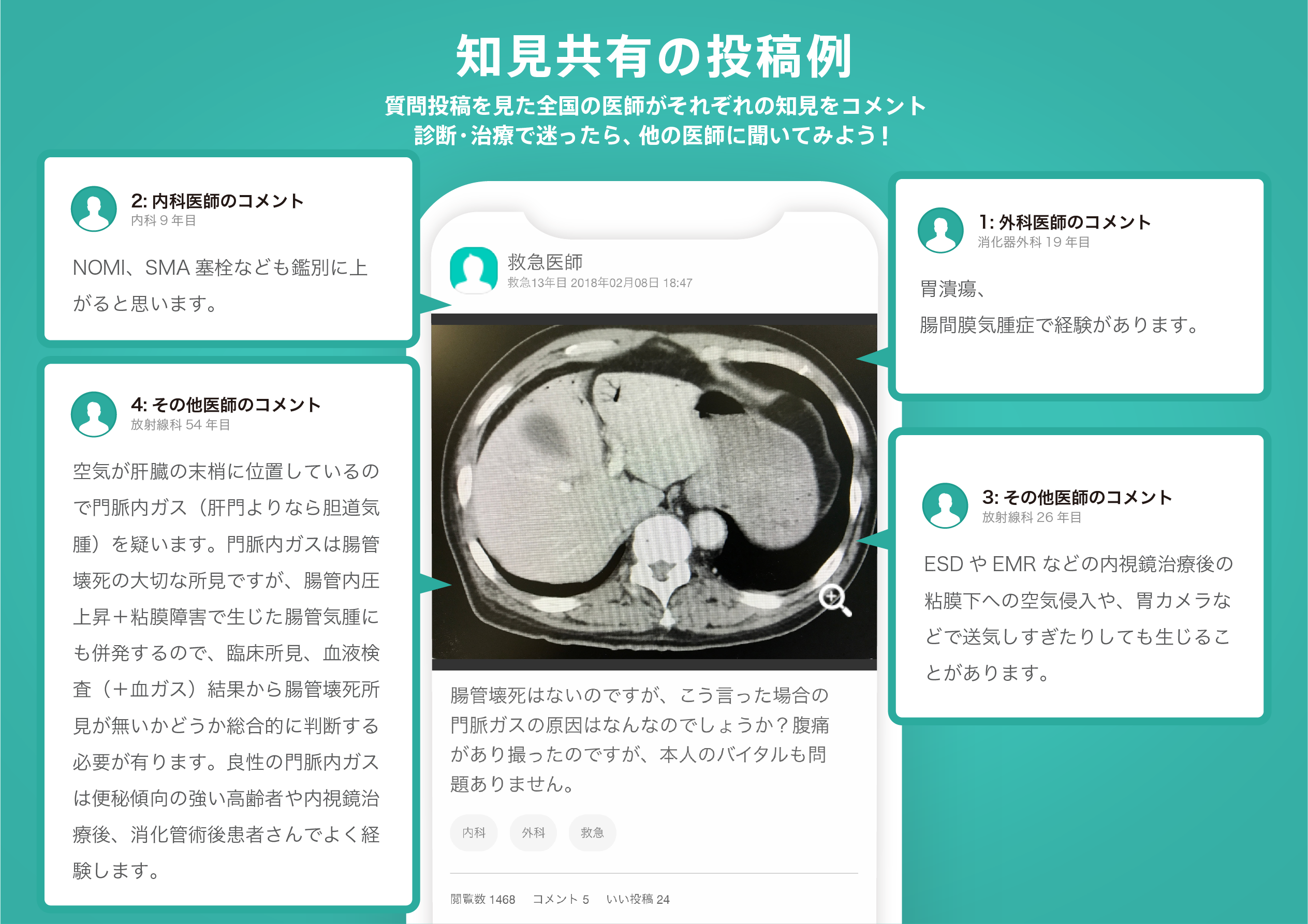
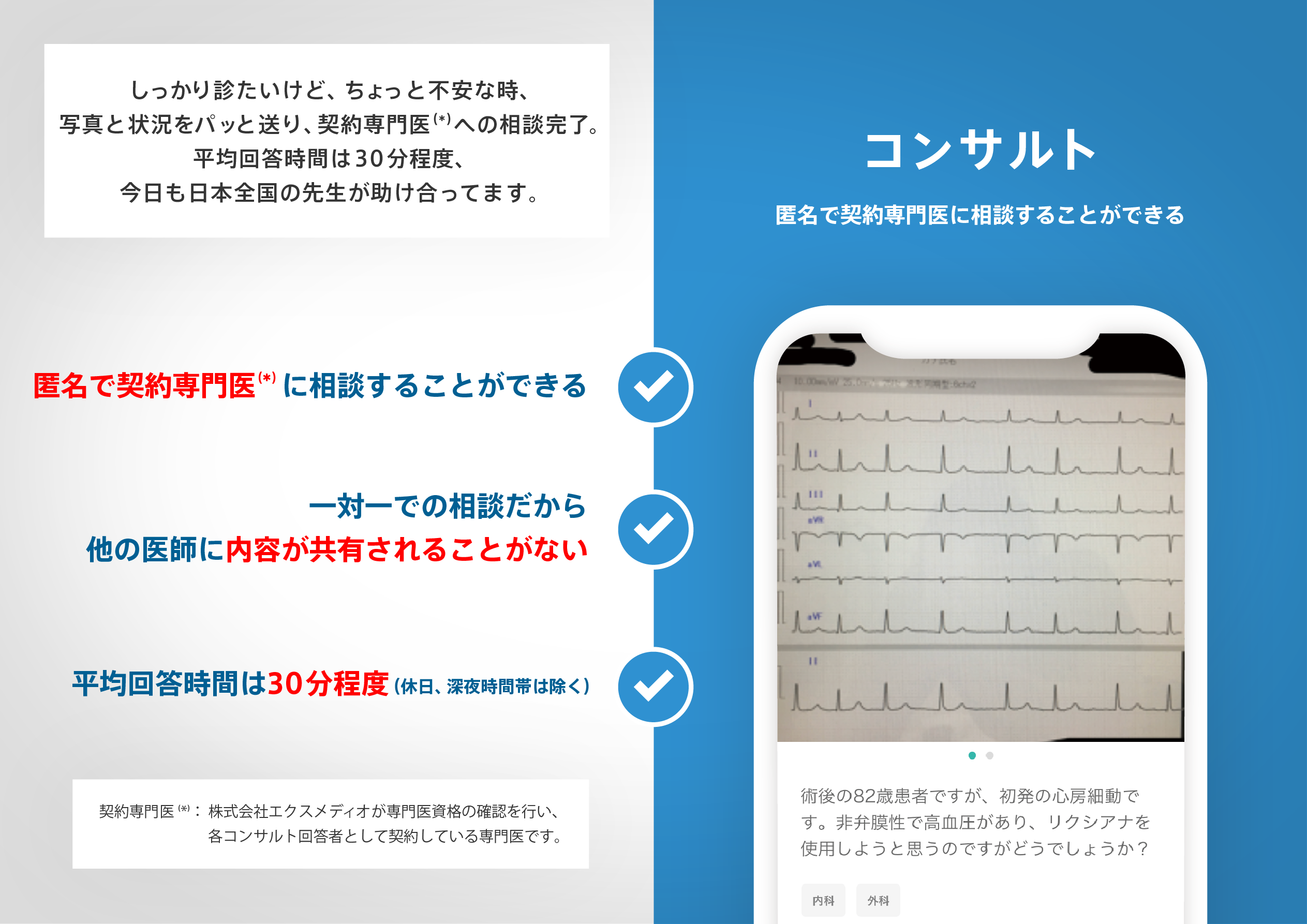
医師のための臨床サポートサービス
ヒポクラ x マイナビのご紹介
無料会員登録していただくと、さらに便利で効率的な検索が可能になります。